Variance- Definition, Formula, Selected Practice Question & Answer

Variance, a term rooted in statistics, is crucial in analyzing data sets. Understanding variance provides insights into the spread and distribution of data points, aiding in decision-making processes. By grasping this concept, individuals can make informed choices based on the variability within their data.

Definition Of Variance In Statistics

Variance in statistics refers to the spread of a set of data points around their mean. It quantifies the variability or dispersion of data values. It is calculated by squared differences between each data point and the mean, then averaged. This process captures how far each data point is from the average value.

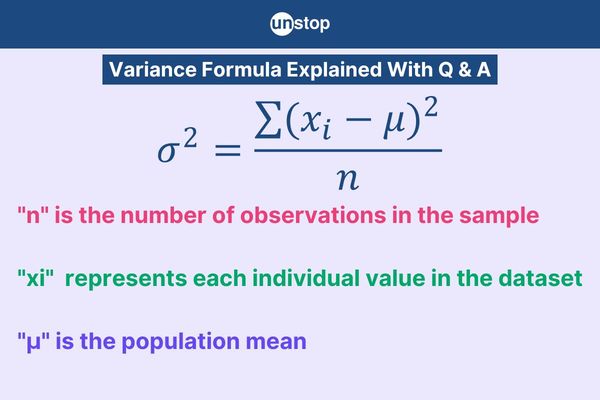
Variance Formula

The formula for variance, denoted as σ² (sigma squared) for a population variance or s² (s squared) for a sample variance, is calculated as follows:

For a population data: 𝜎²=1/𝑁∑^𝑁_𝑖=1(𝑥𝑖−𝜇)²

For a sample data: 𝑠²=1/𝑛−1∑^𝑛_𝑖=1(𝑥_𝑖−𝑥ˉ)²

Where:

N is the number of observations in the population.
n is the number of observations in the sample.
x_irepresents each individual value in the dataset.
μ is the population mean.
xˉ is the sample mean.

The variance measures the dispersion or spread of a set of data points around the mean. The formula calculates the average of the squared differences between each data point and the mean.

The population variance formula is divided by N, while the sample variance formula is divided by n−1 to correct for bias and provide an unbiased estimate of the population variance from the sample.

Calculating Variance

To understand variance better, let's consider a scenario where we have a dataset of numbers 4, 7, 9, 11, and 15. Firstly, find the mean by adding all values and dividing by the total count (4+7+9+11+15 / 5 = 9.2). Next, calculate the differences between each number and the mean: (-5.2, -2.2, -0.2, 1.8, 5.8).

Now that we have these differences, square each one to get rid of negative values: (27.04, 4.84, 0.04, 3.24, 33.64).

To find the variance for this dataset, sum up these squared differences (68.8) and divide by the total count minus one (4): Variance = Sum of Squared Differences / (Total Count - 1).

In this example, the variance is approximately 17.2. A higher variance indicates that data points are more spread out from the mean value of 9.2 in our dataset.

Click here to learn more about various topics related to quantitative aptitude, including variance!

Variance Vs. Standard Deviation

When we look at variance and standard deviation, it's important to know that both show how spread out data is. Variance is found by averaging the squared differences between each data point and the mean. The standard deviation, on the other hand, is simply the square root of the variance.

Standard deviation and variance both reveal how data points differ, but standard deviation is usually preferred because it's easier to grasp. It's presented in the same units as the original data, making comparisons between datasets simpler.

Selected Practice Questions And Answers

Practice is the key to upskilling your knowledge and success! Provided below are some of the best-selected practice questions based on variance:

Question 1: What is the variance of the following dataset: 5, 7, 9, 11, 13, 15, 17?

a) 7

b) 12

c) 10

d) 15

Answer: c) 10

Explanation: To calculate the variance, first find the mean of the dataset. Then, subtract the mean from each data point, square the differences, and find the mean of those squared differences, which is the variance.

Question 2: If the variance of a set is 16 and the mean is 20, what is the standard deviation of the set?

a) 12

b) 8

c) 4

d) 16

Answer: c) 4

Explanation: The standard deviation is the square root of the variance. So, if the variance is 16, the standard deviation is 16=4.

Question 3: Find the variance of the following dataset: 10, 15, 20, 25, 30, 35.

a) 150

b) 107

c) 110

d) 125

Answer: d) 125

Explanation: To calculate the variance, first find the mean of the dataset. Then, subtract the mean from each data point, square the differences, and find the mean of those squared differences, which is the variance.

Question 4: If the variance of a set is 25 and the mean is 12, what is the standard deviation of the set?

a) 5

b) 10

c) 12

d) 15

Answer: a) 5

Explanation: The standard deviation is the square root of the variance. So, if the variance is 25, the standard deviation is 25=5.

Question 5: If the variance of a set is 36 and the mean is -5, what is the standard deviation of the set?

a) 6

b) 24

c) 30

d) 36

Answer: a) 6

Explanation: The standard deviation is the square root of the variance. So, if the variance is 36, the standard deviation is 36=6.

Question 6: What is the variance of the following dataset: 3, 6, 9, 12, 15, 18?

a) 10

b) 25

c) 15

d) 20

Answer: d) 20

Explanation: To calculate the variance, first find the mean of the dataset. Then, subtract the mean from each data point, square the differences, and find the mean of those squared differences, which is the variance.

Question 7: If the variance of a set is 9 and the mean is 5, what is the standard deviation of the set?

a) 2

b) 3

c) 4

d) 5

Answer: b) 3

Explanation: The standard deviation is the square root of the variance. So, if the variance is 9, the standard deviation is 9=3.

Question 8: If the variance of a set is 36 and the mean is 10, what is the standard deviation of the set?

a) 6

b) 12

c) 30

d) 36

Answer: a) 6

Explanation: The standard deviation is the square root of the variance. So, if the variance is 36, the standard deviation is 36=6.

Question 9: What is the variance of the following dataset: 4, 4, 4, 4, 4, 4, 4?

a) 1

b) 3

c) 0

d) 4

Answer: c) 0

Explanation: In a dataset where all values are the same, the variance is 0.

Question 10: If the variance of a set is 100 and the mean is -4, what is the standard deviation of the set?

a) 40

b) 10

c) 20

d) 30

Answer: b) 10

Explanation: The standard deviation is the square root of the variance. So, if the variance is 100, the standard deviation is 100=10.

Conclusion

In conclusion, variance is a key statistical measure that indicates the extent of data dispersion around the mean. By assessing variability within datasets, variance aids in making informed comparisons and drawing meaningful conclusions.

Frequently Asked Questions

1. What is variance in statistics?

Variance in statistics measures the spread or dispersion of a set of data points around their mean. It indicates how much the data points differ from the average.

2. How is variance calculated?

To find the variance, you need to first figure out the mean of all the numbers in your data set. Next, calculate the difference between each data point and the mean. Then, square each of these differences. After that, find the average of all these squared differences. That's your variance!

3. Why is variance important in data analysis?

Variance is crucial in data analysis as it helps to understand the variability within a dataset, identify patterns, make predictions, and assess the reliability of statistical results.

4. What are the advantages of using variance?

Using variance allows for quantifying data variability, comparing different datasets, detecting outliers, and making informed decisions based on the level of dispersion present in the data.

5. Explain the difference between variance and standard deviation.

Variance and standard deviation both show how spread out data is, but the standard deviation is just the square root of variance. People like using standard deviation more because it's in the same units as the original data.

Kaihrii Thomas

Associate Content Writer

Instinctively, I fall for nature, music, humour, reading, writing, listening, travelling, observing, learning, unlearning, friendship, exercise, etc., all these from the cradle to the grave- that's ME! It's my irrefutable belief in the uniqueness of all. I'll vehemently defend your right to be your best while I expect the same from you!