Table of content:
- Basics Of Square Numbers
- Square Chart Upto 30
- Basics Of Cube Numbers
- Cube Chart Upto 30
- Solving Square And Cube Problems
- Identifying Perfect Squares And Cubes
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Squares And Cubes: Roots, Formula, Calculation, Question & Answer
Squares and cubes are mathematical concepts used to find the result of multiplying a number by itself. Understanding the concepts of square and cube is essential in various mathematical calculations.
Basics Of Square Numbers
To begin with, let us understand the basics of square numbers:

Notation
Square numbers are a result of multiplying a number by itself. For example, 3 squared is calculated as 3 x 3, resulting in 9. This process can be represented using the notation n2, where n is the number being squared.
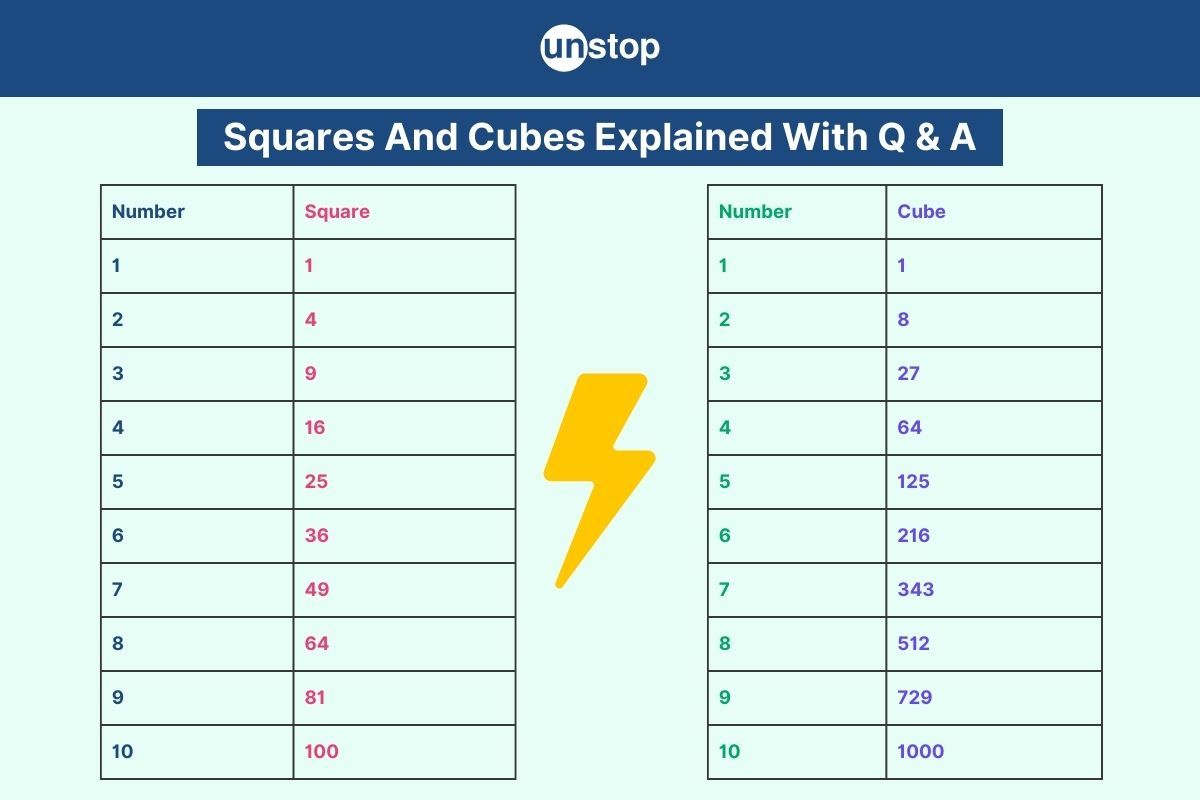
Square Chart Upto 30
It is highly recommended that you memorize the square of numbers up to 20 to save time and speed up your calculation process. Provided below is the chart of squares up to 30:
| Number | Square |
|---|---|
| 12 | 1 |
| 22 | 4 |
| 32 | 9 |
| 42 | 16 |
| 52 | 25 |
| 62 | 36 |
| 72 | 49 |
| 82 | 64 |
| 92 | 81 |
| 102 | 100 |
| 112 | 121 |
| 122 | 144 |
| 132 | 169 |
| 142 | 196 |
| 152 | 225 |
| 162 | 256 |
| 172 | 289 |
| 182 | 324 |
| 192 | 361 |
| 202 | 400 |
| 212 | 441 |
| 222 | 484 |
| 232 | 529 |
| 242 | 576 |
| 252 | 625 |
| 262 | 676 |
| 272 | 729 |
| 282 | 784 |
| 292 | 841 |
| 302 | 900 |
Basics Of Cube Numbers
Let us also study the basics of cube numbers:

Notation
Cube numbers are numbers that result from multiplying a number by itself twice. Cube numbers are represented using the notation n^3, where "n" denotes the base number being cubed. For example, to find the cube of 3 (3√3), you would multiply 3 by 3 by 3, resulting in 27.
Cube Chart Upto 30
Like memorizing square numbers, it is also important to memorize the cube of numbers up to 20 to save time and speed up your calculation process. Provided below is the chart of squares up to 30:
| Number | Cube |
|---|---|
| 13 | 1 |
| 23 | 8 |
| 33 | 27 |
| 43 | 64 |
| 53 | 125 |
| 63 | 216 |
| 73 | 343 |
| 83 | 512 |
| 93 | 729 |
| 103 | 1000 |
| 113 | 1331 |
| 123 | 1728 |
| 133 | 2197 |
| 143 | 2744 |
| 153 | 3375 |
| 163 | 4096 |
| 173 | 4913 |
| 183 | 5832 |
| 193 | 6859 |
| 203 | 8000 |
| 213 | 9261 |
| 223 | 10648 |
| 233 | 12167 |
| 243 | 13824 |
| 253 | 15625 |
| 263 | 17576 |
| 273 | 19683 |
| 283 | 21952 |
| 293 | 24389 |
| 303 | 27000 |
Solving Square And Cube Problems
Let us understand the importance of approach and practice while solving square and cube problems:
Approach
When tackling square and cube problems, start by understanding the basic formulae for calculating them. For squares, multiply a number by itself. When dealing with cubes, multiply a number by itself twice.
Practice
To reinforce your understanding of squares and cubes, practice solving problems that involve both. This not only sharpens your mathematical skills but also helps you grasp the concepts more effectively.
Identifying Perfect Squares And Cubes
Let us study perfect squares and perfect cubes:
Perfect Square
Perfect squares are numbers that result from multiplying a number by itself, such as 1, 4, 9, 16, and so on.
Perfect Cubes
Cubes are numbers obtained by multiplying a number by itself twice, like 1, 8, 27, and beyond.
Methods For Identification
To identify perfect squares and cubes in numerical sequences, one can observe the last digit of the given number. If the last digit is 0, 1, 4, 5, 6, or 9, it might be a perfect square or cube.
Another method involves looking at the sum of digits.
-
For perfect squares: The sum of digits will either be 1, 4, 7, or 9.
-
For perfect cubes: The sum of digits will be divisible by either 3 or 9.
Click here to learn more about various topics and questions related to quantitative aptitude, including those related to squares and cubes.
Selected Practice Questions & Answers
Provided below are some selected practice questions with answers for you to practice and improve your quantitative aptitude skill consistently:
Question 1: If x2 = 81, what is the value of x?
a) 9
b) -9
c) 9 or -9
d) 90
Answer: c) 9 or -9
Explanation: The square root of both sides of the equation x2 = 81.
Either positive or negative square roots will satisfy the equation. The square root of 81=9, so the values of x are x=9 and x=−9.
Question 2: If x3 = -125, what is the value of x?
a) -6
b) 5
c) 7
d) -5
Answer: d) -5
Explanation: Taking the cube root of both sides of the equation x3 =−125. Since −125 is a perfect cube, you can find its cube root easily.
x=3√−125
The cube root of −125 is −5, so the value of x= −5.
Question 3: What is the cube root of -8?
a) -2
b) -4
c) -6
d) -3
Answer: a) -2
Explanation:
The cube root of -8 is -2. When you multiply -2 three times (−2×−2×−2), you get -8. So, the cube root of -8 is -2.
Question 4: What is the value of (x2+y2)2 when x=3 and y=4?
a) 265
b) 365
c) 625
d) 525
Answer: c) 625
Explanation: Given x=3 and y=4
(x2+y2)2=⟨(3)2+(4)2⟩2=(9+16)2=(25)2=625
Therefore, the value of (x2+y2)2 when x=3 and y=4 is 625.
Question 5: If the volume of a cube is 64cm3, what is the length of each side of the cube?
a) 8 cm
b) 6 cm
c) 4 cm
d) 5 cm
Answer: c) 4
Explanation: Given that the volume of the cube is 64cm3, we need to find the cube root of 64:
Cube root of 64=3√64=4
So, the length of each side of the cube is 4 cm.
Question 6: If the volume of a cube is 343 cubic meters, what is the length of one side of the cube?
a) 9 meters
b) 7 meters
c) 8 meters
d) 6 meters
Answer: b) 7 meters
Explanation: Given that the volume of the cube is 343m3, we need to find the cube root of 343:
Cube root of 343=3√343=7
So, the length of each side of the cube is 7 meters.
Question 7: Express 16 as the sum of two perfect squares.
a) 16=52+12
b) 16=32+32
c) 16=42+02
d) 16=62+22
Answer: c) 16=42+02
Question 8: What is the value of (33−23)+(52−42)?
a) 28
b) 25
c) 30
d) 35
Answer: a) 28
Explanation: (33−23)=(27−8)=19
(52−42)=(25−16)=9
Now, we add these results together:
19+9=28
Question 9: What is the value of (62×33)−(42×23)?
a) 874
b) 894
c) 784
d) 844
Answer: d) 844
Explanation: (62×33)=(36×27)=972
(42×23)=(16×8)=128
Now, we subtract the second result from the first:
972−128=844
Question 10: What is the value of (102+53)÷5?
a) 55
b) 45
c) 44
d) 40
Answer: b) 45
Explanation: 102=100
53=125
Now, we add these results together:
100+125=225
Finally, we divide the sum by 5:
225/5=45
Conclusion
You've now mastered the basics of square and cube numbers, learned how to calculate their values, and identified perfect squares and cubes. Keep practising to solidify your understanding and boost your math skills further.
Dive deeper into the world of squares and cubes, explore higher values, and challenge yourself with intricate problem-solving scenarios. Your dedication to mastering these fundamental principles will undoubtedly pave the way for smoother advancement in mathematics.
Frequently Asked Questions (FAQs)
1. What are square numbers and cube numbers?
Square numbers are resulted by multiplying a number by itself, while cube numbers are the result of multiplying a number by itself three times.
2. How can I calculate square values?
To calculate the square value of a number, simply multiply the number by itself. For example, 25 is 5 x 5 = 25.
3. How do I calculate cube values?
Calculating cube values involves multiplying a number by itself twice. For instance, to find the cube of 3, you multiply 3 x 3 x 3 = 27.
4. What is the significance of perfect squares and cubes?
Perfect squares and cubes are important in mathematics as they have exact integer roots. Identifying them aids in simplifying calculations and recognizing patterns in various mathematical problems.
5. Why are squares and cubes of numbers important for a student?
Understanding squares and cubes of numbers is important for students because it helps them develop a strong foundation in mathematics. By learning how to calculate squares and cubes, students improve their mental math skills and problem-solving abilities.
Suggested reads:
- Surds And Indices- Basics, Formulas, Questions And Answers
- Trigonometry Table- Formula, Trick, Selected Questions & Answers
- Train Problems: Concept & Selected Practice Question With Answer
- Decimal To Fraction Conversion Guide (+ Solved Questions)
- Simple Interest- Definition, Formulas, Practice Question & Answer
Instinctively, I fall for nature, music, humour, reading, writing, listening, travelling, observing, learning, unlearning, friendship, exercise, etc., all these from the cradle to the grave- that's ME! It's my irrefutable belief in the uniqueness of all. I'll vehemently defend your right to be your best while I expect the same from you!
Login to continue reading
And access exclusive content, personalized recommendations, and career-boosting opportunities.
Subscribe
to our newsletter



Comments
Add comment