Table of content:
- Step 1: The Groundwork
- Step 2: The Three Sections
- Step 3: Practice Makes Improvement
- The Secret Ingredient
Table of content:
- Understanding Basics Of Number Series
- Types Of Number Series Reasoning Questions
- Addition & Multiplication In Number Series
- Square & Cube-Based Number Series Patterns
- Missing Terms & Arranging Sequences
- Tricks & Tips For Solving Number Series
- Selected Sample Questions With Detailed Solutions
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Terminologies Used in Blood Relation Questions
- What is a Family Tree?
- Types Of Blood Relation Questions
- Verbal Reasoning in Blood Relation
- Tips For Solving Blood Relation Questions
- Common Pitfalls while Solving Questions
- Selected Questions (MCQs) For Practice With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Letter Series In Logical Reasoning
- Types Of Letter Series Patterns
- Tips For Solving Letter Series Reasoning
- Best Practice Question Samples With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basics of Problems On Age-Related Questions
- Formula to Solve Problems on Ages-Related Questions
- Types of Age-Related Questions & Examples
- Tips & Tricks for Solving Ages Problems
- Best MCQs on Problems on ages with solved answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Definition of Calendar
- Understanding Days of the Week
- Understanding the Concept of Leap Year
- Concept of Odd Days in a Century
- Tips For Calendar Reasoning Questions
- Top 10 Calendar Questions & Answers For Practice (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basic Concepts of Clock
- Structure of a Clock
- Angle Equilavalence in Clock
- Tips For Solving Clock Questions
- Selected Clock Questions & Answers (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding The Concept Of Direction Sense
- Tips For Effective Problem-Solving In Direction Sense
- Practical Test Practice Questions And Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Dice Reasoning
- Dice Numbers In Dice Reasoning
- Classification Of Dice
- Constructed Vs Deconstructed Dice
- Tricks & Tips For Solving Dice Problems
- Practice MCQs With Detailed Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Alphanumeric Series Defined
- Alphanumeric Series In Reasoning Tests
- Tips & Strategies For Solving Alphanumeric Series
- Practice Sample Questions With Detailed Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept Of Mirror Image Reasoning Explained
- Important Terms In Mirror Image Reasoning
- Types Of Mirror Images
- Identifying Correct Mirror Image
- Finding Clock's Mirror Image
- Tips To Solve Mirror Images
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept & Overview Of Input-Output
- Input-Output In Competitive Exams
- Types Of Input-Output Problems
- Strategies, Tips & Tricks For Solving Reasoning Questions
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Finding The Odd One Out
- Tricks And Tips
- Strategies For Finding The Odd One Out
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding Key Concepts
- Exploring Different Ranking Types
- Formula And Application Of Order And Ranking
- Tips For Solving Order & Ranking
- Selected Practice Questions And Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Pipes & Cistern Aptitude
- Key Terminologies used in Pipes and Cisterns
- Pipes and Cisterns Formula with Examples
- Pipes and Cisterns Shortcut Tricks
- Tips For Solving Pipes & Cistern Problems
- Selected Questions & Answers For Practice (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Key Concept in Boats and Streams
- Formulas Of Boats & Streams
- Distance & Time Formula
- Tips For Solving Boats & Streams Questions
- Selected Practice Questions With Answers (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept of Mixture and Alligation
- Types Of Alligation Questions
- Formula for Solving Mixture & Alligation
- Tips For Solving Mixture And Alligation
- Selected Questions With Answers For Practice
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Time & Work Concepts
- Time And Work Formulas
- Tips For Solving Time And Work Questions
- Time And Work Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is Harmonic Progression(HP)?
- Formula to find the nth Term in Harmonic Progression
- Formula to find the Sum of the nth Term in HP
- What is Harmonic Mean?
- Harmonic Progression Solved Best MCQs
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is Mensuration in Maths?
- What are 2D figures in Mensuration?
- What are 3D figures in Mensuartion?
- Basic Terminologies In Mensuration
- Basic 2D Formulas in Mensuration
- Basic 3D Formulas in Mensuration
- 2D vs 3D in Mensuration
- Solved Questions With Solutions (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Relationship Between Time, Speed And Distance
- Conversion Units Time, Speed And Distance
- Average & Relative Speed: Two Trains Moving in the same or opposite direction
- Solved MCQs on Time, Speed And Distance
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basics Of Simplification
- BODMAS Rule in Simplification Explained
- Simplification & Approximation Relation
- Key Terms in Simplification
- Examples Of Simplification Techniques
- Simplification Questions With Solved Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Height And Distance Important Terms
- Right Angled Triangle In Trigonometry
- Trigonometric Ratios
- Solved Examples For Better Understanding
- Height And Distance Applications In Trigonometry
- Height And Distance Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Interest Types
- Simple Interest Vs. Compound Interest
- Selected Solved Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basic Concepts Of Profit And Loss
- Determining Selling Price
- Calculating Discounts
- Formulas For Calculating Profit And Loss
- Examples Of Profit And Loss
- Profit & Loss Questions With Detailed Solution
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Angle Of Elevation
- Key Terms Used In Angle Of Elevation
- Angle of Elevation Formula with Example
- Angle of Elevation vs. Angle of Depression
- Angle of Elevation MCQs with Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining HCF And LCM
- Calculation Methods Of HCF And LCM
- HCF By Prime Factorization Method
- LCM By Prime Factorization Method
- Difference Between HCF And LCM
- HCF & LCM Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is fraction and decimal?
- Understanding Decimal Fraction
- Place Value in Decimal Fraction
- Mathematical Operations with Decimal Fraction
- Practice with Solved Examples
- Summary
- Frequently Asked Questions
Table of content:
- All About Decimals
- All About Fractions
- How to Convert a Decimal into Fraction
- Simple vs Recurring Decimals
- Converting Recurring Decimals to Fractions
- Conversion Charts
- Practice Questions (With Solutions)
- Closing Thoughts
- Frequently Asked Questions
Table of content:
- What is Arithmetic Mean?
- Arithmetic Mean Formula- Ungroup Data & Group Data
- Merits of Arithmetic Mean
- Demerits of Arithmetic Mean
- Alternatives to Arithmetic Mean
- What is the Weighted Arithmetic Mean?
- Arithmetic vs. Geometric Mean
- Arithmetic Mean Application in Statistical Analysis
- Arithmetic Mean Practice Questions with Explanation
- Frequently Asked Questions
Table of content:
- What is Geometric Progression?
- Key Properties of Geometric Progression
- General Form Of Geometric Progression
- General Term or the Nth Term of Geometric Progression
- The sum of nth Terms of GP
- Types Of Geometric Progression
- Solved Questions and Answers of GP
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Average in Maths
- Average Formula in Maths
- Differentiating Types of Average
- How to Calculate Average of Negative Numbers?
- Practical Applications of Averages
- Average Questions For Practice
- Frequently Asked Questions
Table of content:
- What is Simple Interest in Maths?
- Simple Interest Formula Explained
- Simple Interest Formula for Years, Months & Days
- Simple Interest Examples & Practice Questions
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Mathematical Ratios
- Understanding Proportions Fundamentals
- Differentiating Ratios from Proportions
- Ratio and Proportion Formulas
- Properties of Ratio and Proportion
- How to Solve Ratio and Proportion Problems
- Ratio and Proportion Problems (With Solutions)
- Summary
- Frequently Asked Questions
Table of content:
- What is Number in Maths?
- Types of Numbers With Example
- Real vs Complex Numbers Explored
- Basic Operations on Numbers
- Practice Questions (With Solutions)
- Frequently Asked Questions
Table of content:
- What is Arithmetic Progression (AP) in Maths?
- Important Terminologies in Arithmetic Progression
- Basic Terms in Arithmetic Progression
- General Form Of Arithmetic Progression Series
- Types Of Arithmetic Progression
- Solved Questions With Explanation (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding Basic Concept
- Importance Of Train Problems In Aptitude
- Tips To Solve Train Problems
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Definition Of Mode
- Calculating Mode For Ungrouped Data
- Calculating Mode For Grouped Data
- Bimodal, Trimodal & Multimodal Distributions
- Comparing Mean, Median & Mode
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Important Concept In Races And Games
- Calculating Time, Speed & Distance
- Importance Of Races And Games Problems
- Sample Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Types Of Partnership
- Formula For Partnership Questions
- Tips To Solve Partnership Aptitude Questions
- Selected Partnership Questions (Practice MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Simple Interest: Definition, Formulas, Solved Questions & Examples
Master the concept of simple interest and familiarize yourself with the formulas and techniques crucial for solving simple interest problems efficiently to prepare for your upcoming competitive exams.
Boost your knowledge and equip yourself with the skills needed to excel in your exams with a solid grasp of simple interest.

What is Simple Interest in Maths?
Simple interest is the rate applied to the principal amount when borrowing or lending money. One of the key characteristics of simple interest is its linear growth. This means that the amount of interest earned or paid remains constant over time since it is based only on the original principal amount.
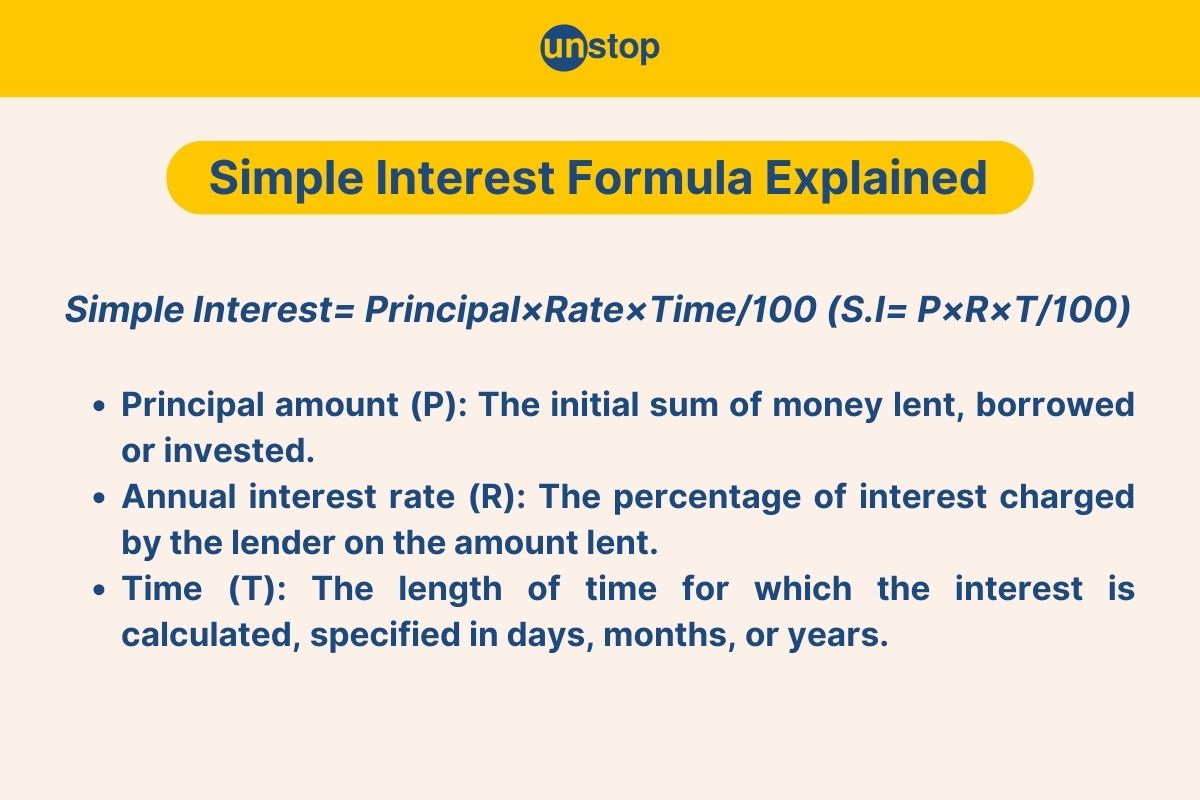
Simple Interest Formula Explained
The formula to calculate simple interest is S.I. = (P × R × T)/100, where P = Principal, R = Rate of Interest in % per annum, and T = Time.
This formula comprises some key elements:
-
Principal amount (P): The initial sum of money lent, borrowed or invested.
-
Annual rate of interest (R): The percentage of interest charged by the lender on the amount lent.
-
Time (T): The length of time for which the interest is calculated, specified in days, months, or years.
- Amount (A): The amount is the total sum of money accumulated after adding the interest to the initial principal calculated by using the formula Amount= Principal+Interest.
Simple Interest Formula for Years, Months & Days
| Time Period | Interest Formula | Amount Formula |
|---|---|---|
| Years | SI=Principal×Rate×Time/100 | Amount=Principal+Interest |
| Months | SI=Principal×Rate/100×Time/12 | Amount=Principal+Interest |
| Days | SI=Principal×Rate/100×Time/365 | Amount=Principal+Interest |
- Principal: The initial amount of money
- Rate: The annual interest rate (as a percentage)
- Time: Duration (in years, months, or days)
Here's a table outlining the key differences between simple and compound interest:
| Aspect | Simple Interest | Compound Interest |
|---|---|---|
| Interest Calculation | Interest is calculated only on the initial principal amount. | Interest is calculated on the principal plus any accumulated interest. |
| Formula | Interest=P×r×t/100 | A=P(1+r/n)nt |
| Interest Accumulation | Interest remains constant over time. | Interest grows exponentially as it compounds. |
| Compounding Frequency | It is not applicable, as interest is not compounded. | Interest can be compounded annually, semi-annually, quarterly, monthly, etc. |
| Complexity | It is simpler to calculate and understand. | It is more complex due to the compounding effect. |
| Growth Over Time | Linear growth, which means interest grows at a constant rate. | Exponential growth means interest grows at an increasing rate over time. |
In summary, simple interest is straightforward and calculated on the principal alone, while compound interest considers the interest on both the principal and the accumulated interest, leading to more significant growth over time.
Click here to enhance and upskill your quantitative aptitude by exploring different topics including simple interest and compound interest.
Simple Interest Examples & Practice Questions
Practice is the key to excelling in simple interest calculation! Provided below are some important selected questions with detailed answers:

Question 1. Calculate the simple interest earned on a principal amount of ₹5000 at an interest rate of 3% per annum for a period of 2 years.
Solution: To solve the question, we can use the formula:
Simple Interest = (Principal amount x Rate x Time)/100
Simple Interest = (5,000x3x2)/100= 30,000/100= 300
Therefore, the simple interest earned on a principal amount of ₹5,000 at an interest rate of 3% per annum for 2 years is ₹300.
Question 2: If ₹500 is invested at an annual interest rate of 4%, how much interest will be earned after 3 years?
Solution: Principal amount (P) = ₹500
Annual interest rate (R)= 4%= 0.04 Time
(T)= 3 years
Simple Interest (I)= P x R x T
= 500 x 0.04 x 3= 60
Therefore, the interest earned after 3 years will be ₹60.
Question 3: If ₹500 is invested at an annual simple interest rate of 8%, calculate the interest earned after 3 years.
Solution: Principal (P)= ₹500
Rate (R)= 8%
Time (T)= 3 years
Simple Interest (SI)= P x R x T / 100
=500x8x3 /100=120
Therefore, the interest earned after 3 years will be ₹120.
Question 4: If ₹5,000 is invested at an annual interest rate of 6%, how much interest will be earned after 3 years using simple interest?
Solution: Simple Interest = Principal (P) x Rate (R) x Time (T)
For the given question, the simple interest = 5,000 x 0.06 x 3= 900
Therefore, the total interest earned after 3 years would be ₹900.
Question 5: John invested ₹5,000 in a savings account for 3 years. In the first year, he earned a simple interest of 5%; in the second year, he earned 7%; and in the third year, he earned 10%. How much money did John have in his account at the end of the third year?
Solution: First-year simple interest = 5,000 x 5% = ₹250
Amount after first year = 5,000 + 250 = ₹5,250
Second-year interest = 5,250 x 7% = ₹367.50
Amount after second year = 5,250 + 367.50 =₹5,617.50
Third-year interest = 5,617.50 x 10% = ₹561.75
Total amount after third year = 5,617.50 + 561.75 = ₹6,179.25
Therefore, John had ₹6,179.25 in his account at the end of the third year.
Question 6: If you invest ₹5,000 in an account that offers a compound interest rate of 5% per year, how much money will you have after 5 years?
Solution: To solve this problem, we can use the formula for compound interest:
A=P×(1+n/r)nt
Now, according to the question:
P = ₹5,000
r=5%=5/100=0.05
n=1 (interest compounded annually)
t=5 years
A=5,000×(1+0.05/1)(1×5)
=5,000×(1.05)5
A=5,000×(1.27628)
A≈6,381.40
So, after 5 years, you would have approximately ₹6,381.40 in the account.
Question 7: If you invest ₹8,000 in an account that offers a compound interest rate of 4% per year, compounded semi-annually, how much money will you have after 3 years?
Solution: According to the question,
-
P = ₹8,000
-
r=4%=0.04 (decimal)
-
n=2 (interest compounded semi-annually)
-
t=3 years
Putting these values into the formula:
A=8,000×(1+0.04/2)(2×3)
A=8,000×(1.02)6
Now, calculate A:
A=8,000×(1.126825)
A≈9,014.60
So, after 3 years, you would have approximately ₹9,014.60 in the account.
Question 8: If you invest ₹10,000 with an annual interest rate of 6%, compounded quarterly, how much money will you have after 7 years?
Solution: According to the question,
-
P = ₹10,000
-
r=6%=0.06 (decimal)
-
n=4 (interest compounded quarterly)
-
t=7 years
Plugging these values into the formula: A=P×(1+r/n)nt
A=10,000×(1+0.06/4)(4×7)
A=10,000×(1+0.06/4)28
A=10,000×(1.015)28
A≈10,000×(1.662792)
A≈16,627.92
So, after 7 years, you would have approximately ₹16,627.92 in the account.
Question 9: Vikas borrowed ₹1,000 from a friend at an annual interest rate of 5%. How much interest will he owe after 2 years?
Solution: Interest=P×r×t
Given:
-
Principal amount ₹1,000
-
Annual interest rate 5% or 0.05
-
Time 2 years
Substituting the given values into the formula:
Interest=1,000×0.05×2=1,000×0.1=100
So, Vikas will owe ₹100 in interest after 2 years.
Question 10: Samaj deposited ₹2000 into a savings account that offers an annual interest rate of 3.5%. How much interest will he earn after 1 year?
Solution: Interest=P×r×t
Given:
- Principal amount ₹2,000
- Annual interest rate 3.5% or 0.035
- Time 1 year
Substituting the given values into the formula:
Interest=2,000×0.035×1=70
So, Samaj will earn ₹70 in interest after 1 year.
Conclusion
For students preparing for competitive exams, mastering the simple interest formula and using simple interest calculators can be highly beneficial. Grasping the differences between simple and compound interest is essential for making informed decisions on financial questions that may appear on exams.
By grasping these concepts, students can approach questions related to interest calculations with confidence and accuracy, ultimately improving their performance in the exam.
Time For A Short Quiz
Frequently Asked Questions (FAQs)
1. What is Simple Interest?
Simple interest is a basic type of interest calculated on the principal amount only. It does not take into account any accrued interest over time.
2. How is Simple Interest calculated?
To calculate simple interest, this formula is utilised: Simple Interest = Principal x Rate x Time. The result gives you the additional amount you will pay or receive based on the principal amount and rate.
3. What are the advantages of using Simple Interest calculators?
Simple interest calculators provide quick and accurate calculations for determining interest amounts. They are user-friendly tools that help individuals make informed financial decisions without complex mathematical computations.
4. List some practical applications of simple interest.
Practical applications of simple interest include calculating interest on loans, determining returns on investments like fixed deposits, and understanding interest charges on credit card balances.
5. How does Simple Interest differ from Compound Interest?
Simple interest is calculated by taking into account only the original amount of money you started with. Compound interest considers both the initial amount and any interest earned so far when determining future interest payments.
6. What is the formula to calculate simple interest separately?
To compute the simple interest separately, subtract the principal from the total accrued amount using the formula (Interest = Total accrued amount—Principal amount).
Suggested reads:
- Decimal To Fraction Conversion Guide (+ Solved Questions)
- Arithmetic Mean: Get Definition, Formula, Solved Practice Questions
- Decimal Fraction: Find Types, Operations & Practice Questions
- Profit And Loss- Basic Concepts, Formulas, Questions & Answers
- Time, Speed And Distance- Formula With Aptitude Question & Answer
Instinctively, I fall for nature, music, humour, reading, writing, listening, travelling, observing, learning, unlearning, friendship, exercise, etc., all these from the cradle to the grave- that's ME! It's my irrefutable belief in the uniqueness of all. I'll vehemently defend your right to be your best while I expect the same from you!
Comments
Add commentLogin to continue reading
And access exclusive content, personalized recommendations, and career-boosting opportunities.
Subscribe
to our newsletter










ANKIT KUMAR 1 week ago
Khushal Bansal 3 weeks ago
YASH KAPOOR 1 month ago
avneesh kaur 1 month ago
Pardha Venkata Sai Patnam 1 month ago
Sanchita roy 1 month ago