Home Entrance Exam Prep Calendar Questions: Selected Aptitude Questions & Answers (MCQs)

Calendar Questions: Selected Aptitude Questions & Answers (MCQs)

Looking for quantitative calendar questions? Get the best-selected questions and answers in MCQ format with detailed explanations.

Kaihrii Thomas

0 min read

Calendar Questions: Selected Aptitude Questions & Answers (MCQs)

0 min read

Table of content:

Definition of Calendar
Understanding Days of the Week
Understanding the Concept of Leap Year
Concept of Odd Days in a Century
Tips For Calendar Reasoning Questions
Top 10 Calendar Questions & Answers For Practice (MCQs)
Conclusion
Frequently Asked Questions (FAQs)

Studying calendars helps us learn about leap years, odd days, and how to solve calendar questions in logical reasoning. Get ready to enhance your knowledge and find answers to your burning calendar questions right here.

Definition of Calendar

A calendar is a visual representation that displays the days, weeks, and months of a specific year, often providing details about different seasons as well.

Grasping the Basic Structure of a Calendar

Ordinary Year: An ordinary year is defined as a year that contains 365 days. Examples of such years include 1879, 2009, and 2019.
Leap Year: A leap year is defined as a year that consists of 366 days instead of the usual 365. Examples of leap years include 2012, 2016, and 2020.
When you divide 365 by 7, the result is 52 with a remainder of 1, meaning that a typical year consists of 52 weeks plus one additional day. This extra day is commonly known as an “odd day” in discussions about calendars.
A leap year consists of 366 days. When you divide 366 by 7, you get a quotient of 52 and a remainder of 2. This means that in a leap year, there are 52 full weeks along with 2 additional days. These extra days are commonly known as “odd days.”

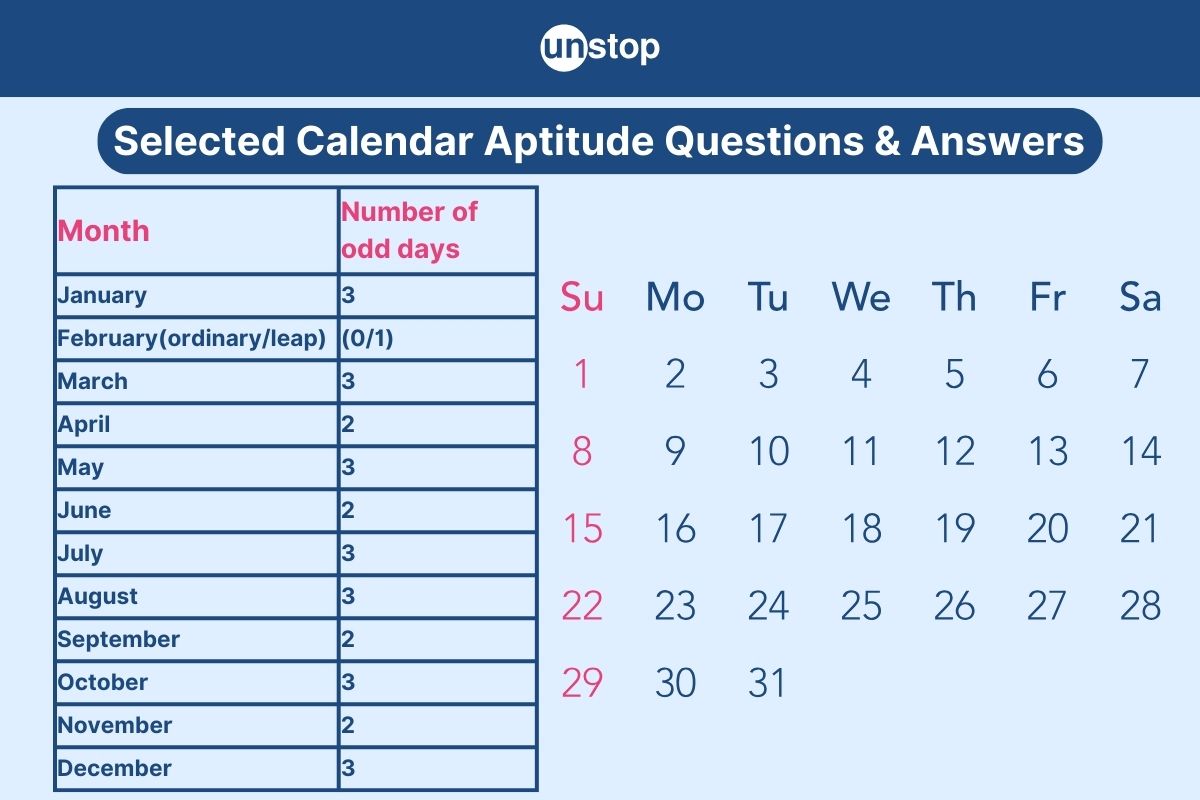
The table below shows the count of odd days for each month in a calendar year:

Month Number of odd days

January 3

February(ordinary/leap) (0/1)

March 3

April 2

May 3

June 2

July 3

August 3

September 2

October 3

November 2

December 3

Understanding Days of the Week

The week starts on Monday, which is why Saturday and Sunday are known as the weekend. This understanding can help simplify calculations and save time during exams.

The table below illustrates the coding system for the days of the week:

Code of the day	Day
0	Sunday
1	Monday
2	Tuesday
3	Wednesday
4	Thursday
5	Friday
6	Saturday

This table assists in interpreting the responses gathered after completing the problem-solving steps related to calendar subjects. For example, if the final result of your calculations is 10, you would divide that number by 7, since there are 7 days in a week.

The remainder from this division, which is 3, helps you identify the correct answer from the options provided. In this case, a remainder of 3 corresponds to Wednesday as the correct choice.

Understanding the Concept of Leap Year

Leap year infographic

A leap year is a year that has 366 days instead of the usual 365, with an extra day added to February (February 29). Leap years occur every 4 years to help synchronize the calendar year with the Earth's orbit around the Sun, which takes about 365.25 days.

Rules to determine a leap year:

A year is a leap year if it is divisible by 4.
However, if the year is divisible by 100, it is not a leap year unless:
The year is also divisible by 400. In that case, it is a leap year.

Examples:

2020 was a leap year (divisible by 4).
1900 was not a leap year (divisible by 100 but not 400).
2000 was a leap year (divisible by 400).

Concept of Odd Days in a Century

The concept of odd days in a century helps students answer the question of calendars as quickly as possible. Let us study the question below in order to understand the concept better:

What was the day of the week on December 31st in the year 100 A.D.?

Solution: Let's examine the initial century, specifically from Year 1 A.D. to Year 100 A.D.

When we divide 100 by 4, we find that within the first century, there are 76 ordinary years and 24 leap years. Although dividing 100 by 4 gives us 25, the year 100 is not a leap year because it is not divisible by 4. Therefore, we count only 24 leap years instead of 25.

100 years = 76 ordinary years + 24 leap years

An ordinary year contributes 1 odd day, while a leap year adds 2 odd days. Therefore, if we consider 76 ordinary years, they will accumulate a total of 76 odd days. In the case of 24 leap years, they will generate 24 multiplied by 2, resulting in 48 odd days. When we combine these two figures, we find that there are 124 odd days altogether.

100 years = (76 x 1 + 24 x 2) odd days = 124 odd days.

When you divide the total of 124 odd days by 7, you find that it equals 17 weeks with a remainder of 5. This means that in those 124 days, there are 17 complete weeks and an additional 5 odd days left over.

100 years = 17 weeks + 5 odd days. Therefore, the number of odd days in 100 years = 5.

According to the table provided above, the number 5 corresponds to Friday when decoding numbers into days of the week.

In the same way, you can determine the final day of another century years by applying this reasoning. Over a span of 100 years, there are typically 5 odd days. Therefore, for 200 years, we would expect to see 10 odd days. Since 10 exceeds 7, if we divide 10 by 7, the remainder is 3. This indicates that after 200 years, there are 3 odd days remaining. Consequently, this means that December 31, 2000, fell on a Wednesday.

Number of odd days in 200 years = (5 x 2) = 10 = (7+3) = 3 odd days.

In a century, there are 5 odd days, which means that over 200 years, there are a total of 10 odd days. Following this pattern, it stands to reason that in 300 years, there would be 15 odd days. When you divide 15 by 7, the remainder is 1, which tells us that the last day of the year 300 falls on a Monday. Therefore, the final day of that year was a Monday.

Number of odd days in 300 years = (5 x 3) = 15 = (14+1) = 1 odd day.

Over a span of 400 years, there should be a total of 20 odd days because the last year, being a leap year is divisible by 4. Therefore, this specific leap year adds one more odd day, resulting in 21 odd days altogether. When you divide 21 by 7, the remainder is zero. This means that after 400 years, there are no odd days left, indicating that the starting day was a Sunday.

The table below provides a logical approach to calculating the number of odd days and identifying the corresponding days of the week in 100 years:

Century	Number of odd days	Day of the week
100	5	Friday
200	3	Wednesday
300	1	Monday
400	0	Sunday
500 = (100+400)	(5+0) = 5	Friday
600 =(200+400)	(3+0) =3	Wednesday
700 = (300+400)	(1+0) = 1	Monday
800 = (400+400)	(0+0) = 0	Sunday
900 = ( 400 + 500)	(0 + 5) = 5	Friday
1000 = (500 +500)	(5+5)= (7+3) = 3	Wednesday

Studying the table closely, you will observe the following points:

1. Every four centuries, the sequence of days repeats itself, including the specific days on which events conclude. The recurring order of these days is always Friday, Wednesday, Monday, and Sunday.

2. A century will conclude on one of the following days: Friday, Wednesday, Monday, or Sunday. These days correspond to the values of 5, 3, 1, and 0 when decoded.

3. A century cannot conclude on a Tuesday, Thursday, or Saturday, as the numerical representations for these days are 2, 4, and 6, respectively.

Tips For Calendar Reasoning Questions

Let us look at some of the tips for solving calendar reasoning questions:

Understanding Concepts

To tackle calendar questions effectively, grasp the fundamentals like leap years and odd days.

Practice & Techniques

Regular practice with various types of calendar reasoning questions is essential for honing your skills. Employ logical reasoning techniques to break down the information systematically and reach accurate conclusions.

To improve your logical reasoning skills specifically for calendar questions, there is a dedicated platform that offers practice materials just for you. Click here to access the platform right away!

Conclusion

The exploration of calendar questions has shed light on the intricate world of calendars, leap years, odd days, and effective strategies for solving related reasoning questions. By grasping these concepts and practicing sample questions, individuals can enhance their problem-solving skills and excel in this domain.

Engage in further practice, challenge yourself with diverse scenarios, and continue exploring the fascinating realm of calendars to sharpen your reasoning abilities.

Frequently Asked Questions (FAQs)

1. What are the basics of understanding calendars?

Understanding calendar basics encompasses learning about days, months, years, and their relationships. It involves comprehending concepts like weekdays, leap years, odd days, and different calendars used worldwide.

2. How do you evaluate leap years in a calendar?

Grasping the evaluation of leap years involves knowing that a leap year has 366 days instead of 365. It occurs every four years except for centuries, which are not divisible by 400. This adjustment compensates for the extra quarter day in Earth's orbit around the sun.

3. What are odd days in calendars, and how are they counted?

Odd days in calendars refer to the leftover days when a certain number of weeks or complete months don't fit into a given period. Counting odd days involves dividing the total number of days by seven (days in a week) and considering the remainder as odd days.

4. How can one effectively solve calendar reasoning questions?

Effective strategies for solving calendar reasoning questions include practicing regularly to improve mental calculations related to dates and weekdays. Understanding basic calendar concepts such as leap years and odd days is crucial for mastering reasoning questions efficiently.

5. Why is it important to explore various types of calendars used today?

Discovering various types of calendars used today helps broaden knowledge of cultural diversity and historical significance related to timekeeping systems globally. Exploring different calendars enhances understanding of traditions, religious practices, astronomical observations, and societal influences on time reckoning.