Seating Arrangement: Best Aptitude Questions With Tips and Tricks

14 mins read

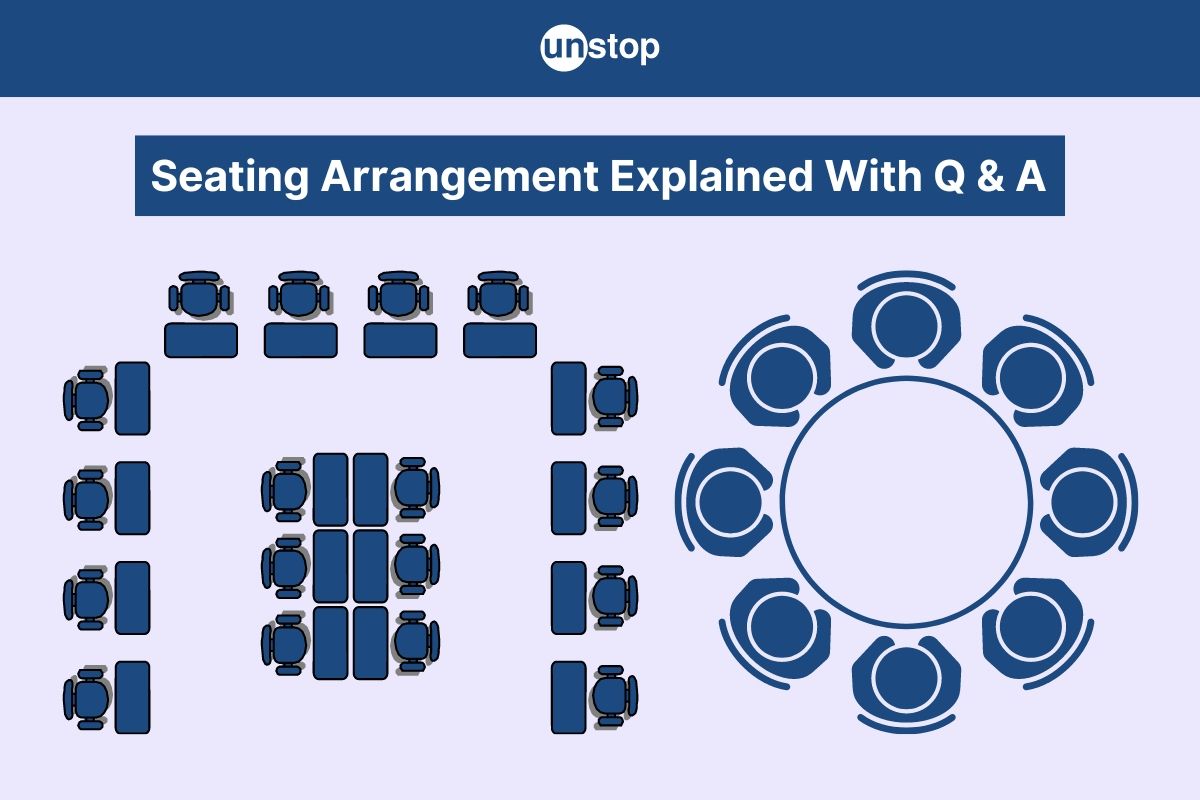
Seating arrangement plays a crucial role in reasoning tests, where candidates are often presented with conditions or rules that dictate how individuals or items should be arranged in a particular order.

By studying the information and correctly applying logic, you can determine the right seating order that meets all the rules. Practising different seating problems can boost test scores and thinking skills.

Key Concepts in Seating Arrangement

Let us understand the key concepts for seating arrangement in reasoning:

Logical Reasoning Skills

Mastering logical reasoning through practice and utilizing reasoning resources can enhance one's ability to tackle seating arrangement questions efficiently. This skill is essential for various competitive exams, job interviews, and daily problem-solving scenarios. 

Essential Concepts & Principles

To excel in seating arrangement reasoning, grasping fundamental concepts such as direction sense, order ranking, blood relations, etc., is vital. Applying principles like creating diagrams or grids while solving puzzles enhances clarity and organization when arranging persons based on conditions.

Types of Seating Arrangement Questions

To begin with, let us understand the different types of seating arrangement questions:

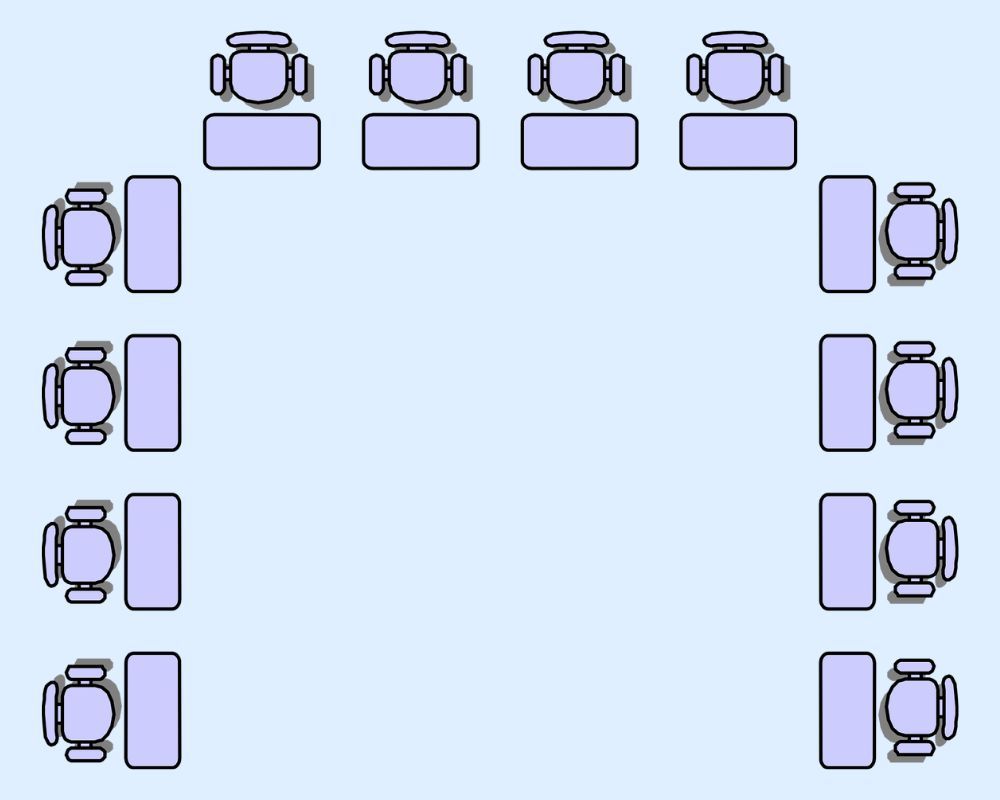
Linear seating arrangements

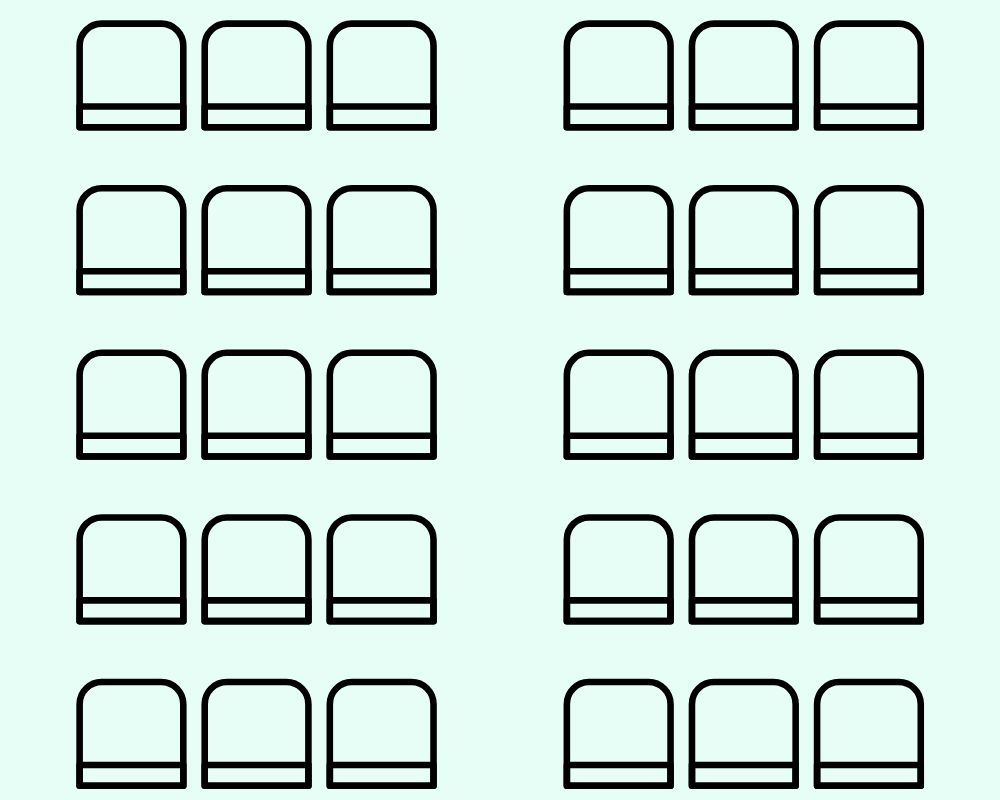
Linear seating arrangements involve arranging individuals in a straight line. Properties of linear seating include facing each other or facing the same direction. Linear setups can be tricky when choosing who sits at the ends or next to specific individuals.

Example

In a logical and aptitude test, a suitable example of a linear seating arrangement could be as follows:

A, B, C, D, and E - are friends sitting in a row looking towards the north. They like different colors - red, blue, green, yellow, and orange. The following information is known:

1. A is sitting at one of the ends.

2. B is sitting next to E and does not like red.

3. C is sitting at the extreme left end and likes green.

4. D is sitting between A and C.

5. The person who likes blue is sitting next to the person who likes yellow.

Linear Seating Arrangements characteristics:

• Easy visualization

• Clear ordering of seats

• Simple directions for positioning

Circular seating arrangements

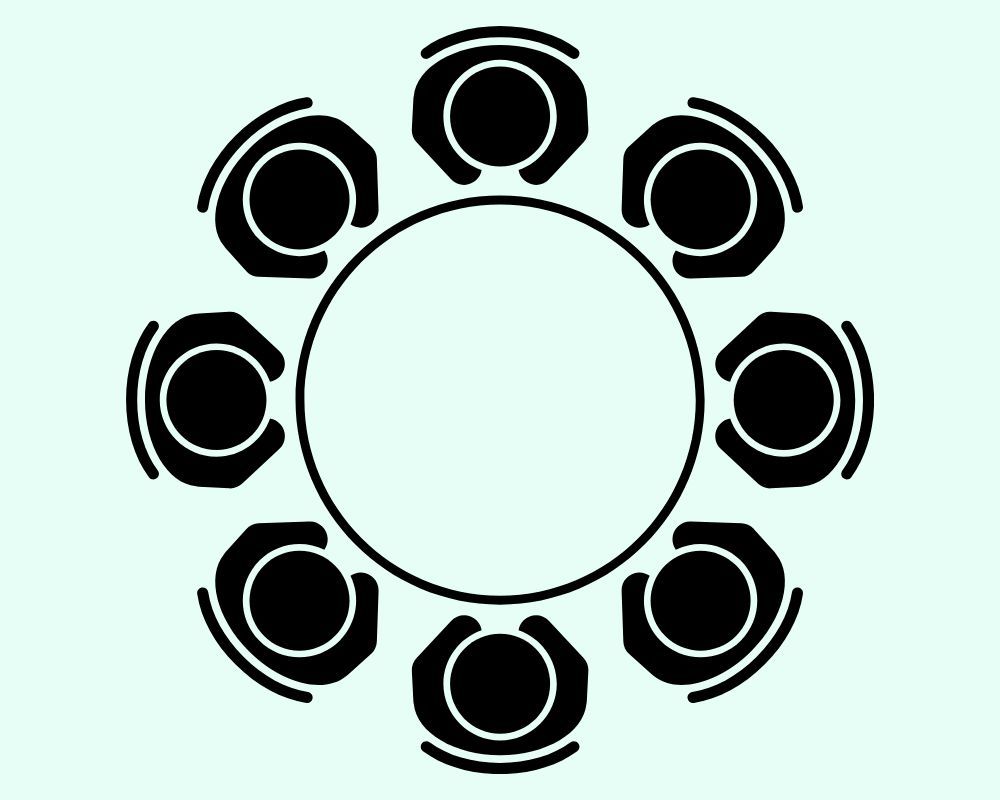
Circular seating involves placing people around a table or in a circular formation. The arrangement revolves around fixed points like centre-facing chairs or fixed positions on the circle's circumference.

Understanding properties such as clockwise or counterclockwise direction is key. In circular setups, identifying immediate neighbours can be challenging due to continuous rotation possibilities.

Example

For a logical test involving circular seating arrangements, consider a scenario where six friends - A, B, C, D, E, and F - are seated around a circular table facing the centre. The following conditions apply:

1. A is sitting two seats away from B.

2. C is seated next to D.

3. E is not sitting next to F.

Circular seating characteristics:

• Equidistant placement from the center

• Directional considerations

• Fixed central point reference

Polygonal seating arrangements

Polygonal arrangements are complex configurations where individuals are seated along multiple sides, forming shapes like squares, rectangles, or hexagons. These setups require attention to angles and distances between each person based on their position within the shape.

Example

In a logical and aptitude test, a suitable example of polygonal seating arrangements could be as follows:

Six friends - Alex, Ben, Chris, David, Emily, and Fiona - are seated around a hexagonal table. The following conditions apply:

1. Alex is seated opposite to Ben.

2. Chris is seated next to Fiona.

3. David is not seated next to Emily.

Polygonal seating characteristics:

• Different angle considerations

• Varying distance calculations

• Unique corner interactions

Navigating polygonal arrangements demands understanding geometric relationships among participants positioned across different edges.

Concentric Seating Arrangement

Concentric seating arrangements place individuals in a circular formation, with each subsequent circle surrounding the centre. This setup creates a dynamic where participants are positioned based on their relation to the core point.

In this configuration, those closer to the middle have varying degrees of influence or importance compared to those on the outer edges.

Example

Consider a situation with three concentric circles labelled A, B, and C in a concentric seating arrangement scenario. Each circle represents a different group of people. Circle A contains 10 people, Circle B comprises 15 people, and Circle C includes 20. How many handshakes will occur if each person shakes hands with everyone in the other two circles?

Tips on How to Solve Seating Arrangement Questions

Let us look at some of the important rules to be followed while solving seating arrangement questions:

Understand the Question

Seating arrangement questions involve arranging people or objects around a table. These questions may include conditions like "A is sitting next to B" or "C is opposite D." To solve them, carefully read and visualize the given information. Key clues can be phrases like "next to," "opposite of," or "between." 

Use Elimination Techniques

Eliminate impossible options based on given conditions. For instance, if P must sit beside Q and R cannot sit next to S, use this information to eliminate choices where P is not near Q or R is beside S. This method helps narrow down possibilities quickly.

Keep Track of Information

Maintain a clear record of all details provided in the question while solving seating arrangement problems. This prevents confusion and ensures no conditions are overlooked during analysis.

Common Mistakes to Avoid in Seating Arrangement Problems

Let us study some of the common mistakes that you need to avoid while solving seating arrangement questions:

Overlooking Important Details

When tackling seating arrangement problems, a common mistake is overlooking vital details. Missing key information can lead to incorrect conclusions. Another aspect often overlooked is the total number of people involved.

Not accounting for the exact number of individuals in the arrangement may cause confusion and errors when arranging the seats.

Falling into Traps

Individuals attempting seating arrangement questions might fall into traps set within the problem statement. These traps are designed to mislead and confuse, leading to incorrect solutions if not identified promptly.

Making Careless Mistakes

Carelessness during the solving process can also lead to errors in seating arrangements. Failing to double-check placements or missing out on subtle clues provided in the question can result in wrong answers.

10 Sample Practice Questions with Answers

Let us study some of the sample questions of seating arrangement with solutions of seating arrangement:

Question 1: The letters A, P, R, X, S, and Z are arranged in rows. S and Z are positioned in the middle. A and P are at the ends. R is seated to the left of A. Who is seated to the right of P?

Solution

• Let's start with R is seating to the left of A and since A sitting at the corner hence it will be right most corner then P will be at left most corner
• Since S & Z are in middle hence X is only left who will be neighbour of P.

Question 2: What is the current location of A in a linear arrangement facing north?

1. F is immediately to the right of E.
2. E is in the fourth position to the right of G.
3. C is sitting next to both B and D.
4. The person to the third position on the left of D is at one of the ends.

Solution

Begin with placing G & E, which will make 3 cases, then, place D using statement 4. Next, using statement 3 place C & B which will eliminate one case. Next, by using statement 1 place F which will eliminate another case and leaving only one position for A which is right corner.

Question 3: 5 friends - A, B, C, D, and E are sitting around a circular table facing the centre. A is sitting to the immediate left of B, D is sitting opposite to E, and C is sitting to the immediate right of E. Who is sitting between A and C?

Solution

Begin with placing E & D opposite of each other and then placing C to immediate right of E. Now there is only one way to place A & E.

The seating arrangement is as follows: A - B - E - C - D
Therefore, D is sitting between A and C.

Question 4: Five friends, P, Q, R, S, and T, sit around a circular table facing the centre. P is sitting second to the right of Q. R is sitting second to the left of S. T is seated opposite to R. Who is sitting between P and T?

Solution

First, we need to determine the positions of each friend around the table:

• R is second to the left of S.
• T is sitting opposite R.
• Now, P is second to the right of Q 

From the arrangement, we can see that S sits between P and T on the circular table.

Question 5: Who is situated next to B?

1. F is immediately to the right of E.
2. E is in the fourth position to the right of G.
3. C is sitting next to both B and D.
4. The person to the third position on the left of D is at one of the ends.

Solution     

Begin with placing G & E, which will make 3 cases. Then, place D using statement 4. Next, using statement 3 place C & B which will eliminate one case. Now by using statement 1 place F which will eliminate another case and leaving only one position for A which is right corner.

Hence G & C will be sitting next to B.

Question 6: To the left of C, who are standing?

1. F is immediately to the right of E.
2. E is in the fourth position to the right of G.
3. C is sitting next to both B and D.
4. The person to the third position on the left of D is at one of the ends.

Solution

Begin with placing G & E, which will make 3 cases. Then, place D using statement 4. Next, using statement 3 place C & B which will eliminate one case. Then, using statement 1 place F which will eliminate another case and leaving only one position for A which is right corner.

Hence B & G are standing to the next of C.

Question 7: A, B, C, D, E, F, G, and H are 8 friends seated in a circular arrangement facing the centre.

1. A is sitting third to the left of B.
2. G is sitting second to the right of A.
3. F is sitting second to the right of H.
4. D is sitting second to the left of E.
5. C is sitting second to the left of D.

Who is sitting between C and D?

Solution

Begin with placing A, B & G as they are straight forward statements. So, there will be total 4 cases when you will place H & F along with D & E. Then, by using last statement about C & D, only 1 case will remain which will give you that F is sitting between C and D in the circular seating arrangement.

Question 8: In a tech exhibition, seven mobiles of seven different companies, viz. Motorola, Sony, HTC,
Oppo, Micromax, Lenovo, and Apple were displayed in a row, facing north such that:

1. Motorola was to immediate right of Apple.
2. Apple was fourth to the right of HTC.
3. Oppo was between Sony and Lenovo
4. HTC, which was third to the left of Sony, was at one of the ends.

Which mobiles are on the immediate either sides of Motorola?

Solution

Start with statement 4 placing HTC & Sony in the left corner and then using statement 2 place Apple. Next, using statement 1 place Motorola and then by using statement 3 place Oppo and Lenovo. This will make Micromax in the right most corner.

Hence Apple & Micromax will be immediate sides of Motorola.

Question 9: In a tech exhibition, seven mobiles of seven different companies, viz. Motorola, Sony, HTC,
Oppo, Micromax, Lenovo, and Apple were displayed in a row, facing north such that:

1. Motorola was immediate right of Apple.
2. Apple was fourth to the right of HTC.
3. Oppo was between Sony and Lenovo
4. HTC, which was third to the left of Sony, was at one of the ends.
   

Which of the mobiles are to the right of Sony?

Solution

Start with statement 4 placing HTC & Sony in the left corner and then, using statement 2 place Apple. Next, using statement 1 place Motorola and then using statement 3 place Oppo and Lenovo. This will leave Micromax to be placed in the right most corner.

Hence Apple, Motorola & Micromax will be in the right sides of Sony.

Question 10: A, B, C, D, and E are friends who are standing in a row looking towards the north.

1. A is sitting at one of the ends.
2. B is sitting to the right of C.
3. D is sitting between B and E.
4. C is not sitting at any of the ends.

Based on the given information, determine the seating arrangement of the five friends.

Solution

From point 1, we know that A is sitting at one of the ends. Therefore, A can only be at the left end or the right end. Let's consider both possibilities:

Case 1: A is at the left end A _ _ _ _

From point 2, B is sitting to the right of C. Since C cannot be at either end, C must be in the middle. Therefore, the arrangement becomes:
A C B _ _

From point 3, D is sitting between B and E. Since E is not mentioned in any other condition, we can place E at the right end:
A C B D E

Therefore, the final seating arrangement is A, C, B, D, E.

Case 2: A is at the right end _ _ _ _ A

Using the same logic as above, we can determine that the final seating arrangement in this case is: C B D E A

Therefore, there are two possible seating arrangements:

1. A C B D E
2. C B D E A

But from statement 4 we know that C cannot be at either ends. Hence, only 1st arrangement will be left and A C B D E will be the arrangement.

If you are looking for a course to enhance your logical and aptitude skills to crack placement interviews or any competitive examination, click here to access the course right away.

Conclusion

In conclusion, mastering the intricacies of seating arrangement problems demands a keen eye for detail and a methodical approach. Understanding the types of questions, key concepts, and various arrangements is essential for success.

Embracing a systematic mindset and honing these techniques will undoubtedly sharpen one's reasoning abilities in seating arrangement problems. Continuous practice, coupled with a focus on accuracy and efficiency, is key to conquering these challenges successfully. The provided sample questions offer valuable practice opportunities to consolidate this knowledge.

Quiz Time- Let's Test Your Understanding

Frequently Asked Questions (FAQs)

1. What are the different types of seating arrangement questions covered in this article?

The blog post covers various types, such as linear, circular, polygonal, and concentric seating arrangements. Each type presents unique challenges and requires specific strategies for solving them effectively.

2. How can one improve their reasoning skills for seating arrangement problems?

Understanding key concepts like spatial visualization, logical reasoning, and pattern recognition is crucial. Practice regularly with different scenarios to enhance your problem-solving abilities in seating arrangement questions.

3. Are there specific rules to follow when tackling seating arrangement problems?

Yes, certain rules apply, such as considering clues given in the question, creating accurate diagrams or models of the seating arrangements, eliminating impossibilities systematically, and using logic to deduce correct placements.

4. What are some common mistakes that test-takers make while solving seating arrangement problems?

Common mistakes include misinterpreting clues provided in the question, overlooking important details leading to incorrect assumptions or placements, and neglecting to update information based on new deductions made during the process.

5. How can one effectively approach sample practice questions provided in this blog post?

Analyze each question carefully by identifying all given conditions and constraints before attempting a solution. Practice drawing accurate diagrams representing different scenarios based on the information provided for better comprehension and problem-solving accuracy.

Suggested reads:

• CAT 2024: Tips To Score Well In Logical Reasoning And Data Interpretation (LRDI) Section
• What Is Bitsat Exam?: Find Application Process, Exam Pattern & More
• 50+ Power BI Interview Questions (With Answers) For 2024 Job Interviews
• 70+ Agile Interview Questions And Answers For 2024 Job Interviews
• How To Become An Astronaut In India | Qualification & Requirement