Table of content:
- Step 1: The Groundwork
- Step 2: The Three Sections
- Step 3: Practice Makes Improvement
- The Secret Ingredient
Table of content:
- Understanding Basics Of Number Series
- Types Of Number Series Reasoning Questions
- Addition & Multiplication In Number Series
- Square & Cube-Based Number Series Patterns
- Missing Terms & Arranging Sequences
- Tricks & Tips For Solving Number Series
- Selected Sample Questions With Detailed Solutions
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Terminologies Used in Blood Relation Questions
- What is a Family Tree?
- Types Of Blood Relation Questions
- Verbal Reasoning in Blood Relation
- Tips For Solving Blood Relation Questions
- Common Pitfalls while Solving Questions
- Selected Questions (MCQs) For Practice With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Letter Series In Logical Reasoning
- Types Of Letter Series Patterns
- Tips For Solving Letter Series Reasoning
- Best Practice Question Samples With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basics of Problems On Age-Related Questions
- Formula to Solve Problems on Ages-Related Questions
- Types of Age-Related Questions & Examples
- Tips & Tricks for Solving Ages Problems
- Best MCQs on Problems on ages with solved answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Definition of Calendar
- Understanding Days of the Week
- Understanding the Concept of Leap Year
- Concept of Odd Days in a Century
- Tips For Calendar Reasoning Questions
- Top 10 Calendar Questions & Answers For Practice (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basic Concepts of Clock
- Structure of a Clock
- Angle Equilavalence in Clock
- Tips For Solving Clock Questions
- Selected Clock Questions & Answers (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding The Concept Of Direction Sense
- Tips For Effective Problem-Solving In Direction Sense
- Practical Test Practice Questions And Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Dice Reasoning
- Dice Numbers In Dice Reasoning
- Classification Of Dice
- Constructed Vs Deconstructed Dice
- Tricks & Tips For Solving Dice Problems
- Practice MCQs With Detailed Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Alphanumeric Series Defined
- Alphanumeric Series In Reasoning Tests
- Tips & Strategies For Solving Alphanumeric Series
- Practice Sample Questions With Detailed Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept Of Mirror Image Reasoning Explained
- Important Terms In Mirror Image Reasoning
- Types Of Mirror Images
- Identifying Correct Mirror Image
- Finding Clock's Mirror Image
- Tips To Solve Mirror Images
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept & Overview Of Input-Output
- Input-Output In Competitive Exams
- Types Of Input-Output Problems
- Strategies, Tips & Tricks For Solving Reasoning Questions
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Finding The Odd One Out
- Tricks And Tips
- Strategies For Finding The Odd One Out
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding Key Concepts
- Exploring Different Ranking Types
- Formula And Application Of Order And Ranking
- Tips For Solving Order & Ranking
- Selected Practice Questions And Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Pipes & Cistern Aptitude
- Key Terminologies used in Pipes and Cisterns
- Pipes and Cisterns Formula with Examples
- Pipes and Cisterns Shortcut Tricks
- Tips For Solving Pipes & Cistern Problems
- Selected Questions & Answers For Practice (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Key Concept in Boats and Streams
- Formulas Of Boats & Streams
- Distance & Time Formula
- Tips For Solving Boats & Streams Questions
- Selected Practice Questions With Answers (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept of Mixture and Alligation
- Types Of Alligation Questions
- Formula for Solving Mixture & Alligation
- Tips For Solving Mixture And Alligation
- Selected Questions With Answers For Practice
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Time & Work Concepts
- Time And Work Formulas
- Tips For Solving Time And Work Questions
- Time And Work Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is Harmonic Progression(HP)?
- Formula to find the nth Term in Harmonic Progression
- Formula to find the Sum of the nth Term in HP
- What is Harmonic Mean?
- Harmonic Progression Solved Best MCQs
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is Mensuration in Maths?
- What are 2D figures in Mensuration?
- What are 3D figures in Mensuartion?
- Basic Terminologies In Mensuration
- Basic 2D Formulas in Mensuration
- Basic 3D Formulas in Mensuration
- 2D vs 3D in Mensuration
- Solved Questions With Solutions (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Relationship Between Time, Speed And Distance
- Conversion Units Time, Speed And Distance
- Average & Relative Speed: Two Trains Moving in the same or opposite direction
- Solved MCQs on Time, Speed And Distance
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basics Of Simplification
- BODMAS Rule in Simplification Explained
- Simplification & Approximation Relation
- Key Terms in Simplification
- Examples Of Simplification Techniques
- Simplification Questions With Solved Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Height And Distance Important Terms
- Right Angled Triangle In Trigonometry
- Trigonometric Ratios
- Solved Examples For Better Understanding
- Height And Distance Applications In Trigonometry
- Height And Distance Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Interest Types
- Simple Interest Vs. Compound Interest
- Selected Solved Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basic Concepts Of Profit And Loss
- Determining Selling Price
- Calculating Discounts
- Formulas For Calculating Profit And Loss
- Examples Of Profit And Loss
- Profit & Loss Questions With Detailed Solution
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Angle Of Elevation
- Key Terms Used In Angle Of Elevation
- Angle of Elevation Formula with Example
- Angle of Elevation vs. Angle of Depression
- Angle of Elevation MCQs with Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining HCF And LCM
- Calculation Methods Of HCF And LCM
- HCF By Prime Factorization Method
- LCM By Prime Factorization Method
- Difference Between HCF And LCM
- HCF & LCM Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is fraction and decimal?
- Understanding Decimal Fraction
- Place Value in Decimal Fraction
- Mathematical Operations with Decimal Fraction
- Practice with Solved Examples
- Summary
- Frequently Asked Questions
Table of content:
- All About Decimals
- All About Fractions
- How to Convert a Decimal into Fraction
- Simple vs Recurring Decimals
- Converting Recurring Decimals to Fractions
- Conversion Charts
- Practice Questions (With Solutions)
- Closing Thoughts
- Frequently Asked Questions
Table of content:
- What is Arithmetic Mean?
- Arithmetic Mean Formula- Ungroup Data & Group Data
- Merits of Arithmetic Mean
- Demerits of Arithmetic Mean
- Alternatives to Arithmetic Mean
- What is the Weighted Arithmetic Mean?
- Arithmetic vs. Geometric Mean
- Arithmetic Mean Application in Statistical Analysis
- Arithmetic Mean Practice Questions with Explanation
- Frequently Asked Questions
Table of content:
- What is Geometric Progression?
- Key Properties of Geometric Progression
- General Form Of Geometric Progression
- General Term or the Nth Term of Geometric Progression
- The sum of nth Terms of GP
- Types Of Geometric Progression
- Solved Questions and Answers of GP
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Average in Maths
- Average Formula in Maths
- Differentiating Types of Average
- How to Calculate Average of Negative Numbers?
- Practical Applications of Averages
- Average Questions For Practice
- Frequently Asked Questions
Table of content:
- What is Simple Interest in Maths?
- Simple Interest Formula Explained
- Simple Interest Formula for Years, Months & Days
- Simple Interest Examples & Practice Questions
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Mathematical Ratios
- Understanding Proportions Fundamentals
- Differentiating Ratios from Proportions
- Ratio and Proportion Formulas
- Properties of Ratio and Proportion
- How to Solve Ratio and Proportion Problems
- Ratio and Proportion Problems (With Solutions)
- Summary
- Frequently Asked Questions
Table of content:
- What is Number in Maths?
- Types of Numbers With Example
- Real vs Complex Numbers Explored
- Basic Operations on Numbers
- Practice Questions (With Solutions)
- Frequently Asked Questions
Table of content:
- What is Arithmetic Progression (AP) in Maths?
- Important Terminologies in Arithmetic Progression
- Basic Terms in Arithmetic Progression
- General Form Of Arithmetic Progression Series
- Types Of Arithmetic Progression
- Solved Questions With Explanation (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding Basic Concept
- Importance Of Train Problems In Aptitude
- Tips To Solve Train Problems
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Definition Of Mode
- Calculating Mode For Ungrouped Data
- Calculating Mode For Grouped Data
- Bimodal, Trimodal & Multimodal Distributions
- Comparing Mean, Median & Mode
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Important Concept In Races And Games
- Calculating Time, Speed & Distance
- Importance Of Races And Games Problems
- Sample Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Types Of Partnership
- Formula For Partnership Questions
- Tips To Solve Partnership Aptitude Questions
- Selected Partnership Questions (Practice MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Height And Distance- Trigonometry Basics With Question & Answer
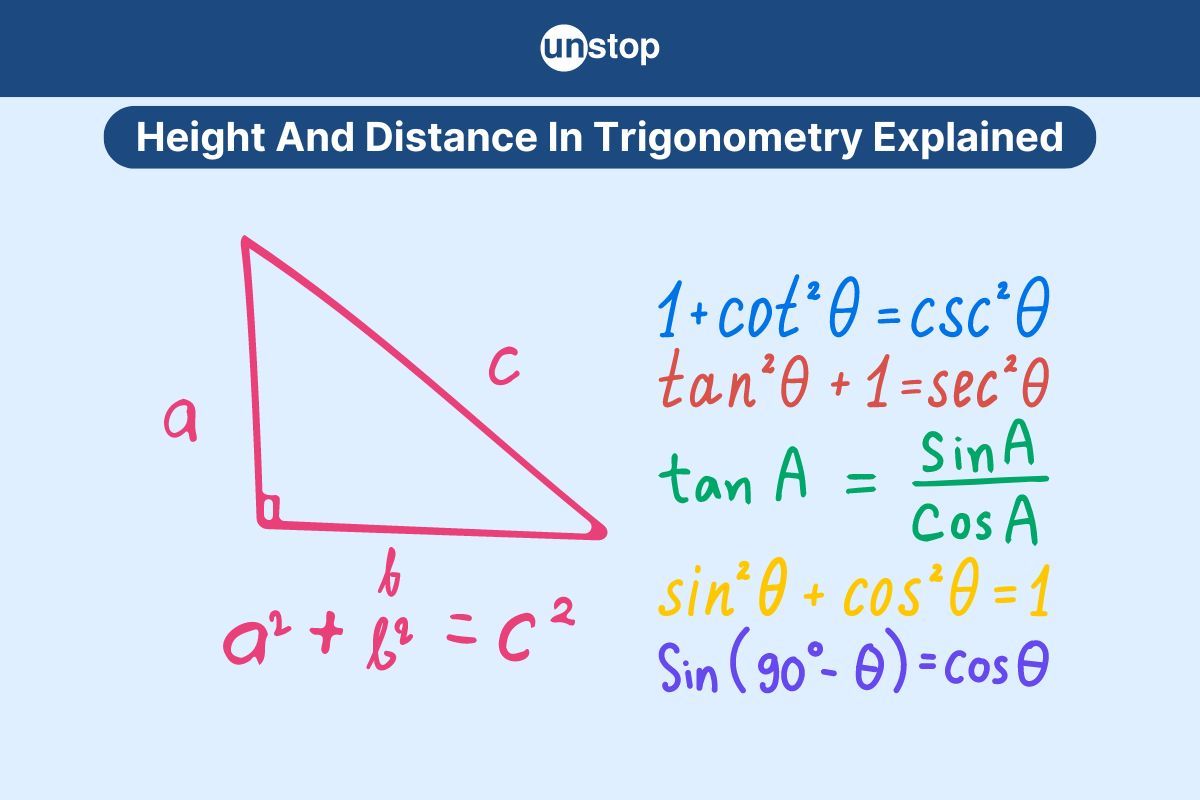
Regarding height and distance in trigonometry, we often use trigonometric ratios to solve problems involving measuring height and distance that are difficult to measure directly. Trigonometry provides us with the tools to calculate heights and distances accurately.
Height And Distance Important Terms
Important terms to understand when working with height and distance include the following definitions:
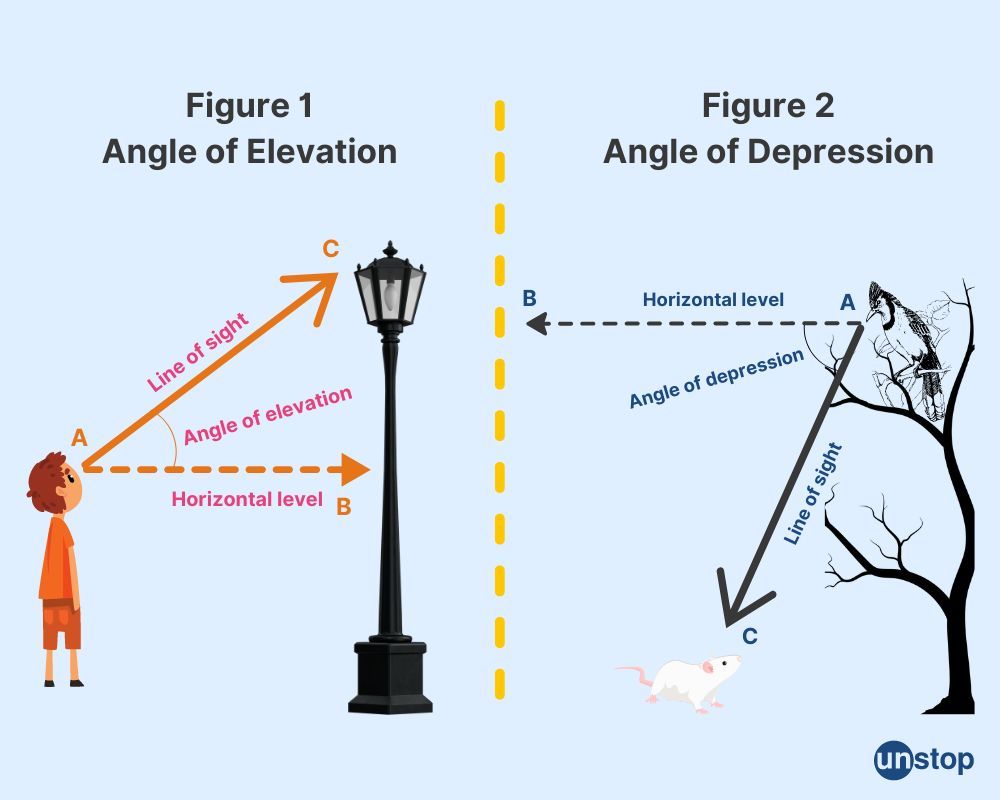
Line of Sight – The line drawn from the observer's eyes to the point being viewed on the object is known as the line of sight.
Angle of Elevation: The angle of elevation is the angle between the line of sight and the horizontal level when looking up at a point on an object.
The angle of Depression – The angle of depression is the angle formed by the line of sight with the horizontal level when an observer views a point on an object below them.
Right Angled Triangle In Trigonometry
Trigonometry deals with angles and sides of triangles, especially right triangles. The primary trigonometric functions are sine (sin), cosine (cos), and tangent (tan). Understanding these functions helps in calculating unknown angles or sides in a triangle.
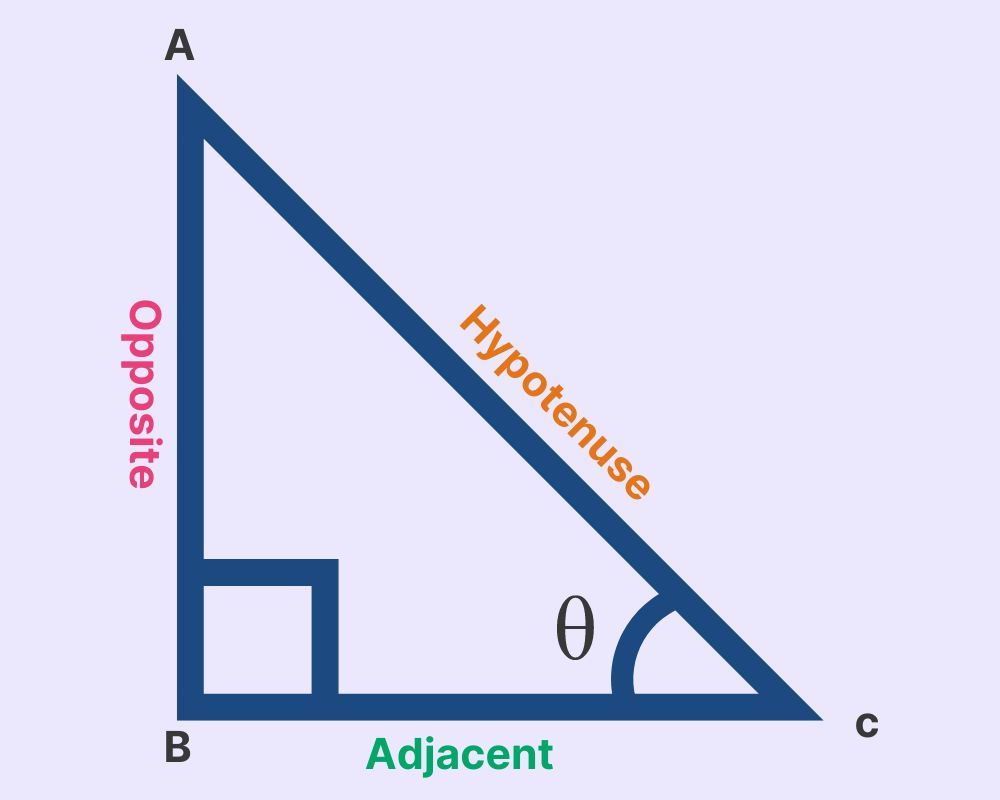
In the right triangle ABC,
sin θ = Opposite/Hypotenuse = AB/AC
cos θ = Adjacent/Hypotenuse = BC/AC
tan θ = Opposite/Adjacent = AB/BC
Formula For Height & Distance
The most important formula for calculating height and distance is the tangent formula. This formula involves using the tangent of an angle in a right triangle to determine the height or distance of an object. The formula is:
Tangent (θ) = Opposite / Adjacent
By rearranging this formula, you can solve for the height or distance depending on the information given. This formula is essential for various applications, such as surveying, navigation, and trigonometry.
Trigonometric Ratios
Here is a table of the most commonly used trigonometric ratios and degrees for height and distance:
| Angle (θ) | Sin(θ) | Cos(θ) | Tan(θ) |
|---|---|---|---|
| 0° | 0 | 1 | 0 |
| 30° | 1/2 | √3/2 | 1/√3 |
| 45° | √2/2 | √2/2 | 1 |
| 60° | √3/2 | 1/2 | √3 |
| 90° | 1 | 0 | Undefined |
Solved Examples For Better Understanding
Example 1: A person standing on a cliff spots a boat at an angle of elevation of 30 degrees. If the cliff is 50 meters high, how far is the boat from the base of the cliff?
Solution: Using the tangent ratio: tan(30) = opposite/adjacent, we find that the boat is approximately 28.87 meters away from the base of the cliff.
Example 2: A kite flying at an angle of elevation of 60 degrees has its string anchored to the ground. Given that the string is 100 meters long, find the height of the kite.
Solution: Applying sine: sin(60) = opposite/hypotenuse, we determine that the height of the kite is around 86.60 meters above ground level.
Click here to enhance and upskill your quantitative aptitude in trigonometry related to height and distance right away!
Height And Distance Applications In Trigonometry
Trigonometry's height and distance application covers various concepts like:
-
Measuring tall towers and huge mountains.
-
Determining how far the shore is from the sea.
-
Calculating the space between two heavenly bodies.
Tips For Height & Distance
Here are some tips for you to tackle problems related to height and distance:
-
Draw diagrams to visualize the problem accurately.
-
Label components clearly to avoid confusion while applying trigonometric functions.
-
Practice regularly to enhance your understanding and speed in solving such problems.
Height And Distance Practice Questions & Answers
Provided below are some of the selected questions related to height and distance with solved answers for practice to enhance your quantitative aptitude prowess:
Question 1. One side of a staircase is to be closed in by rectangular planks from the floor to each step. The width of each plank is 9 inches, and their height is successively 6 inches, 12 inches, 18 inches and so on. There are 24 planks required in total. Find the area in square feet.
(a) 112.5
(b) 107
(c) 118.5
(d) 105
Solution: a) 112.5
Explanation: The series of plank sizes would be:
0.75 × 0.5, 0.75 × 1, 0.75 × 1.5….0.75 × 12.
The sum of this AP is 112.5.
Question 2. The average height of 40 girls out of a class of 50 is 150 cm, and that of the remaining is 155 cm. The average height of the whole class is
(a) 151 cm
(b) 152 cm
(c) 156 cm
(d) 153 cm
Solution: a) 151 cm
Explanation: Average height of the whole class = (40 x 150 + 10 x 155)/50 = 151
Question 3. Raunak generally wears his father’s coat. Unfortunately, his cousin Vikas told him one day that he was wearing a coat of length more than his height by 15%. If the length of Raunak’s father’s coat is 345 cm, then find the actual length (in cm) of his coat.
(a) 110
(b) 345
(c) 300
(d) 105
Solution: c) 300
Explanation: Let Raunak’s height be H.
Then, H X 1.15 = 345,
H = 345/1.15 = 300.
Question 4. Increasing the height of an equilateral triangle by 30% poses a question: what is the maximum percentage increase in the base's length to limit the area increase to a maximum of 90%?
(a) 33.33%
(b) 20.67%
(c) 46.15%
(d) 25.34%
Solution: c) 46.15%
Explanation: The area of a triangle depends on the product: base x height.
Since the height increases by 30% and the area has to increase by 90% overall, the following PCG will give the answer. Let 100 be the original area.
100(30% increase, + 30 effect of increase in base)= 130
130( effect of increase in height)=190 therefore , 60/130 X 100 = 46.15%
Question 5. When the height of an equilateral triangle measures 10 cm, what will its area be?
(a) 100√3 cm2
(b) 100/3√3 cm2
(c) 100/3 cm2
(d) 200√3/3 cm2
Solution: b) 100/3√3 cm2
Explanation: h = 10 cm
H = a√3/2⇒ a= 10 X 2/√3 = 20/√3cm
Area = 1/2 X 20/√3 X 10 = 100/√3cm2 or 100√3/3cm2
Question 6. The volume of a right circular cone is 100p cm3, and its height is 12 cm. Find its curved surface area.
(a) 130 π cm2
(b) 65 π cm2
(c) 204 π cm2
(d) 65 π cm2
Solution: b) 65 π cm2
Explanation: Volume of a cone = πr2h/3
Then 100 π= πr212/3
⇒r=5 cm
Curved surface area = πrl
l = √h2 + √r2
⇒122 + 52 = 13
πrl = π x 13 x 5 = 65 π cm2.
Question 7. The diameters of the two cones are equal. If their slant height is in the ratio 5: 7, find the ratio of their curved surface areas.
(a) 25:7
(b) 25:49
(c) 5:49
(d) 5:7
Solution: d) 5:7
Explanation: Let the radius of the two cones be = x cm
Let the slant height of 1st cone = 5 cm and
The slant height of 2nd cone = 7 cm
Then the ratio of covered surface area = πX 5/ πX 7 = 5 : 7
Question 8. The ratio of radii of a cylinder to that of a cone is 1 : 2. If their heights are equal, find the ratio of their volumes.
(a) 3:1
(b) 2:3
(c) 3:4
(d) 2: 1
Solution: c) 3:4
Here's a step-by-step explanation:
Let the radius of cylinder = 1(r)
Then the radius of cone be = 2(R)
Then, as per question = πr2h/πR2h/3
⇒3πr2h/πR2h
⇒3r2/3R2
⇒3:4
Question 9. The ratio of the areas of two isosceles triangles with equal angles is 16:25. What is the ratio of their corresponding heights?
(a) 4/5
(b) 4/6
(c) 5/4
(d) 5/7
Solution: a) 4/5
Explanation: (Ratio of corresponding sides)2= Ratio of area of similar triangles
The ratio of corresponding sides in this question= √16/√25 = 4/5.
Question 10. What is the height of a tower that casts a shadow of 60 meters on the ground, given that a vertical stick 30 meters long casts a shadow of 20 meters simultaneously?
(a) 105 m
(b) 120 m
(c) 25 m
(d) 200 m
Solution: b) 120m
Explanation: When the length of the stick = 30 m,
then the length of shadow = 20 m
Here, the length formula is = 2 x shadow.
Assuming the same angle of inclination of the sun, the tower's length that casts a shadow of 60 m 2 x 60m = 120m, i.e. the height of the tower = 120 m.
Conclusion
The exploration of trigonometry's application in determining heights and distances has shed light on the fundamental principles governing these calculations. By delving into trigonometric formulas and ratios, solving practical examples, and understanding the relationships between various elements, a comprehensive understanding of height and distance problems has been achieved.
Identifying different types of triangles and their relevance to real-world scenarios further solidifies the practicality of these mathematical concepts.
Time For A Small Quiz
Frequently Asked Questions (FAQs)
1. What is the significance of understanding heights and distances in trigonometry?
Knowing about heights and distances in trigonometry is super important for things like building stuff, finding your way, and measuring land. You can calculate how tall or far away something is using trigonometric ratios.
2. How are Trigonometry Formulas explained for calculating heights and distances?
Trigonometry formulas play a vital role in calculating height and distance by providing relationships between angles and side lengths of triangles. These formulas, such as sine, cosine, and tangent, help determine unknown measurements accurately.
3. Can you explain the relationship between height and distance in trigonometry?
The relationship between height and distance in trigonometry involves using angles and side lengths of triangles to determine the height or distance of an object from a specific point. This relationship is based on trigonometric functions like sine, cosine, and tangent.
4. Why are solved examples important for better understanding heights and distances?
Solved examples provide practical applications of trigonometric concepts related to heights and distance. By analyzing these examples step by step, learners can grasp how to apply trigonometry principles effectively to solve height and distance-related problems.
5. How do types of triangles play a role in understanding height and distance concepts?
Different types of triangles (such as right-angled triangles) are fundamental in understanding height and distance concepts in trigonometry. These triangles' properties help determine angles and side lengths and apply trigonometric ratios to calculate heights or distances accurately.
Suggested reads:
- What Is Mensuration: Formulas With Top Selected Question & Answer
- Harmonic Progression- Formula With Selected Questions & Answers
- Mixture And Alligation: Explained With Selected Question & Answer
- Boats And Streams: Formula, Top Question With Solution (Aptitude)
- Clock Questions: Selected Question & Answer (Aptitude) Explained
Instinctively, I fall for nature, music, humour, reading, writing, listening, travelling, observing, learning, unlearning, friendship, exercise, etc., all these from the cradle to the grave- that's ME! It's my irrefutable belief in the uniqueness of all. I'll vehemently defend your right to be your best while I expect the same from you!
Comments
Add commentLogin to continue reading
And access exclusive content, personalized recommendations, and career-boosting opportunities.
Subscribe
to our newsletter



K.SHIVA GANESH 1 week ago
Khushal Bansal 2 weeks ago
YASH KAPOOR 1 month ago
Lahari Datta 1 month ago
avneesh kaur 1 month ago
Sanchita roy 1 month ago