Dice Reasoning: Types & Tricks Explained With Question & Answer 

9 mins read

Understanding how to use dice reasoning effectively can improve students' critical thinking abilities by up to 50%. This can be particularly useful when faced with challenging exam questions or decision-making during job interviews.

By incorporating dice reasoning into their study and preparation routines, students can enhance their cognitive abilities and increase their chances of success in exams and job placements.

Importance Of Dice Reasoning

Let us study the importance of dice reasoning in detail:

Competitive Exams

In competitive exams, dice-based questions assess candidates' ability to apply logic and reasoning under time constraints. These questions often require candidates to decipher patterns, sequences, and relationships between numbers displayed on the faces of the dice.

Enhances Problem-Solving

Dice reasoning plays a crucial role in logical reasoning tests by challenging individuals to think critically and analytically. By incorporating dice into reasoning assessments, individuals are required to visualize and manipulate various outcomes, thus significantly enhancing their problem-solving skills.

Dice Numbers In Dice Reasoning

Let us understand how dice numbers work in dice reasoning:

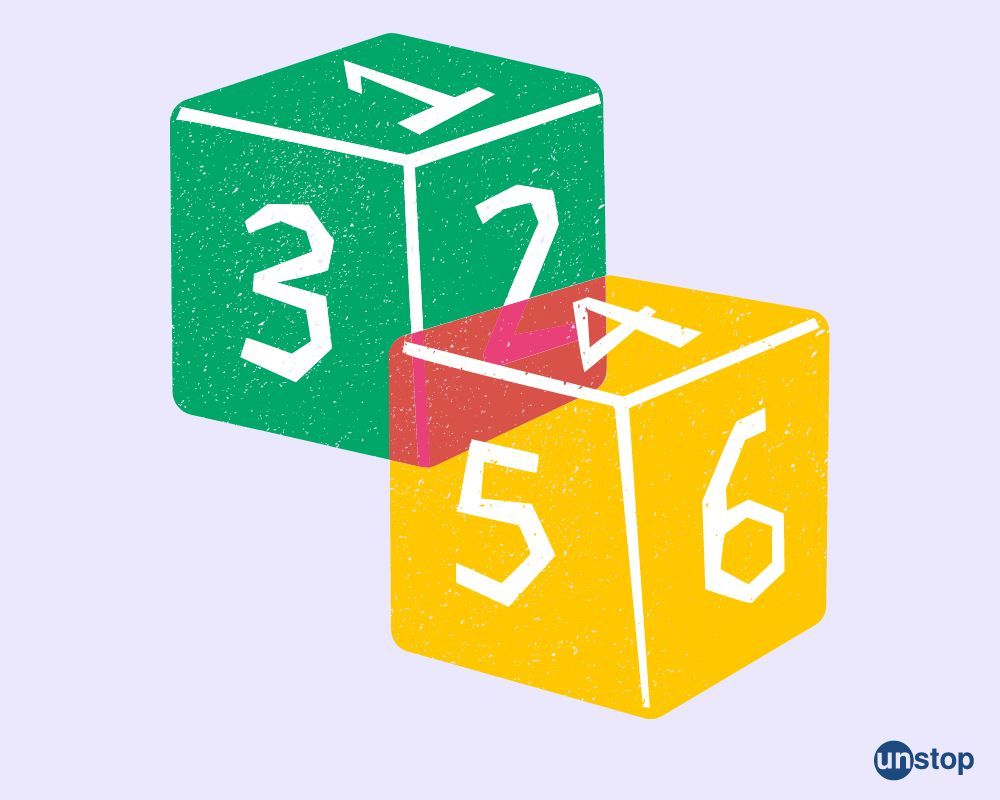
Arrangement

Dice are numbered in a way that the sum of numbers on opposite faces always equals the total number of faces plus one. For instance, a standard six-sided dice has opposite faces, adding up to seven.

Relationship

Opposite faces on dice are designed always to equal the total number of sides plus one when you add the numbers together. This principle applies to all types of dice, ensuring fairness and randomness in games and probability calculations.

Numbering Scheme Rules

The numbering scheme on dice follows specific rules to maintain consistency and fairness. Each face is marked with a different number, ensuring that no two faces with adjacent numbers are placed next to each other.

This arrangement prevents bias and ensures random outcomes in various applications such as board games and gambling.

Classification Of Dice

Let us study the classification of dice in dice reasoning:

Base Dice

Base dice are primarily cuboid in shape, featuring numbers on each face from 1 to 6. They are commonly used in traditional board games. These dice offer a straightforward approach to gaming, with clear outcomes determined by the number rolled.

Open Dice

In contrast, open dice have an irregular shape, which sets them apart from the standard cuboid form. This uniqueness introduces a level of complexity and unpredictability to games that utilize them. Open dice often come with varying numbers of faces, ranging from four to twenty or more.

Standard Dice

Standard dice adhere to the classic six-sided cuboid design, ensuring uniformity and consistency in gameplay. Standard dice maintain a predictable outcome due to their symmetrical structure.

Ordinary Dice

Ordinary dice encompass a broader category, including base and open dice variations. Also, ordinary dice introduce an element of surprise and strategy.

Constructed Vs Deconstructed Dice

Let us study the constructed and deconstructed dice in dice reasoning:

Defined Problems

Constructed dice involve assembling a three-dimensional cube with numbers placed on each face. On the other hand, deconstructed dice problems require you to visualize a two-dimensional flat shape representing a die. Imagine a cube where 1 is opposite 6, 2 is opposite 5, and 3 is opposite 4.

To understand constructed dice, imagine folding the flat shape into a cube to see how the numbers align on different faces. For deconstructed dice, picture laying out the cube and identifying which numbers are opposite. Visualize a flattened-out cube showing all six faces with numbers arranged in a specific pattern.

Tricks & Tips For Solving Dice Problems

Let us study some of the tricks and tips for solving dice problems in reasoning:

Approach Strategies

When tackling dice reasoning problems, start by visualizing the dice's different positions. Understand that each face has a specific rule: the sum of numbers on opposite sides is always seven. Familiarize yourself with the rules governing dice and the possible outcomes in various scenarios.

Regular Practice

Regular practice is crucial for mastering dice reasoning. By practising consistently, students can improve their aptitude for deciphering complex dice problems efficiently. Moreover, practice helps in developing a keen eye for detail when analyzing dice configurations.

Grasp The Logic

Dice reasoning plays a crucial role in logical reasoning questions, especially in interviews and mock tests. By grasping the logic behind dice configurations, candidates can effectively tackle verbal reasoning problems. This involves analyzing the patterns of symbols and numbers on the different faces of a dice.

Mental Manipulation

One effective technique for tackling verbal reasoning questions related to dice is to visualize the dice's rotations in your mind. This mental manipulation helps you determine how the symbols or numbers would appear on each face after rotation..

Practice MCQs With Detailed Answers

Solving a variety of practice questions is key to mastering dice-based multiple-choice questions (MCQs). Provided below are some of the best-selected dice-based reasoning questions and answers:

Question 1: If two dice are thrown simultaneously, what is the probability of getting two numbers whose product is even?

a) 1/2

b) 1/4

c) 3/4

d) 1/3

Answer: c) 3/4

Explanation: In a throw of two dice, there are 36 possible outcomes. Out of these, 27 outcomes have the product of two numbers as even. So, the required probability = 27/36 = 3/4

Question 2: A die is thrown twice; what is the probability that the sum of the numbers appearing on the dice is 11?

a) 1/9

b) 1/12

c) 1/6

d) 1/18

Answer: d) 1/18

Explanation: There are 36 possible outcomes when a die is thrown twice. The event of getting the sum of the numbers as 11 is 5,6 and 6,5. So, there are 2 favourable outcomes. Therefore, the required probability = 2/36 = 1/18.

Question 3: If two dice are thrown simultaneously, what is the probability of getting two numbers whose product is odd?

a) 1/2

b) 1/4

c) 3/4

d) 1/3

Answer: b) 1/4

Explanation: In a throw of two dice, there are 36 possible outcomes. Out of these, 9 outcomes have the product of two numbers as odd. So, the required probability = 9/36 = 1/4.

Question 4: A die is thrown twice; what is the probability that the sum of the numbers appearing on the dice is 7?

a) 1/9

b) 1/6

c) 1/12

d) 1/8

Answer: b) 1/6

Explanation: There are 36 possible outcomes when a die is thrown twice. The event of getting the sum of the numbers as 7 is (1,6), (2,5), (3,4), (4,3), (5,2), (6,1). So, there are 6 favourable outcomes. Therefore, the required probability = 6/36 = 1/6.

Question 5: If two dice are thrown simultaneously, what is the probability of getting two numbers whose product is prime?

a) 1/2

b) 1/4

c) 1/6

d) 1/3

Answer: c) 1/6

Explanation: In a throw of two dice, there are 36 possible outcomes. Out of these, 15 outcomes have the product of two numbers as prime. So, the required probability = 15/36 = 5/12.

Question 6: A die is thrown twice; what is the probability that the sum of the numbers appearing on the dice is 10?

a) 1/9

b) 1/12

c) 1/6

d) 1/8

Answer: b) 1/12

Explanation: There are 36 possible outcomes when a die is thrown twice. The event of getting the sum of the numbers as 10 is (4,6), (5,5), (6,4). So, there are 3 favourable outcomes. Therefore, the required probability = 3/36 = 1/12.

Question 7: If two dice are thrown simultaneously, what is the probability of getting two numbers whose product is divisible by 3?

a) 1/2

b) 1/4

c) 3/4

d) 1/3

Answer: a) 1/2

Explanation: In a throw of two dice, there are 36 possible outcomes. Out of these, 18 outcomes have the product of two numbers divisible by 3. So, the required probability = 18/36 = 1/2.

Question 8: A die is thrown twice; what is the probability that the sum of the numbers appearing on the dice is 9?

a) 1/9

b) 1/12

c) 1/6

d) 1/8

Answer: a) 1/9

Explanation: There are 36 possible outcomes when a die is thrown twice. The event of getting the sum of the numbers as 9 is (3,6), (4,5), (5,4), (6,3). So, there are 4 favourable outcomes. Therefore, the required probability = 4/36 = 1/9.

Question 9: A die is thrown twice; what is the probability that the difference between the numbers appearing on the dice is 1?

a) 1/9

b) 5/18

c) 1/6

d) 1/8

Answer: b) 5/18

Explanation: There are 36 possible outcomes when a die is thrown twice. The event of getting the difference of the numbers as 1 is (1,2), (2,1), (2,3), (3,2), (3,4), (4,3), (4,5), (5,4), (5,6), (6,5). So, there are 10 favourable outcomes. Therefore, the required probability = 10/36 = 5/18.

Question 10: A die is thrown twice; what is the probability that the difference of the numbers appearing on the dice is 2?

a) 1/9

b) 2/9

c) 1/12

d) 1/8

Answer: b) 2/9

Explanation: There are 36 possible outcomes when a die is thrown twice. The event of getting the difference of the numbers as 2 is (1,3), (3,1), (2,4), (4,2), (3,5), (5,3), (4,6), (6,4). So, there are 8 favourable outcomes. Therefore, the required probability = 8/36 = 2/9.

Conclusion

Mastering dice reasoning unlocks a valuable tool for enhancing your problem-solving skills and acing various MCQs. Practice solving problems and challenging yourself with diverse scenarios to solidify your understanding.

Remember, the more you engage with dice reasoning, the sharper your analytical skills become. Keep honing your abilities, and watch as your proficiency in reasoning reaches new heights.

Quiz Time To Test Your Mind

Frequently Asked Questions (FAQs)

1. What is the significance of understanding dice in reasoning?

Understanding dice in reasoning is crucial as it enhances problem-solving skills, aids in tackling verbal reasoning questions effectively, and improves overall cognitive abilities by mastering dice-based MCQs.

2. How are dice numbers classified?

Dice numbers are categorized based on their positions and relationships on the dice's faces. This classification helps differentiate between regular and irregular dice patterns for various reasoning exercises.

3. What distinguishes constructed from deconstructed dice?

Constructed dice refer to standard, solid structures with predefined number arrangements on opposite faces, while deconstructed dice involve rearranging these numbers to form new configurations. This distinction impacts the complexity of reasoning tasks involving dice manipulation.

4. How does understanding dice enhance problem-solving skills?

Understanding dice enables individuals to visualize spatial relationships, analyze patterns efficiently, and develop logical thinking strategies essential for solving complex problems across various domains beyond just mathematical reasoning.

5. Why are verbal reasoning questions important when dealing with dice problems?

Verbal reasoning questions provide context and additional challenges when working with dice problems, requiring individuals to interpret instructions accurately, apply critical thinking skills, and make informed decisions based on the given information.

Suggested reads: 

• Data Interpretation: Definition, Steps, & Practice Questions
• What Is Number In Maths: History, Types, Operations & More
• Ratio and Proportion: Mastering Fundamentals (+ Solved Questions)
• Arithmetic Progression- Notation, Formulas, Questions And Answers
• Geometric Progression- Definition, Formula, Questions & Answers