Decimal Fraction: Find Types, Operations & Practice Questions

You’ve probably heard of decimals and fractions in Mathematics, but ever wondered about decimal fractions? How do they work and why do they matter?

Dive into this blog post to unravel the mysteries of decimal fractions, understand their significance, and master their applications in everyday life.

Understanding Decimal Fraction

What is fraction and decimal?

Let’s start with the basics - understanding fractions and decimals:

Decimals

Decimals are a way to represent fractions or parts of a whole using the base-ten system. They consist of a whole number part followed by a decimal point and numbers representing parts of one whole. For example, 0.5 represents half, while 0.75 represents three-fourths.

Decimals offer a precise way to express values that fall between two whole numbers, making them essential in various fields like mathematics, finance, and science. Understanding decimals is crucial for tasks involving measurements, calculations, and comparisons.

Fractions

Fractions, on the other hand, represent parts of a whole or a group. They consist of a numerator (top number) representing the part being considered and a denominator (bottom number) indicating the total number of parts that make up the whole. For instance, 1/2 represents one out of two equal parts.

Fractions are used in everyday situations such as cooking recipes, construction measurements, and determining probabilities in statistics. Mastering fractions is fundamental for accurately performing operations like addition, subtraction, multiplication, division, etc.

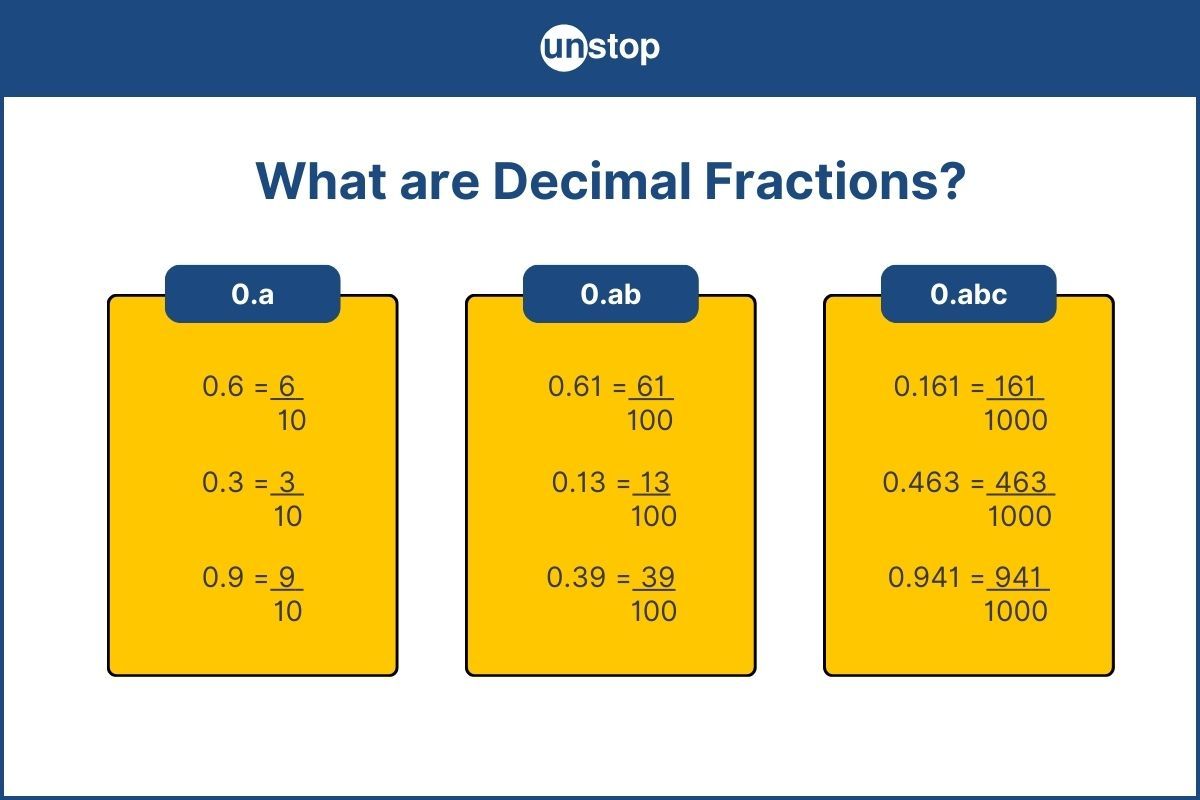
What are decimal fractions?

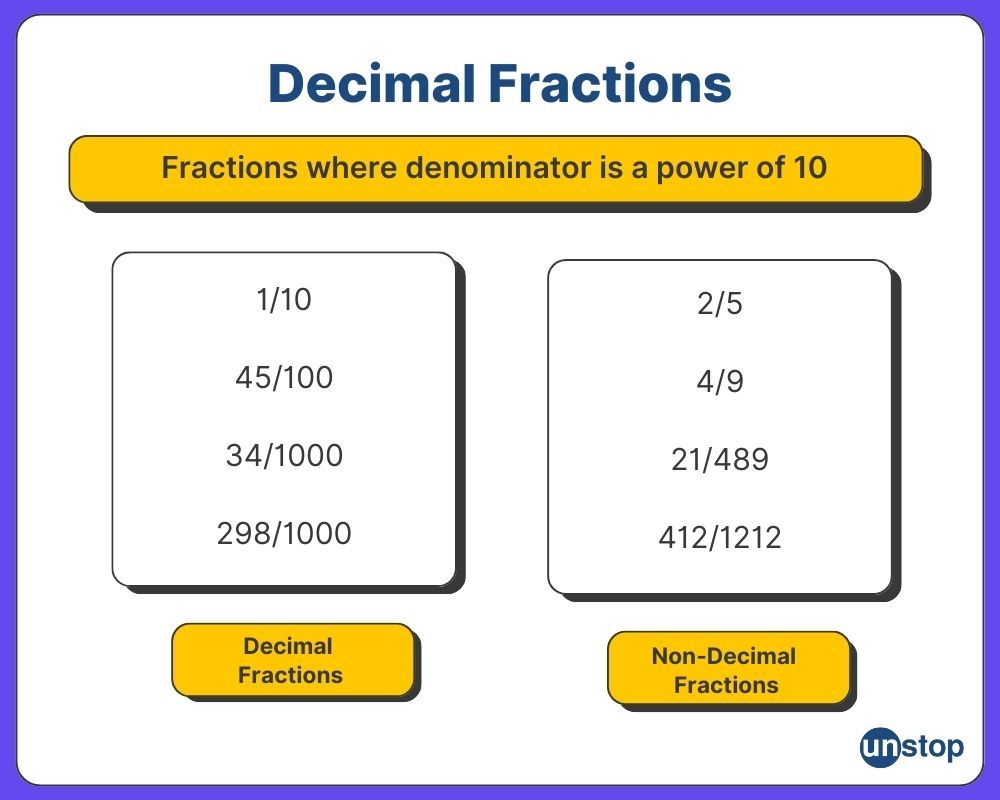
Definition: Decimal fractions are fractions where the denominator is 10 or its multiples. They represent parts of a whole in decimal form. Decimal fractions include digits after the decimal point, indicating values smaller than one whole unit.

A decimal fraction simplifies mathematical operations such as division and multiplication due to its base-10 system. Decimal fractions are crucial in representing parts of a whole precisely. For instance, 0.5 represents half, 0.25 represents one-fourth, and so on.

Decimal Fraction vs. Other Fractions

In a decimal fraction, the denominator is always a power of 10, such as 10, 100, 1000, and so on. This characteristic sets decimal fractions apart from other types of fractions like common fractions or mixed numbers.

Key Differences:

• Simplicity: Decimal fractions offer a straightforward way to represent parts of a whole due to their base 10 denominators.
• Ease of Comparison: Comparing decimal fractions is often simpler than comparing other types of fractions due to their uniformity.
• Convenience in Calculations: Decimal fractions are commonly used in everyday calculations like money, measurements, and percentages for their ease of computation.

Decimal Fraction: Common Types

• Terminating decimal fractions are those that end after a certain number of digits. These decimals can be expressed as fractions and have a finite number of digits after the decimal point. For example, 0.5, 0.75, and 0.25 are all terminating decimals.
• Non-terminating decimal fractions, on the other hand, continue indefinitely without repeating. They cannot be expressed as simple fractions and go on infinitely without stopping. An example is π (pi), which is approximately equal to 3.14159.
• Recurring decimals have a pattern that repeats continuously, such as 0.3333..., while non-recurring decimals do not follow any repetitive pattern like √2 ≈ 1.41421356.

Place Value in Decimal Fraction

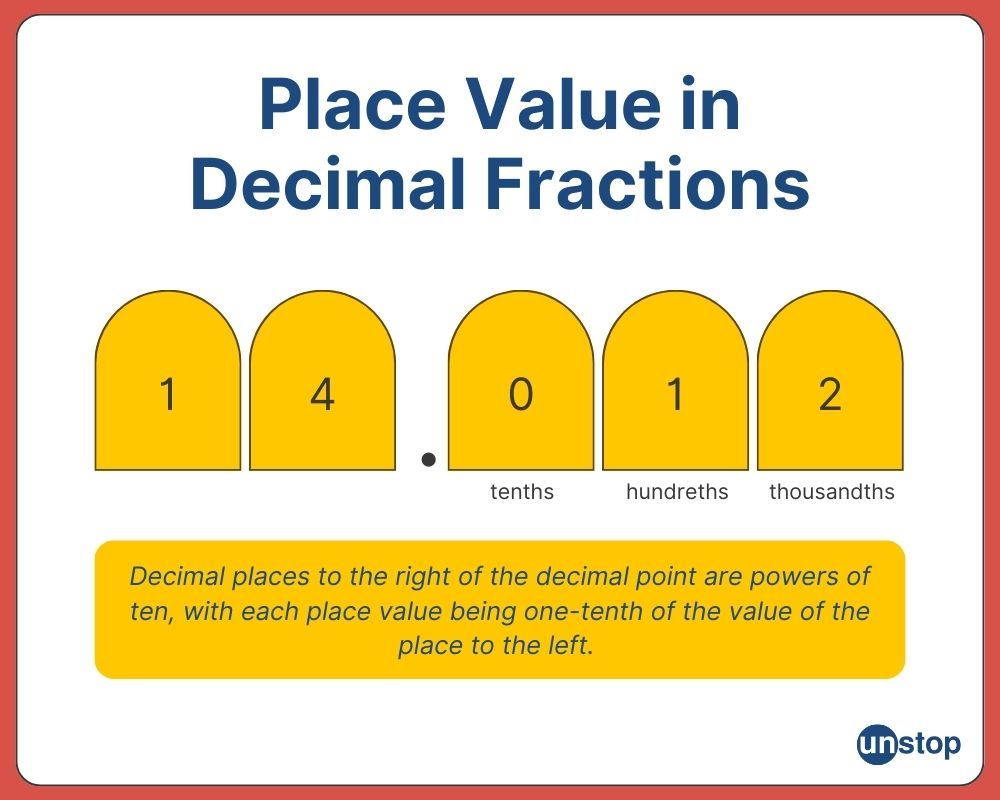
In decimal notation, each digit's position indicates its value relative to the whole number. This is referred to as the place value.

Decimal places to the right of the decimal point are powers of ten, with each place value being one-tenth of the place to its immediate left. This pattern continues infinitely, allowing for precise representation of fractional quantities.

For instance, the first digit after the decimal point represents tenths. The second digit after the decimal point signifies hundredths, representing one part out of a hundred. Continuing this pattern, the third digit after the decimal point denotes thousandths, and so on.

This granular breakdown enables precise measurements and calculations in various fields like science and finance. Each place value becomes smaller by a factor of ten than its preceding value. This level of detail is essential for intricate calculations where precision matters significantly.

Understanding place value aids in manipulating and comparing decimal fractions efficiently. It simplifies operations such as addition, subtraction, multiplication, and division by providing a clear framework for working with numbers accurately.

Mathematical Operations with Decimal Fraction

Addition and Subtraction

When adding or subtracting decimal fractions, it's crucial to ensure that the decimal points are properly aligned. This alignment simplifies the process and avoids errors in the calculation. To achieve this, place the numbers one below the other so that their decimal points are in a straight line.

To add or subtract decimal fractions accurately, follow these steps:

1. Step 1: Line up the decimal points of the numbers being added or subtracted.
2. Step 2: Add zeros to the right of a number if it has fewer decimal places than the other number.
3. Step 3: Perform addition or subtraction as you would with whole numbers, taking care to align digits according to place value after adjusting for any additional zeros.

Tips for Precision

• When adding or subtracting decimal fractions, always double-check that the decimal points are correctly aligned before proceeding with the operation.
• Use grid paper or ruled paper to assist in maintaining alignment when working on multiple-digit decimals.
• Practice regularly to enhance your speed and accuracy in adding and subtracting decimal fractions.

Example:

Add 12.4 + 0.75

1. Since 12.4 has only one digit after the decimal, add a zero to make the number of decimal places the same (12.40).
2. Then, align the decimals one below the other, making sure the decimal points are in the same column.
3. Add each digit column like you would with whole numbers.
4. Final answer: 12.40+0.75 =13.15

Subtract 8.1 - 2.34

1. Since 8.1 has only one digit after the decimal, add a zero to make the number of decimal places the same (8.10)
2. Align the decimals one below the other, making sure the decimal points are in the same column.
3. Subtract each digit column as you would with whole numbers, borrowing when necessary.
4. Final answer: 8.10-2.34 = 5.76

Multiplication with Decimal Fraction

Multiplying and dividing decimal fractions follow similar principles but with a key step involving the decimal point. Here's a breakdown of multiplication

Multiplication of Decimal Fractions:

1. Ignore the decimal points for now: Treat both decimals like whole numbers for the moment.
2. Perform the multiplication: Multiply the digits as you would normally multiply whole numbers.
3. Count the total number of digits behind the decimal point in BOTH of the original decimal numbers.
4. Place the decimal point in the product (answer): The decimal point in the answer should be shifted to the right by the total number of digits you counted in step 3.

Example: Multiply 2.54 x 1.3

• Ignore decimals for now: 254 x 13 = 3302
• Count decimal digits: 2.54 has 2 digits after the decimal and 1.3 has 1 digit after the decimal. Total digits = 2 + 1 = 3
• Place the decimal point: 3.302. (Move the decimal 3 places to the right because of the 3 total digits after the decimal in the original numbers).

Quick tip: To multiply by multiples of 10, shift the decimal point to the right by as many places as there are zeros in the multiple of 10. For example, when multiplying 3.25 by 100, move the decimal point two places to the right to get 325.

Division of Decimal Fractions

When dividing decimal fractions, first convert them into whole numbers by moving the decimals to the right. Then perform division as usual. Lastly, place the decimal point in the quotient directly above its position in the dividend. Let’s go through this step-by-step:

1. Convert the divisor (the number you're dividing by) into a whole number: This is where the decimal point manipulation comes in.
   • Count the number of digits to the right of the decimal point in the divisor.
   • Move the decimal point in BOTH the divisor and the dividend (the number you're dividing) the same number of places to the right. This essentially multiplies the dividend by a power of 10 (10, 100, 1000, etc.) and creates a whole number divisor for easier division.
2. Perform the division: Now that the divisor is a whole number, you can perform the division like you would normally divide whole numbers.
3. Place the decimal point in the quotient (answer): The decimal point in the quotient (answer) will have the same number of digits to the right as it did in the ORIGINAL dividend (before converting the divisor).

Example: Divide 3.24 by 1.2

• Convert divisor to a whole number: There is one digit after the decimal in 1.2 (the divisor). So, move the decimal in BOTH 3.24 (dividend) and 1.2 (divisor) one place to the right: 32.4 / 12
• Perform the division: 32.4 / 12 = 2.7
• Place the decimal point: The original dividend (3.24) has two digits after the decimal point. So, the answer (quotient) 2.7 also has two digits after the decimal point (2.70).

Tips for Placing Decimal Points

• When dividing a smaller number by a larger one, ensure that your quotient is less than 1.
• Always align your decimals vertically when performing long division with decimal fractions.

Practice with Solved Examples

Q1. If a barrel initially holds 25.7 liters of water and 12.3 liters are consumed, find the remaining water in a barrel.

Options:
a) 12.1
b) 13.2
c) 15.4
d) 13.4

Answer: d) 13.4 liters

Solution Explained:

The solution involves subtracting the amount consumed from the initial quantity to determine the remaining water.

• Total Capacity of the barrel: 25.7
• Consumed Capacity: 12.3
• Remaining Capacity: 25.7 - 12.3 = 13.4

Q2. Determine the total weight of bags, if three bags weigh 5.8 kg, 6.4 kg, and 7.2 kg respectively.

Options:
a) 19.1
b) 16.2
c) 19.4
d) 14

Answer: c) 19.4 kg

Solution Explained:

The solution involves adding the individual weight of all three bags.

• Weight of bag 1: 5.8 kg
• Weight of bag 2: 6.4 kg
• Weight of bag 3: 7.2 kg
• Total weight of bags: 5.8+7.2+6.4 = 19.4 kg

Q3. Express 0.125 as a fraction in its simplest form.

Options:
a) 1/2
b) 6/25
c) 1/8
d) 2/16

Answer: 1/8

Solution Explained:

The decimal point indicates the place value of each digit.

• In this case, 0.125 is equivalent to 125 divided by 1000 because the digit 1 is in the thousandth place: 0.125=125/10000
• Now, we simplify the fraction by finding the greatest common divisor (GCD) of the numerator and denominator, which is 125.
• Dividing both the numerator and denominator by 125 gets the fraction: 1/8

Q4. Write 0.6 as a ratio of two integers.

Options:
a) 2/3
b) 3/5
c) 1/5
d) 2/5

Answer: b) 3/5

Solution Explained:

• To write 0.6 as a ratio of two integers, we first need to express 0.6 as a fraction.
• Since 0.6 is equivalent to 6 tenths, we can represent it as 6/10.
• To simplify this fraction, we find the greatest common divisor (GCD) of 6 and 10, which is 2.
• Dividing both the numerator and the denominator by 2: 3 /5
• So, 0.60.6 as a ratio of two integers is 3/5.

Q5. A cake recipe calls for 3.2 cups of flour, but Shivani only has a measuring cup that holds 0.75 cups. How many times can she fill the measuring cup to get enough flour for the recipe?

Options:
a) 4 times
b) 6 times
c) 1.2 times
d) 2.3 times

Answer: a) 4 times

Solution Explained:

We need to find out how many times 0.75 cups (divisor) goes into 3.2 cups (dividend) to get the total number of fillings needed. Here are the steps to follow

Convert the divisor (0.75 cups) to a whole number:

• There are two digits after the decimal point in 0.75.
• Move the decimal point in BOTH the dividend (3.2 cups) and divisor (0.75 cups) two places to the right. This multiplies the dividend by 100 (without changing its value) and creates a whole number divisor (75) for easier division.
• 3.2 cups becomes 320 cups (move decimal 2 places to the right)
• 0.75 cups becomes 75 cups (move decimal 2 places to the right)
• Now, we can perform the division with whole numbers: 320/75 = 4.2
• Sarah can fill the measuring cup 4 times (with some leftover flour) to get enough flour for the recipe (3.2 cups).

Q6. If 0.142857=x, what is the value of 1000x?

Options:
a) 1428.57
b) 142.857
c) 14.2857
d) 1.42857

Answer: b) 142.857

Solution Explained

Here’s a step-by-step solution:

• x=0.142857; Now, multiply x by 1000:
• 1000x=1000×0.142857
• So, 1000x is equal to 142.857

Q7. Express 0.7777… as a fraction in simplest form.

Options:
a) 7/9
b) 7/10
c) 7/11
d) 8/7

Answer: a) 7/9

Solution Explained:

Here’s a step-by-step solution:

• Identify the repeating pattern, which is 77.
• Determine the number of digits in the repeating pattern, which is 1.
• Write an equation to represent the repeating decimal. Since there's only one repeating digit, we multiply by 10 to shift the repeating part: 10x=7.7777...
• Subtract the original equation from the multiplied one to eliminate the repeating part:
  • 10x−x=7.7777...−0.7777...
  • 9x=7
• Solve for x: x=7/9​
• So, 0.7777... as a fraction in simplest form is 7/9.

Q8. What is the product of 0.125 and 0.16 when expressed as a decimal fraction?

Options:
a) 0.0025
b) 0.32
c) 0.2
d) 0.02

Answer: d) 0.02

Solution Explained

Here are the steps to multiply decimal fractions like 0.125 and 0.16 and place the decimal point in the correct position in the product:

• Ignore the decimal points and multiply: Perform the multiplication as if there were no decimal points present. So, for 0.125 and 0.16, multiply 125X16. This gives the result = 2000
• Count the total decimal places: We have 3 decimal places in 0.125 and 2 decimal places in 0.16, for a total of 5 decimal places.
• Place the decimal point in the product: Count from the right of the product to place the decimal point, ensuring the total number of decimal places matches the count from the previous step. For 0.02000, we have 5 decimal places as we should.
• So, the result of multiplying 0.125 and 0.16 is 0.02000. We can simplify this to 0.02 by removing the trailing zeros.

Q9. If 0.07x is equal to 0.105, what is the value of x?

Options:
a) 1.75
b) 1.25
c) 1.5
d) 2.5

Answer: d) 1.5

Solution Explained:

• If 0.07x=0.105, then this simplifies to x= 0.105/0.07.
• Now, we perform the division: x= 0.105/0.07.
• Since there are two digits after the decimal in the divisor, place the decimal two points to the right in both dividend and divisor. This brings x=10.5/7
• After performing the division, and placing the decimal point two points to the right brings x = 1.50 or 1.5

Q10. Solve the equation 2x−0.3=0.7x+0.02 for x.

Options:
a) 0.25
b) 0.35
c) 0.14
d) 0.21

Answer: a) 0.25

Solution Explained:

Here’s a step-by-step solution:

• Move the x-terms to one side of the equation and the constant terms to the other side.
• Combine like terms and solve for x.
  • Step 1: 2x−0.7x=0.3+0.02
  • Step 2: 1.3x=0.32
  • Step 3: x=0.32​/1.3
• After dividing, x = 0.25 (rounding off 0.246)

Explore this topic, along with other important concepts of Quant on Unstop, and race ahead of the competition.

Summary

By grasping the fundamentals of decimals, fractions, and decimal fractions, one can master the concept for real-world applications in various fields. Practice with solved examples to solidify your understanding and boost your confidence in dealing with decimal fractions effortlessly. Keep exploring different scenarios to enhance your skills further and apply these concepts in everyday situations. Embrace the power of decimal fractions in your mathematical toolkit!

Frequently Asked Questions

1. What is the difference between decimals and fractions?

Decimals represent numbers in a continuous number line, while fractions represent parts of a whole or a set.

2. What are some techniques for addition and subtraction with decimal fractions?

When adding or subtracting decimal fractions, align the decimal points vertically and fill in any missing places with zeros. Then, perform the operation as usual. Finally, place the decimal point in the answer directly below the other decimals.

3. Can you explain how multiplication works with decimal fractions?

To multiply decimal fractions, ignore the decimals initially and multiply the numbers as whole numbers. Count the total number of digits after the decimals in both factors and place the decimal point in the product that many places from the right.

4. Why is understanding place value crucial when working with decimal fractions?

Understanding place value is vital as it determines the value of each digit in a number. In decimal fractions, each digit's position signifies its value relative to others. This knowledge helps in correctly reading and manipulating decimal numbers effectively.

Suggested Reads:

• Simplification- Basic Formulas With Practice Questions & Answers
• Height And Distance- Trigonometry Basics With Question & Answer 
• Mixture And Alligation: Explained With Selected Question & Answer
• Boats And Streams: Formula, Top Question With Solution (Aptitude)
• Clock Questions: Selected Question & Answer (Aptitude) Explained