Table of content:
- Step 1: The Groundwork
- Step 2: The Three Sections
- Step 3: Practice Makes Improvement
- The Secret Ingredient
Table of content:
- Understanding Basics Of Number Series
- Types Of Number Series Reasoning Questions
- Addition & Multiplication In Number Series
- Square & Cube-Based Number Series Patterns
- Missing Terms & Arranging Sequences
- Tricks & Tips For Solving Number Series
- Selected Sample Questions With Detailed Solutions
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Terminologies Used in Blood Relation Questions
- What is a Family Tree?
- Types Of Blood Relation Questions
- Verbal Reasoning in Blood Relation
- Tips For Solving Blood Relation Questions
- Common Pitfalls while Solving Questions
- Selected Questions (MCQs) For Practice With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Letter Series In Logical Reasoning
- Types Of Letter Series Patterns
- Tips For Solving Letter Series Reasoning
- Best Practice Question Samples With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basics of Problems On Age-Related Questions
- Formula to Solve Problems on Ages-Related Questions
- Types of Age-Related Questions & Examples
- Tips & Tricks for Solving Ages Problems
- Best MCQs on Problems on ages with solved answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Definition of Calendar
- Understanding Days of the Week
- Understanding the Concept of Leap Year
- Concept of Odd Days in a Century
- Tips For Calendar Reasoning Questions
- Top 10 Calendar Questions & Answers For Practice (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basic Concepts of Clock
- Structure of a Clock
- Angle Equilavalence in Clock
- Tips For Solving Clock Questions
- Selected Clock Questions & Answers (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding The Concept Of Direction Sense
- Tips For Effective Problem-Solving In Direction Sense
- Practical Test Practice Questions And Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Dice Reasoning
- Dice Numbers In Dice Reasoning
- Classification Of Dice
- Constructed Vs Deconstructed Dice
- Tricks & Tips For Solving Dice Problems
- Practice MCQs With Detailed Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Alphanumeric Series Defined
- Alphanumeric Series In Reasoning Tests
- Tips & Strategies For Solving Alphanumeric Series
- Practice Sample Questions With Detailed Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept Of Mirror Image Reasoning Explained
- Important Terms In Mirror Image Reasoning
- Types Of Mirror Images
- Identifying Correct Mirror Image
- Finding Clock's Mirror Image
- Tips To Solve Mirror Images
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept & Overview Of Input-Output
- Input-Output In Competitive Exams
- Types Of Input-Output Problems
- Strategies, Tips & Tricks For Solving Reasoning Questions
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Finding The Odd One Out
- Tricks And Tips
- Strategies For Finding The Odd One Out
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding Key Concepts
- Exploring Different Ranking Types
- Formula And Application Of Order And Ranking
- Tips For Solving Order & Ranking
- Selected Practice Questions And Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Pipes & Cistern Aptitude
- Key Terminologies used in Pipes and Cisterns
- Pipes and Cisterns Formula with Examples
- Pipes and Cisterns Shortcut Tricks
- Tips For Solving Pipes & Cistern Problems
- Selected Questions & Answers For Practice (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Key Concept in Boats and Streams
- Formulas Of Boats & Streams
- Distance & Time Formula
- Tips For Solving Boats & Streams Questions
- Selected Practice Questions With Answers (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept of Mixture and Alligation
- Types Of Alligation Questions
- Formula for Solving Mixture & Alligation
- Tips For Solving Mixture And Alligation
- Selected Questions With Answers For Practice
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Time & Work Concepts
- Time And Work Formulas
- Tips For Solving Time And Work Questions
- Time And Work Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is Harmonic Progression(HP)?
- Formula to find the nth Term in Harmonic Progression
- Formula to find the Sum of the nth Term in HP
- What is Harmonic Mean?
- Harmonic Progression Solved Best MCQs
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is Mensuration in Maths?
- What are 2D figures in Mensuration?
- What are 3D figures in Mensuartion?
- Basic Terminologies In Mensuration
- Basic 2D Formulas in Mensuration
- Basic 3D Formulas in Mensuration
- 2D vs 3D in Mensuration
- Solved Questions With Solutions (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Relationship Between Time, Speed And Distance
- Conversion Units Time, Speed And Distance
- Average & Relative Speed: Two Trains Moving in the same or opposite direction
- Solved MCQs on Time, Speed And Distance
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basics Of Simplification
- BODMAS Rule in Simplification Explained
- Simplification & Approximation Relation
- Key Terms in Simplification
- Examples Of Simplification Techniques
- Simplification Questions With Solved Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Height And Distance Important Terms
- Right Angled Triangle In Trigonometry
- Trigonometric Ratios
- Solved Examples For Better Understanding
- Height And Distance Applications In Trigonometry
- Height And Distance Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Interest Types
- Simple Interest Vs. Compound Interest
- Selected Solved Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basic Concepts Of Profit And Loss
- Determining Selling Price
- Calculating Discounts
- Formulas For Calculating Profit And Loss
- Examples Of Profit And Loss
- Profit & Loss Questions With Detailed Solution
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Angle Of Elevation
- Key Terms Used In Angle Of Elevation
- Angle of Elevation Formula with Example
- Angle of Elevation vs. Angle of Depression
- Angle of Elevation MCQs with Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining HCF And LCM
- Calculation Methods Of HCF And LCM
- HCF By Prime Factorization Method
- LCM By Prime Factorization Method
- Difference Between HCF And LCM
- HCF & LCM Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is fraction and decimal?
- Understanding Decimal Fraction
- Place Value in Decimal Fraction
- Mathematical Operations with Decimal Fraction
- Practice with Solved Examples
- Summary
- Frequently Asked Questions
Table of content:
- All About Decimals
- All About Fractions
- How to Convert a Decimal into Fraction
- Simple vs Recurring Decimals
- Converting Recurring Decimals to Fractions
- Conversion Charts
- Practice Questions (With Solutions)
- Closing Thoughts
- Frequently Asked Questions
Table of content:
- What is Arithmetic Mean?
- Arithmetic Mean Formula- Ungroup Data & Group Data
- Merits of Arithmetic Mean
- Demerits of Arithmetic Mean
- Alternatives to Arithmetic Mean
- What is the Weighted Arithmetic Mean?
- Arithmetic vs. Geometric Mean
- Arithmetic Mean Application in Statistical Analysis
- Arithmetic Mean Practice Questions with Explanation
- Frequently Asked Questions
Table of content:
- What is Geometric Progression?
- Key Properties of Geometric Progression
- General Form Of Geometric Progression
- General Term or the Nth Term of Geometric Progression
- The sum of nth Terms of GP
- Types Of Geometric Progression
- Solved Questions and Answers of GP
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Average in Maths
- Average Formula in Maths
- Differentiating Types of Average
- How to Calculate Average of Negative Numbers?
- Practical Applications of Averages
- Average Questions For Practice
- Frequently Asked Questions
Table of content:
- What is Simple Interest in Maths?
- Simple Interest Formula Explained
- Simple Interest Formula for Years, Months & Days
- Simple Interest Examples & Practice Questions
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Mathematical Ratios
- Understanding Proportions Fundamentals
- Differentiating Ratios from Proportions
- Ratio and Proportion Formulas
- Properties of Ratio and Proportion
- How to Solve Ratio and Proportion Problems
- Ratio and Proportion Problems (With Solutions)
- Summary
- Frequently Asked Questions
Table of content:
- What is Number in Maths?
- Types of Numbers With Example
- Real vs Complex Numbers Explored
- Basic Operations on Numbers
- Practice Questions (With Solutions)
- Frequently Asked Questions
Table of content:
- What is Arithmetic Progression (AP) in Maths?
- Important Terminologies in Arithmetic Progression
- Basic Terms in Arithmetic Progression
- General Form Of Arithmetic Progression Series
- Types Of Arithmetic Progression
- Solved Questions With Explanation (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding Basic Concept
- Importance Of Train Problems In Aptitude
- Tips To Solve Train Problems
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Definition Of Mode
- Calculating Mode For Ungrouped Data
- Calculating Mode For Grouped Data
- Bimodal, Trimodal & Multimodal Distributions
- Comparing Mean, Median & Mode
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Important Concept In Races And Games
- Calculating Time, Speed & Distance
- Importance Of Races And Games Problems
- Sample Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Types Of Partnership
- Formula For Partnership Questions
- Tips To Solve Partnership Aptitude Questions
- Selected Partnership Questions (Practice MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Arithmetic Mean: Definition, Formulas, Solved Questions & Answers
Understanding the arithmetic mean is key to analyzing data effectively. It's not about complex formulas but rather finding the average value of a set of numbers. Whether you're working on college assignments, financial reports, or statistical analysis, knowing how to calculate the arithmetic mean is essential for making informed decisions.
What is Arithmetic Mean?
Arithmetic mean ((often called the average) is a statistical measure that calculates the central value of a data set by summing all the numbers and dividing by the total count. It represents the measure of central tendency that is typical of a data set.
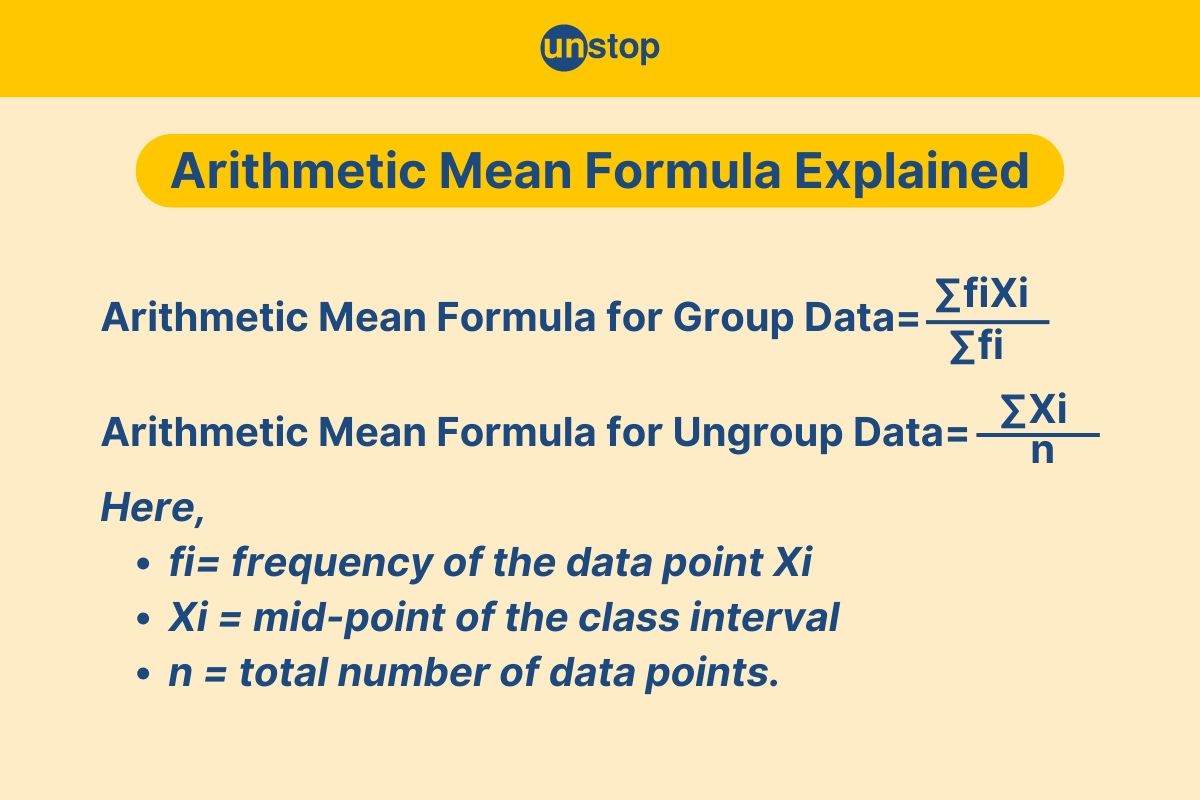
Arithmetic Mean Formula: Ungroup Data & Group Data
Now, let us study the arithmetic formulas for ungroup data and group data accordingly:
Ungroup Data Formula
Ungrouped data in the context of arithmetic mean refers to raw, individual data points that have not been organized into groups or categories. Each value in the dataset is considered separately. The arithmetic mean for ungrouped data is calculated by adding all the individual data points and dividing by the number of data points.
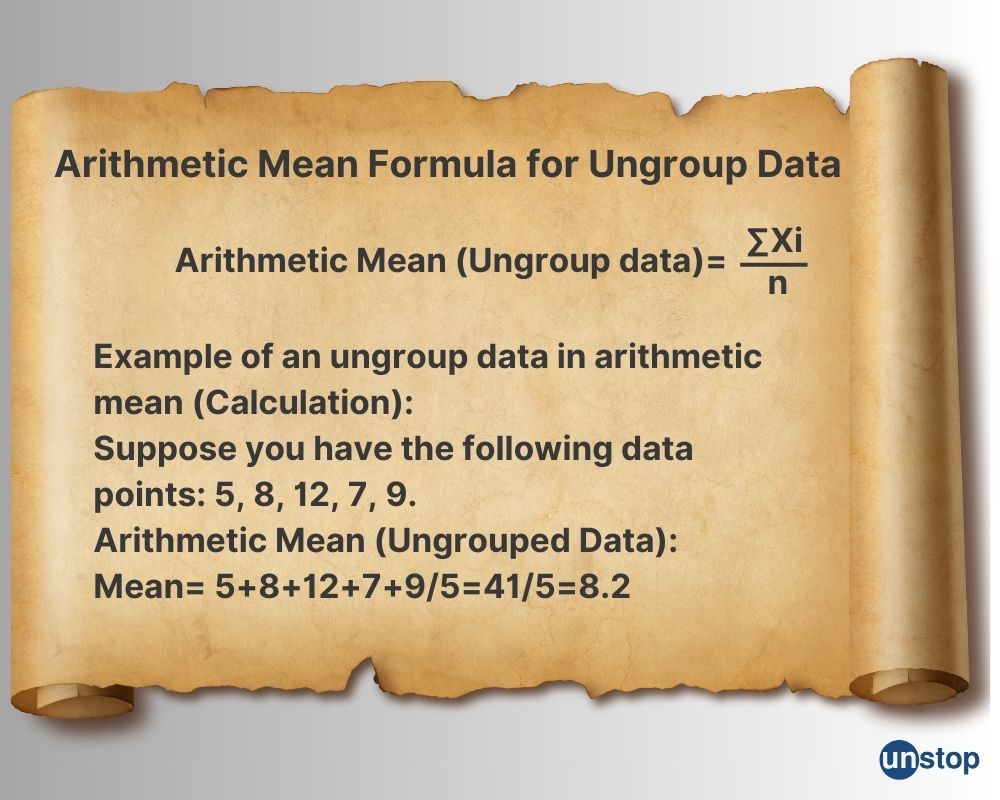
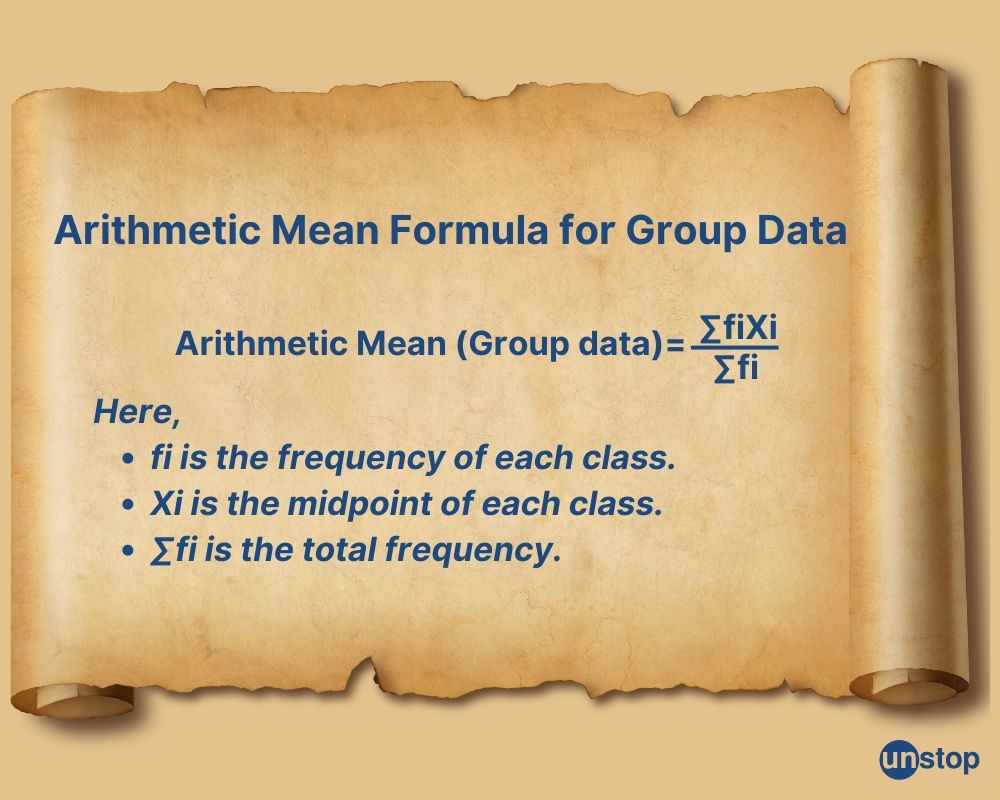
Group Data Formula
Grouped data in the context of arithmetic mean refers to data that has been organized into classes or intervals, along with the frequency of data points in each interval. Instead of working with individual data points, the data is grouped, making it easier to handle large datasets.
Example of Group Data Calculation
Suppose the data is grouped as follows:
| Class Interval | Frequency (fi) |
|---|---|
| 0 - 10 | 4 |
| 10 - 20 | 6 |
| 20 - 30 | 5 |
| 30 - 40 | 3 |
-
Calculate the midpoint (Xi) for each class:
- Midpoint of 0 - 10 = 5
- Midpoint of 10 - 20 = 15
- Midpoint of 20 - 30 = 25
- Midpoint of 30 - 40 = 35
-
Calculate the arithmetic mean:
Mean=(5×4)+(15×6)+(25×5)+(35×3)/4+6+5+3=20+90+125+105/18=340/18≈18.89
Steps for calculating arithmetic mean:
-
To calculate the arithmetic mean, first, sum up all the numbers in the dataset. This step is crucial as it ensures that every data point contributes accurately to the final result.
-
After summing up all the numbers, you will obtain a total which represents the combined value of all data points in the set.
-
Next, divide the sum of all numbers by the total count of values in the dataset. This step involves taking the sum calculated earlier and dividing it by the number of data points present.
-
By dividing the sum by the count, you arrive at a single value that represents the average or arithmetic mean of all values in the dataset.
Merits of Arithmetic Mean
Let us study the merits of arithmetic mean:
The arithmetic mean has several merits, making it a valuable tool in statistics and data analysis. Here are some of the key advantages:
- Simplicity: It's a straightforward concept to understand and calculate. Anyone can easily grasp the idea of adding all the values in a dataset and dividing by the number of values. This makes it a widely accessible and easy-to-explain measure.
- Based on all observations: The arithmetic mean uses information from every data point in a set. This ensures all values contribute to the final value, unlike some methods that might exclude outliers.
- Single unique value: The arithmetic mean provides a single, concise value that represents the "centre" of the data. This makes it easy to compare different datasets or track changes in a data set over time.
- Relatively stable under random fluctuations: While outliers can affect the mean, it's generally less sensitive to random fluctuations in the data compared to some other measures of central tendency (like the median). This makes it a good choice for summarizing data that might have some natural variation.
- Algebraic properties: The arithmetic mean has well-defined mathematical properties that allow for further analysis. For example, deviations from the mean can be easily calculated and used in statistical tests and further calculations.
- Widely used as a reference point: Many other statistical measures, like standard deviation and variance, are calculated based on the arithmetic mean. This makes it a central reference point for understanding the distribution of data.
Demerits of Arithmetic Mean
Let's also look at some of the demerits of arithmetic mean:
Sensitive to Outliers
Outliers, i.e., extreme values, can significantly skew the arithmetic mean, especially in small datasets. For instance, in a set of income data where most values are moderate but a few are extremely high, those outliers can heavily influence the mean. This can lead to a misleading representation of the average income for the entire group.
Challenge of Skewed Distributions
Skewed distributions, where data points are not evenly distributed, pose challenges for the arithmetic mean. In cases with a significant skew towards one end of the distribution, the mean may not accurately reflect the central tendency.
For example, in a class where most students score around 70% on a test but a few score either very low or very high, the mean might not accurately represent the typical performance.
When dealing with financial data or performance metrics, relying solely on the arithmetic mean without considering its limitations can lead to flawed decision-making processes. For example, if an investor uses the mean return on investment to make decisions without accounting for outliers that significantly impact returns, they may underestimate risks and overestimate potential gains.
To mitigate the limitations of arithmetic mean and provide a more accurate representation of data, it is crucial to complement it with other measures such as median and mode. By incorporating these additional measures, analysts can gain a more comprehensive understanding of the dataset and make informed decisions based on a holistic view rather than solely relying on the arithmetic mean.
Alternatives to Arithmetic Mean
Now, let us study some of the alternative measures other than the arithmetic mean:
Median
The median represents the middle value in a dataset when arranged in ascending order. It is less influenced by extreme values compared to the arithmetic mean. For skewed data distributions, the median can provide a more accurate representation of the central tendency.
When dealing with income data, for example, where extreme values like outliers can significantly impact the mean, using the median can offer a better reflection of the typical income level. This makes it a preferred choice in scenarios where extreme values might distort the average.
Mode
The mode refers to the most frequently occurring value in a dataset. It is particularly useful when analyzing categorical data or data with distinct peaks or clusters. Unlike the mean and median, the mode can be applied to non-numeric data as well.
In cases where we are interested in identifying the most common response in a survey or determining popular product preferences, utilizing the mode can provide valuable insights that other measures of central tendency may not capture.
Choosing the Right Measure
Deciding between using the mean, median, or mode depends on various factors such as data distribution, presence of outliers, and the nature of the data being analyzed. Understanding these factors is crucial in selecting an appropriate measure that accurately represents the central tendency.
For instance, if analyzing household sizes in a community where most households have similar sizes but a few exceptionally large families exist, opting for the median would offer a better representation than relying on the mean. This ensures that outliers do not skew the central value.
What is the Weighted Arithmetic Mean?
Weighted arithmetic mean is a type of average where different data points are given varying levels of importance or weight. This method ensures that certain values contribute more significantly to the final average than others.
When calculating a weighted average, each data point is multiplied by its respective weight, and the sum of these products is divided by the total sum of weights. This approach allows for a more accurate representation of the data set by considering the significance of each value.
Significance
By incorporating weights into the calculation, weighted arithmetic mean offers a more precise measure when certain data points hold greater importance. For instance, in financial analysis, revenues from different product lines may have varying impacts on overall performance. Assigning appropriate weights to these revenues can provide a more accurate reflection of the company's financial health.
In scenarios where some values are inherently more significant than others, such as grades in a course where exams carry more weight than homework assignments, using weighted averages ensures that the final result aligns with the true impact of each component.
Arithmetic vs. Geometric Mean
The arithmetic mean is the simple average of a set of numbers, calculated by adding them together and dividing by the count of numbers. On the other hand, geometric mean involves multiplying all numbers together and then taking the nth root, where n is the count of numbers.
When comparing the two, arithmetic mean is more straightforward to calculate as it only requires addition and division. In contrast, finding the geometric mean involves multiplication and roots, making it slightly more complex mathematically.
Here’s a quick look at the differences between arithmetic mean and geometric mean:
| Arithmetic Mean | Geometric Mean |
|---|---|
| The sum of values divided by the number of values | The nth root of the product of N values |
| Suitable for data with linear growth patterns | Suitable for data with exponential growth patterns |
| Sensitive to extreme values in the dataset | Less sensitive to extreme values in the dataset |
| Used for averaging quantities that add up | Used for averaging quantities that multiply together |
Let’s study these differences in detail:
Application Variances
Arithmetic mean is commonly used for general arithmetic calculations like calculating averages in everyday scenarios such as test scores or household expenses. It provides a good representation of a typical value in a dataset.
Geometric mean, however, is preferred when dealing with ratios or growth rates. For instance, in finance, it's used to calculate gross returns on investments over multiple periods. It gives more weight to lower values in a dataset compared to arithmetic mean.
Specific Scenarios
In scenarios where data points vary significantly or there are outliers present, using arithmetic mean might not accurately represent the central tendency of the data due to its sensitivity to extreme values. Geometric mean can be more appropriate in such cases as it's less affected by outliers.
When dealing with datasets involving quantities that grow at a consistent rate over time, like population growth or interest rates, geometric mean provides a better measure of average growth compared to arithmetic mean. It accounts for compounding effects over multiple periods.
Averaging Data Points
Both means have their unique applications based on the nature of the dataset and the specific goal of the analysis. While arithmetic mean gives equal weightage to all data points, geometric mean adjusts for variations in growth rates or ratios within a dataset.
In financial contexts where analyzing investment performance is crucial, using geometric mean helps provide a more accurate representation of returns over time compared to arithmetic mean. It considers compounding effects that occur across different time periods.
Arithmetic Mean Application in Statistical Analysis
Hypothesis Testing
Analysts use the arithmetic mean in statistical analysis to examine hypotheses about population parameters. Analysts determine the likelihood of observing such results by comparing sample means to hypothesised values by chance.
When conducting hypothesis tests, analysts calculate test statistics that quantify the difference between sample means and expected values. These statistics follow specific distributions, allowing analysts to assess the significance of their findings.
Through hypothesis testing, analysts can draw conclusions about population characteristics with a certain level of confidence. This process aids in making informed decisions based on data.
Confidence Intervals
In statistical analysis, analysts leverage the arithmetic mean to construct confidence intervals around sample estimates. These intervals provide a range within which the true population parameter is likely to lie. Analysts determine the width of confidence intervals by considering variability and sample size, aiding in decision-making processes.
Confidence intervals offer insights into the precision of estimates derived from sample data. Analysts interpret these intervals in conjunction with point estimates to assess the reliability of their calculations. This approach enhances the robustness of statistical analyses by accounting for uncertainty and variability inherent in data.
Contextual Interpretation
Interpreting results based on the arithmetic mean requires careful consideration of contextual factors. While the mean provides a summary measure of central tendency, its interpretation hinges on understanding the nature of the data and potential outliers. Analysts must evaluate whether the mean accurately represents the dataset or if, other measures may be more appropriate.
In real-world scenarios, interpreting arithmetic means involves assessing how well they reflect underlying patterns or trends. For instance, in financial analysis, a high mean return on investment may mask significant variations among individual assets. By examining dispersion around the mean, analysts gain insights into data distribution and make informed decisions.
Arithmetic Mean Practice Questions with Explanation
Q1. What is the arithmetic mean of 5, 10, and 15?
Options:
a) 8
b) 12
c) 14
d) 10
Answer: d) 10
Solution Explained
For the numbers 5, 10, and 15, the arithmetic mean is calculated as follows:
- The sum of all digits is the numerator: 5+10+15 = 30
- The count of all digits is the denominator: 3
- Arithmetic Mean is Numerator/Denominator: 30/3 =10
- So, the arithmetic mean of 5, 10, and 15 is 10.
Q2. The average of the three numbers is 20. If two of the numbers are 15 and 25, what is the third number?
Options:
a) 20
b) 30
c) 35
d) 25
Answer: a) 20
Solution Explained
To solve this, consider three numbers: 15, 25 and x.
- If the average of three numbers is 20, then the calculation follows:
- Use the average formula: (15+25+x)/3=20
- Add the numerator and multiply the RHS with the denominator from LHS: (40+x)= 60 (20*3)
- This brings x = 60-40= 20
- So, the value of the third number is 20.
Q3. If the arithmetic mean of 4 numbers is 25, what is their sum?
Options:
a) 90
b) 80
c) 100
d) 110
Answer: c) 100
Solution Explained
Here’s how to solve this:
- Let the four numbers be a,b,c, and d
- The arithmetic mean of these four numbers will be: (a+b+c+d)/4=25
- This brings the total of a+b+c+d=100
- So, the sum of these four numbers is 100.
Q4. The average weight of 5 students is 60 kg. If one more student, weighing 72 kg, is added, what is the new average?
Options:
a) 62
b) 63
c) 66
d) 68
Answer: a) 62
Solution Explained
Here’s how to solve this:
- As per the formula for arithmetic mean:
- Average weight of students = (sum of weight of students)/Number of students
- If the average weight of 5 students is 60 kg, then we can calculate the total sum of the weight of the five students as follows:
- The sum of the weight of five students: 60*5 = 300.
- If one more student, of weight of 72 kg is added, then the total weight of all 6 students: 300+72 =372
- Now, the new average of 6 students is:
- Sum of weight of students/Number of students: 372/6 =62
- So the new average is 62 kg.
Q5. The arithmetic mean of 6 numbers is 12. If one number is excluded, the arithmetic mean becomes 10. What is the excluded number?
Options:
a) 8
b) 10
c) 22
d) 6
Answer: c) 22
Solution Explained
Here’s how to solve this:
- If the mean of six numbers is 12, then the sum of six numbers: 12*6 = 72
- If one number is excluded, then the arithmetic mean becomes 10.
- Thus the sum of five numbers: 5*10 = 50
- The excluded number is the difference between the sum of the 6 numbers and the sum of the 5 numbers: 72-50 =22
- So, the excluded number is 22.
Q7. The average of 2x−1 and 3x+5 is 20. What is the value of x?
Options:
a) 8.8
b) 5
c) 6
d) 7.2
Answer: d) 7.2
Solution Explained
If the average of 2x-1 and 3x+5 = 20, then we can use the formula for the arithmetic mean to calculate the value of x.
- As per the formula, (2x-1+3x+5)/2=20. The calculation for this is as follows:
- Add the numerator: 2x-1+3x+5 = 5x+4
- Take the denominator to the RHS and multiply by 20: 20*2 = 40
- Isolate x further by taking 4 on RHS and subtracting from the total: 40-4=36
- Isolate x completely by dividing the RHS from 5: 36/5 = 7.2
- So the value of x = 7.2
Q8. The arithmetic mean of 10 numbers is 15. If each number is multiplied by 2, what is the new arithmetic mean?
Options:
a) 35
b) 25
c) 20
d) 30
Answer: a) 35
Solution Explained
Here’s how to solve this:
- If the arithmetic mean of 10 numbers is 15, then using the formula, we can get the sum of the 10 numbers.
- The sum of 10 numbers/10 = 15
- The sum of 10 numbers: 15*10= 150
- Now, if each number is multiplied by 2, the overall sum will be multiplied by 2 as well. Thus, the new mean will become:
- 2 (Sum of 10 numbers)/10 = 2*150/10 = 300/10 = 30
- So, the new arithmetic mean is 30.
Q9. The average age of a group of 8 friends is 24 years. If a new friend, aged 32, joins them, what is the new average?
Options:
a) 25
b) 28
c) 27
d) 26
Answer: a) 25
Solution Explained
- To solve this, consider the total sum of the ages of 8 friends as x.
- If the average age is 24, then as per the arithmetic mean formula: x/8 = 24
- Thus, the sum of ages of 8 friends: 8*24 =192
- Sum of ages of friends after 9th friend joins: 192+32 = 224
- Now, the average age of 9 friends will be New Sum/9: 224/9 = 24.88 (nearly 25)
- Therefore the correct answer will be 25.
Q10. The average of 7 numbers is 18. If one number is removed and the average becomes 17, what is the number removed?
Options:
a) 12
b) 13
c) 24
d) 26
Answer: c) 24
Solution Explained
Here’s how to solve this,
- If the average of 7 numbers is 18, then the sum of 7 numbers: 7*18 =126
- If one number is removed, then the average becomes 17. Thus, the sum of 6 numbers: 6*17 = 102
- The removed number is the difference between the sum of the 7 numbers and the sum of the 6 numbers: 126-102 = 24
- The removed number is 24.
Q11. The average of 5 consecutive even numbers is 20. What is the largest number?
Options:
a) 24
b) 28
c) 30
d) 26
Answer: a) 24
Solution Explained
Here’s how to solve it:
- Let’s consider the smallest even number in the set to be x.
- Since consecutive even numbers differ by 2, the other numbers in the series in ascending order are x+2, x+4, x+6, and x+8. Thus, the largest number is x+8.
- As per the arithmetic mean formula, the sum of numbers/5 = 20. Solving it for the numbers listed above:
- x+x+2+x+4+x+6+x+8/5= 20 or 5x +20/5= 20
- To isolate x, take the denomiator of 5 to RHS: 5x+20 = 100 (20*5)
- Further isolate x by subtracting 20 from 100 in the RHS: 5x = 80 (100-20)
- Finally, solve for x: x = 16 (80/5)
- If x =16, then the largest number is x+8: 16+8 = 24
Q12. The average of 6 numbers is 15. If one number is 12 and another number is 18, what is the average of the remaining numbers?
Options:
a) 14.5
b) 16.5
c) 15
d) 13
Answer: c) 15
Solution Explained
Here’s how to solve it:
- The sum of 6 numbers: 6*15 =90
- If two numbers 12 and 18 removed, then the sum of the remaining four numbers will be 90 - (12+18) = 60
- If we remove 2 numbers from 6, we’re left with four numbers. The average of the remaining numbers is the sum of the remaining numbers divided by 4: 60/4 =15
- Therefore, the average of remaining numbers is 15.
Q13. The average of 9 consecutive odd numbers is 25. What is the smallest number in the sequence?
Options:
a) 13
b) 17
c) 19
d) 21
Answer: b) 17
Solution Explained
Here’s how to solve it:
- Let’s consider the smallest odd number in the set to be x.
- Since consecutive odd numbers differ by 2, the other numbers in the series in ascending order are x+2, x+4, x+6, x+8, x+10, x+12, x+14, x+16. The smallest number is x.
- As per the arithmetic mean formula, the sum of numbers/9 = 25. Solving it for the numbers listed above:
- x+x+2+x+4+x+6+x+8+x+10+x+12+x+14+x+16/9= 25 or 9x+72/9=25
- To isolate x, take the denomiator of 9 to RHS: 9x+72 = 225 (25*9)
- Further isolate x by subtracting 72 from 225 in the RHS: 9x = 153 (225-72)
- Finally, solve for x: x = 17 (153/9)
- The smallest number is thus 17.
Q14. The average of 5 numbers is 20. If one number is added, the average becomes 25. What is the added number?
Options:
a) 30
b) 35
c) 40
d) 50
Answer: d) 50
Solution Explained
- If the average of 5 numbers is 20, then the sum of five numbers is: 5*20 =100
- If one number is added, then the average becomes 25. The sum of 6 numbers is: 6*25 =150
- The added number is the difference between the sum of the 6 numbers and the sum of the 5 numbers: 150-100 =50
- Thus, the added number is 50.
Q15. The average of 21 numbers is 40. If the average of the first 10 numbers is 30 and the average of the last 10 numbers is 50, what is the 11th number?
Options:
a) 40
b) 50
c) 70
d) 60
Answer: a) 40
Solution Explained
Here’s how to solve this:
- 11th number = Sum of all 21 numbers - (sum of first 10 numbers + sum of last 10 numbers)
- If the average of 21 numbers is 40, then the sum of 21 numbers is 21*40 = 840
- If the average of the first 10 numbers is 30, then the sum of the first 10 numbers: 10*30 = 300
- If the average of the last 10 numbers is 50, then the sum of the last 10 numbers: 10*50 = 500
- Now, solving for the 11th number: 840 - (500+300) = 840 - 800 = 40
- Therefore, the 11th number is 40.
Explore the topic in detail through this comprehensive course on Quantitative Aptitude on Unstop.
While arithmetic mean is a valuable statistical tool, it's crucial to acknowledge its constraints and explore alternative measures for a comprehensive analysis. To solidify your understanding and analytical capabilities, keep practising calculations and interpretations and master this fundamental statistical concept.
Time For A Short Quiz
Frequently Asked Questions
1. What is the arithmetic mean?
The arithmetic mean is a measure of central tendency that represents the average of a set of numbers. It is calculated by adding up all the values in a dataset and dividing by the total number of values.
2. How do you calculate the arithmetic mean?
To calculate the arithmetic mean, add up all the numbers in your dataset and then divide this sum by the total count of numbers. This formula provides a single value that represents the average of the data points.
3. What are some limitations of using arithmetic mean?
Arithmetic mean can be sensitive to extreme values, known as outliers, which may skew the results. It assumes equal weight for all data points, which may not always be appropriate in certain scenarios.
4. When should weighted arithmetic mean be used?
The weighted arithmetic mean is beneficial when different data points carry varying degrees of importance or significance. By assigning weights to each value based on relevance or priority, a more accurate average can be obtained.
5. How is arithmetic mean applied in statistical analysis?
In statistical analysis, the arithmetic mean is commonly used to summarize a dataset's central tendency, providing insights into the overall trend or average value. It serves as a fundamental tool for understanding and interpreting numerical data.’
Suggested Reads:
I’m a reader first and a writer second, constantly diving into the world of content. If I’m not writing or reading, I like watching movies and dreaming of a life by the beach.
Comments
Add commentLogin to continue reading
And access exclusive content, personalized recommendations, and career-boosting opportunities.
Subscribe
to our newsletter










Khushal Bansal 3 weeks ago
YASH KAPOOR 1 month ago
avneesh kaur 1 month ago
Avinash Mishra 1 month ago
Pardha Venkata Sai Patnam 1 month ago
Sanchita roy 1 month ago