Boats And Streams: Formula, Top Aptitude Questions & Answers (MCQs)

13 mins read

Exploring the fascinating world of boats and streams unveils a realm where mathematical concepts merge seamlessly with real-world applications. Let us delve into the principles governing the speed of a boat in still water to decipher how streams influence its motion.

Key Concept in Boats and Streams

In boat and stream problems, the key concepts revolve around the relative speed of the boat in still water and the speed of the stream (current). Here are the basic concepts and key terms:

Speed in Still Water

• The speed of the boat if there were no currents.
• Represented as B (Boat's speed in still water).

Stream (or Current) Speed

• The speed at which the stream flows.
• Represented as S (Stream speed).

Downstream

Downstream refers to the movement of the boat in the direction of the stream.

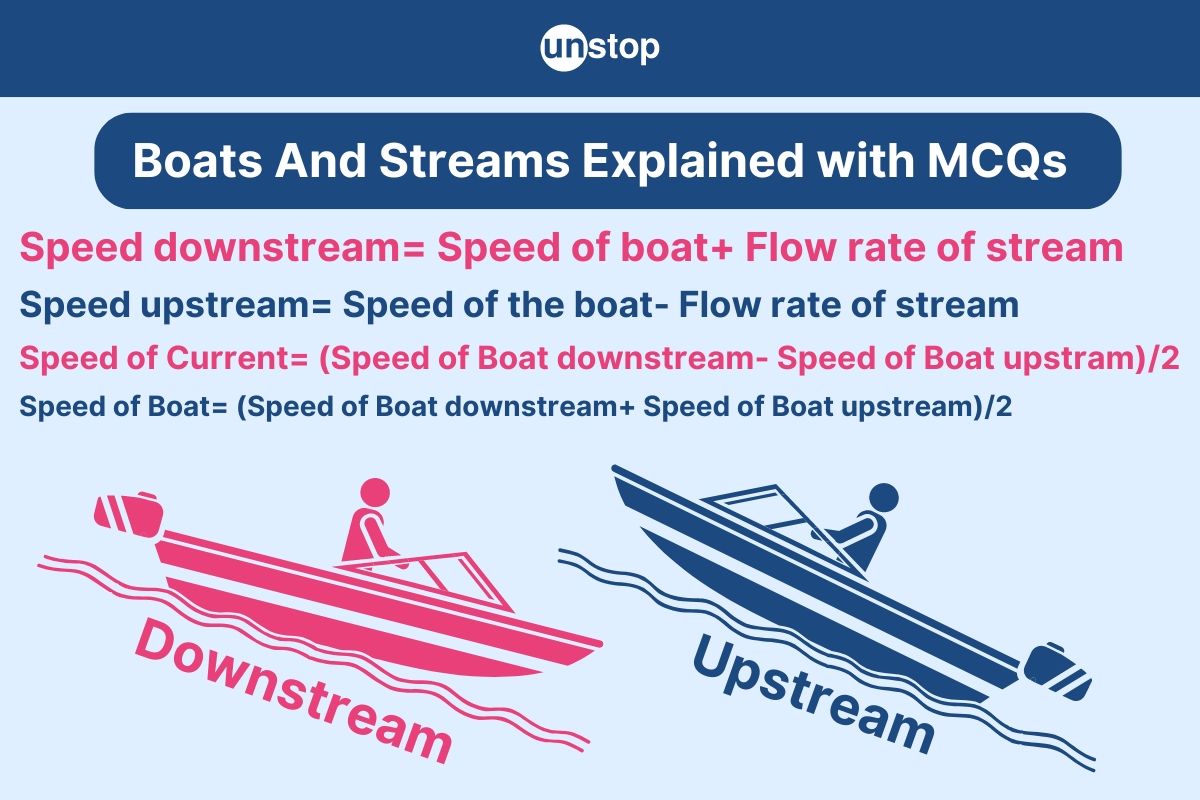
• Downstream speed = Speed of the boat + Speed of the stream
• Downstream speed=B+S

Upstream

Upstream refers to the movement of the boat against the direction of the stream.

• Upstream speed = Speed of the boat − Speed of the stream
• Upstream speed=B−S

Time

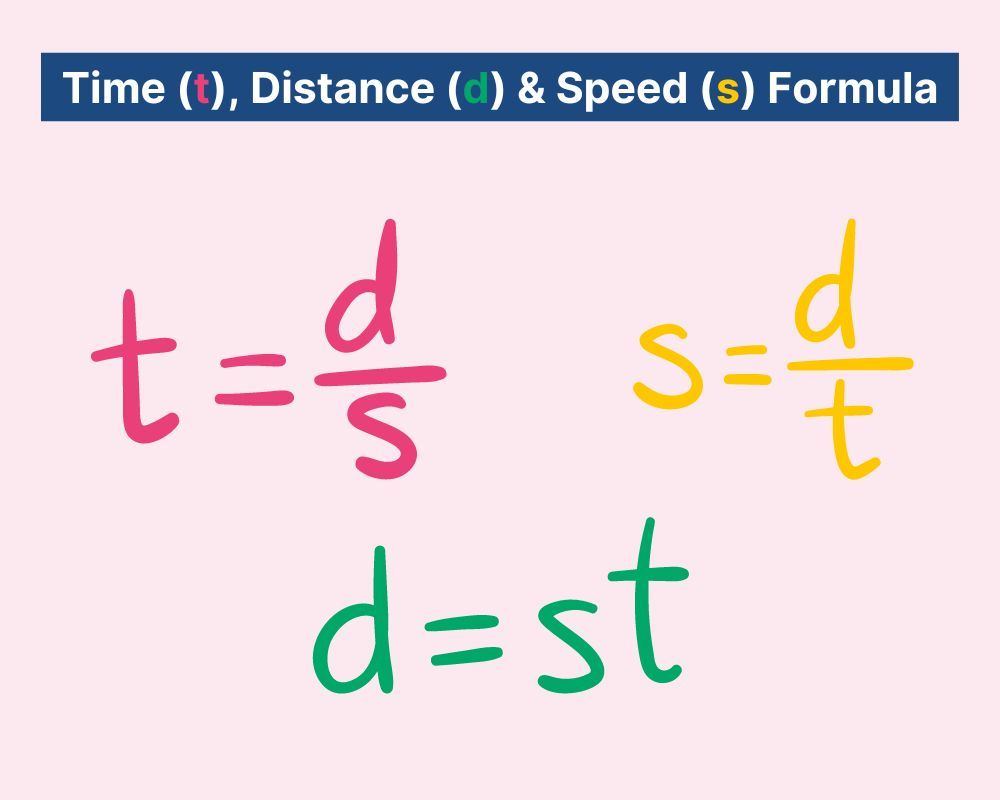
Time is calculated using the formula: Time=Distance/Speed

• For upstream or downstream problems, use respective speeds.

Relative Speed

• Downstream relative speed = Sum of the boat's speed and stream's speed.
• Upstream relative speed = Difference between the boat's speed and the stream's speed.

Let us assume that a boat’s speed in still water is 10 km/h and the stream’s speed is 2 km/h:

• Downstream speed = 10 + 2 = 12 km/h
• Upstream speed = 10 − 2 = 8 km/h

These concepts help solve questions involving distances, times, and speeds in boat and stream problems.

Importance Of Boats and Streams

Solving boat problems helps improve critical thinking and math skills. It makes people better at solving different kinds of problems. The relevance of boat problems extends to competitive exams and aptitude tests, where such questions are commonly featured.

Click here to consistently enhance and upskill your quantitative aptitude and reasoning prowess.

Formulas Of Boats & Streams

Calculating speed in both upstream and downstream scenarios requires specific formulas in boats and streams:

When calculating speed downstream, use the equation:

Speed downstream= Speed of boat+Flow rate of stream

Conversely, when determining speed upstream, utilize the formula:

Speed upstream=Speed of the boat-Flow rate of stream

To calculate the speed of a boat in still water, use the formula:

Speed of Boat = (Speed of Boat downstream + Speed of Boat upstream) / 2

Finding the speed of the current involves using this formula:

Speed of Current = (Speed of Boat downstream - Speed of Boat upstream) / 2

Distance & Time Formula

To find the distance traveled downstream, multiply the total time taken by the combined speeds of the boat and stream.

Similarly, to calculate the time taken in an upstream scenario, divide the total distance by the difference between the boat's speed and the stream's flow rate.

Tips For Solving Boats & Streams Questions

Let's study some of the tips for solving boats and streams questions:

Shortcut for Calculations

A helpful tip for boat and stream problems is to know that the difference between downstream and upstream speeds equals the speed of the current.

Systematic Approach

To solve boat and stream problems, first, understand the basic techniques. Use hints in the question as a starting point. Break down the problem into smaller points for better tackling.

Identifying Patterns

To do well in boat and stream problems, learn about common questions like downstream and upstream situations, relative speed calculations, and time-distance connections.

Time Management

Set time limits for each step of solving a problem to manage time better. Understand the data given before doing calculations. Use shortcuts like cross-multiplication to save time.

Selected Practice Questions With Answers (MCQs)

Let us go through some of the most relevant and up-to-date questions about boats and streams with detailed explanations to arrive at the solution:

Question 1. What is the time taken by a boat to travel 68 km downstream if it can travel at a speed of 18 km/hr in still water? Take the speed of the stream as 5 km/hr.

a) 2 hours 

b) 3 hours 

c) 4 hours 

d) 5 hours

Solution: Speed of the boat in still water (B) = 18 km/hr

Speed of the stream (S) = 5 km/hr

Distance to be travelled downstream (D) = 68 km

The effective speed of the boat downstream is the sum of the boat's speed in still water and the speed of the stream.

Effective Speed Downstream = Speed of Boat + Speed of Stream = 18 km/hr + 5 km/hr = 23 km/hr

Time = Distance / Speed

Time taken to travel downstream = Distance Downstream / Effective Speed Downstream = 68 km / 23 km/hr= 2.957 hours (approx)

Since the time is not in whole hours, we need to convert the fraction of an hour into minutes. (0.957 hours * 60 minutes/hour= 57.42 minutes (approx)

The boat takes approximately 2 hours and 57 minutes to travel 68 km downstream, which is approx. 3 hours.

Question 2. What is the speed of a man against the current if his speed with the current is 18 km/hr? Take the speed of the current as 3.5 km/hr.

a) 14.5 km/hr

b) 15.5 km/hr

c) 16.5 km/hr

d) 17.5 km/hr

Solution: The speed of the man with the current = 18 km/hr

Speed of the current = 3.5 km/hr

We need to find the speed of the man against the current.

The effective speed of the man with the current is the sum of his speed and the speed of the current. 

Effective Speed with the Current=Speed of the Man+Speed of the Current =18 km/hr+3.5 km/hr=21.5km/hr

The effective speed of the man against the current is the difference between his speed and the speed of the current. 

Effective Speed against the Current=Speed of the Man−Speed of the Current =18 km/hr−3.5 km/hr=14.5km/hr

So, the speed of the man against the current is 14.5 km/hr.

Question 3. A motorboat can travel 15 km/hr in still water. If it travels 12 km downstream in 1 hour, what is the speed of the stream?

a) 3 km/hr

b) 4 km/hr

c) 5 km/hr

d) 6 km/hr

Solution: Speed of the motorboat in still water (B) = 15 km/hr

Distance travelled downstream (D) = 12 km

Time taken for downstream journey = 1 hour

Calculate the speed downstream using the formula: Speed downstream=Distance/Time.

Speed downstream=12 km/1 hour=12 km/hr

Using the concept that the speed downstream is equal to the speed of the boat in still water minus the speed of the stream:

Speed downstream=B−S

where S is the speed of the stream.

Substitute the known values into the equation:

12=15−S

Solve for S:

S=15−12

S=3

So, the speed of the stream is 3 km/hr.

Substituting S=3 back into the equation to ensure it satisfies the given conditions:

Speed downstream=Speed of the boat in still water−Speed of stream

12=15−3=12

Question 4. A rowboat can travel 8 km/hr in still water. If it takes the rowboat 2 hours to row 12 km upstream, what is the speed of the stream?

a) 2 km/hr

b) 3 km/hr

c) 4 km/hr

d) 5 km/hr

Solution: Speed of the rowboat in still water (B) = 8 km/hr

Distance travelled upstream (D) = 12 km

Time taken for the upstream journey = 2 hours

Calculate the speed upstream using the formula: Speed upstream=Distance/Time.

Speed upstream=12 km/2 hours=6 km/hr

Using the concept that the speed upstream is equal to the speed of the boat in still water minus the speed of the stream:

Speed upstream=B−S, where S is the speed of the stream.

Substitute the known values into the equation:

6=8−S

S=8−6

S=2

Therefore, the speed of the stream is indeed 2 km/hr.

Question 5. A canoe can travel 12 km/hr in still water. If it takes the canoe 3 hours to paddle 24 km downstream, what is the speed of the stream?

A) 2 km/hr

B) 3 km/hr

C) 4 km/hr

D) 5 km/hr

Solution: Speed of the canoe in still water (B) = 12 km/hr

Distance travelled downstream (D) = 24 km

Time taken for the downstream journey = 3 hours

We need to find the speed of the stream (S).

Speed downstream = Distance / Time = 24 km / 3 hours = 8 km/hr

Speed downstream=Speed of the canoe in still water−Speed of stream

8=12−S

S=12−8 

S=4

Substitute the value of S back into the equation to ensure it satisfies the given conditions: 

Speed downstream=Speed of the canoe in still water−Speed of stream

8=12−4 

8=8

Since the equation holds true, our solution is correct.

Therefore, the speed of the stream is 4 km/hr. 

Question 6. A motorboat can travel at a speed of 20 km/hr in still water. It takes the motorboat 2 hours to travel 30 km upstream. What is the speed of the stream?

a) 5 km/hr

b) 7.5 km/hr

c) 10 km/hr

d) 12.5 km/hr

Solution: Speed of the motorboat in still water (B) = 20 km/hr

Distance travelled upstream (D) = 30 km

Time taken for the upstream journey = 2 hours

We need to find the speed of the stream (S).

Speed upstream = Distance / Time = 30 km / 2 hours = 15 km/hr

Speed upstream is equal to the speed of the boat in still water minus the speed of the stream 

15=20−S

S=20−15

S=5

Therefore, the speed of the stream is 5 km/hr. 

Question 7. A boat travels 45 km upstream and 63 km downstream in a certain time. If the speed of the stream is 3 km/hour, what is the speed of the boat in still water?

a) 15 km/hour

b) 18 km/hour

c) 20 km/hour

d) 24 km/hour

Solution: To solve this question, let x km/hr be the speed of the boat in still water.

The boat's relative upstream speed is (x−3) km/hour, and its relative downstream speed is (x+3) km/hour.

According to the given information:

Time taken to travel upstream = Time taken to travel downstream

Distance traveled upstream = 45 km

Distance traveled downstream = 63 km

Using the formula Time = Distance / Speed, we can set up the following equation based on time, distance, and speed relationships:

For the upstream journey:

45/x−3

For the downstream journey:

63/x+3

Since the times are equal, we can set these expressions equal to each other:

45/x−3​=63/x+3

Let us find the value of x, which represents the speed of the boat in still water: 

45/x−3​=63/x+3

45(x+3)=63(x−3)

45x+135=63x−189

135+189=63x−45x

324=18x

18x=324​

x=324/18

x=18

Therefore, the speed of the boat in still water is 18 km/hour. 

Question 8. A boat travels from point A to point B downstream in 4 hours. The return journey from point B to point A upstream takes 9 hours. Take the speed of the stream as 2 km/hour. Then, what is the speed of the boat in still water?

a) 6 km/hour

b) 8 km/hour

c) 10 km/hour

d) 5.2 km/hour

Solution: Speed of the stream = 2 km/hour

Time taken downstream (from A to B) = 4 hours

Time taken upstream (from B to A) = 9 hours

Let's denote the speed of the boat in still water as x km/hour and the distance between point A and point B as d km.

When the boat travels downstream, its effective speed is x+2 km/hour, and when it travels upstream, its effective speed is x−2 km/hour.

We have the following equations:

For the downstream journey:

d/x+2​=4

For the upstream journey:

d/x−2​=9

We need to solve this system of equations to find the value of x.

Let's start with equation 1:

d/x+2​=4

d=4(x+2)

Now, let's work with equation 2:

d/x−2​=9

d=9(x−2)

Since both expressions are equal to d, we can set them equal to each other:

4(x+2)=9(x−2)

4x+8=9x−18

8+18=9x−4x

26=5x

x=26/5​

x=5.2

So, the speed of the boat in still water is 5.2 km/hour.

Question 9. A boat takes 6 hours to cover a certain distance downstream and takes 9 hours to cover the same distance upstream. Take the speed of the stream as 4 km/hour. Then what is the speed of the boat in still water?

a) 8 km/hour

b) 10 km/hour

c) 20 km/hour

d) 14 km/hour

Solution: Let's denote the speed of the boat in still water as x km/hour.

The distance between the points downstream and upstream is d km

Speed of the stream = 4 km/hour

Time taken downstream = 6 hours

Time taken upstream = 9 hours

When the boat travels downstream, its effective speed is x+4 km/hour, and when it travels upstream, its effective speed is x−4 km/hour.

We have the following equations:

For the downstream journey:

d/x+4​=6

For the upstream journey:

d/x−4​=9

We need to solve this system of equations to find the value of x.

Equation 1:

d/x+4​=6

d=6(x+4)

Equation 2:

d/x−4​=9

d=9(x−4)

Since both expressions are equal to d, we can set them equal to each other:

6(x+4)=9(x−4)

6x+24=9x−36

24+36=9x−6x

60=3x

x=60​/3=20

So, the speed of the boat in still water is 20 km/hour.

Question 10. A boat can travel 54 km downstream in 3 hours. Take the speed of the stream as 3 km/hour. Then what is the speed of the boat in still water?

a) 15 km/hour

b) 18 km/hour

c) 20 km/hour

d) 24 km/hour

Solution: To solve this problem, we can use the formula for the speed of the boat in still water,

Speed of boat in still water= Distance travelled downstream/ Time taken−Speed of the stream

Given:

Distance traveled downstream = 54 km

Time taken = 3 hours

Speed of the stream = 3 km/hour

Using these values in the formula:

Speed of boat in still water=54/3−3=18−3=15 km/hour

So, the speed of the boat in still water is 15 km/hour. 

Conclusion

Studying boat problems, basic concepts, formulas, tips, questions, and quizzes helps you learn about boats and streams. To solve aptitude questions well, understand upstream-downstream relationships and key formulas.

Tips to improve problem-solving and sample questions give real-life practice. To get better at boat problems and math questions, practice regularly.

Time For A Short Quiz 

Frequently Asked Questions (FAQs)

1. What is the meaning of still water in boats and streams?

Still water in boats and streams refers to calm and undisturbed water, without any visible movement or ripples, with its speed at 0 (zero).

2. What is the formula for boats and streams?

The formula for calculating the speed of a boat in still water when moving upstream or downstream in a stream is as follows:

Let the speed of the boat in still water be B km/hr, the stream speed be S km/hr, and the speed of the boat upstream or downstream be U km/hr.

• When moving upstream: Speed of boat in still water - Speed of stream = Speed of boat upstream (B - S) = U

• When moving downstream: Speed of boat in still water + Speed of stream = Speed of boat downstream (B + S) = D

3. What are some key tips for efficiently solving boat and stream problems?

To tackle boat and stream problems successfully, it is recommended to practice regularly, understand the basic concepts thoroughly, visualize scenarios, break down complex questions into simpler parts, and utilize formulas effectively.

4. What is the theory of boat and stream?

The theory of boat and stream involves understanding the relationship between the speed of a boat or swimmer in still water and the speed of the stream or current. By applying formulas related to relative speed, distance, and time, individuals can accurately calculate how long it will take for a boat to travel a certain distance in different conditions.

5. How do you calculate a boat in still water?

To calculate the speed of a boat in still water, you need to consider the boat's velocity without any external factors affecting its movement. This can be determined by measuring the time it takes for the boat to cover a certain distance when there is no current or wind influencing its speed. By dividing the distance travelled by the time taken, you can calculate the boat's speed in still water.

Suggested readings:

• Clock Questions: Selected Question & Answer (Aptitude) Explained 
• Calendar Questions- Selected Aptitude Questions & Answers
• Pipes And Cisterns | Top Selected Question With Answer & Formula
• Problems On Age - Mastering Best Aptitude Questions & Solutions
• Letter & Symbol Series Explained- Top Selected Question & Answer