Aptitude
Table of content:
- Step 1: The Groundwork
- Step 2: The Three Sections
- Step 3: Practice Makes Improvement
- The Secret Ingredient
Table of content:
- Understanding Basics Of Number Series
- Types Of Number Series Reasoning Questions
- Addition & Multiplication In Number Series
- Square & Cube-Based Number Series Patterns
- Missing Terms & Arranging Sequences
- Tricks & Tips For Solving Number Series
- Selected Sample Questions With Detailed Solutions
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Terminologies Used in Blood Relation Questions
- What is a Family Tree?
- Types Of Blood Relation Questions
- Verbal Reasoning in Blood Relation
- Tips For Solving Blood Relation Questions
- Common Pitfalls while Solving Questions
- Selected Questions (MCQs) For Practice With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Letter Series In Logical Reasoning
- Types Of Letter Series Patterns
- Tips For Solving Letter Series Reasoning
- Best Practice Question Samples With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basics of Problems On Age-Related Questions
- Formula to Solve Problems on Ages-Related Questions
- Types of Age-Related Questions & Examples
- Tips & Tricks for Solving Ages Problems
- Best MCQs on Problems on ages with solved answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Definition of Calendar
- Understanding Days of the Week
- Understanding the Concept of Leap Year
- Concept of Odd Days in a Century
- Tips For Calendar Reasoning Questions
- Top 10 Calendar Questions & Answers For Practice (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basic Concepts of Clock
- Structure of a Clock
- Angle Equilavalence in Clock
- Tips For Solving Clock Questions
- Selected Clock Questions & Answers (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding The Concept Of Direction Sense
- Tips For Effective Problem-Solving In Direction Sense
- Practical Test Practice Questions And Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Dice Reasoning
- Dice Numbers In Dice Reasoning
- Classification Of Dice
- Constructed Vs Deconstructed Dice
- Tricks & Tips For Solving Dice Problems
- Practice MCQs With Detailed Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Alphanumeric Series Defined
- Alphanumeric Series In Reasoning Tests
- Tips & Strategies For Solving Alphanumeric Series
- Practice Sample Questions With Detailed Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept Of Mirror Image Reasoning Explained
- Important Terms In Mirror Image Reasoning
- Types Of Mirror Images
- Identifying Correct Mirror Image
- Finding Clock's Mirror Image
- Tips To Solve Mirror Images
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept & Overview Of Input-Output
- Input-Output In Competitive Exams
- Types Of Input-Output Problems
- Strategies, Tips & Tricks For Solving Reasoning Questions
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Finding The Odd One Out
- Tricks And Tips
- Strategies For Finding The Odd One Out
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding Key Concepts
- Exploring Different Ranking Types
- Formula And Application Of Order And Ranking
- Tips For Solving Order & Ranking
- Selected Practice Questions And Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Importance Of Pipes & Cistern Aptitude
- Key Terminologies used in Pipes and Cisterns
- Pipes and Cisterns Formula with Examples
- Pipes and Cisterns Shortcut Tricks
- Tips For Solving Pipes & Cistern Problems
- Selected Questions & Answers For Practice (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Key Concept in Boats and Streams
- Formulas Of Boats & Streams
- Distance & Time Formula
- Tips For Solving Boats & Streams Questions
- Selected Practice Questions With Answers (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Concept of Mixture and Alligation
- Types Of Alligation Questions
- Formula for Solving Mixture & Alligation
- Tips For Solving Mixture And Alligation
- Selected Questions With Answers For Practice
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Time & Work Concepts
- Time And Work Formulas
- Tips For Solving Time And Work Questions
- Time And Work Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is Harmonic Progression(HP)?
- Formula to find the nth Term in Harmonic Progression
- Formula to find the Sum of the nth Term in HP
- What is Harmonic Mean?
- Harmonic Progression Solved Best MCQs
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is Mensuration in Maths?
- What are 2D figures in Mensuration?
- What are 3D figures in Mensuartion?
- Basic Terminologies In Mensuration
- Basic 2D Formulas in Mensuration
- Basic 3D Formulas in Mensuration
- 2D vs 3D in Mensuration
- Solved Questions With Solutions (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Relationship Between Time, Speed And Distance
- Conversion Units Time, Speed And Distance
- Average & Relative Speed: Two Trains Moving in the same or opposite direction
- Solved MCQs on Time, Speed And Distance
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basics Of Simplification
- BODMAS Rule in Simplification Explained
- Simplification & Approximation Relation
- Key Terms in Simplification
- Examples Of Simplification Techniques
- Simplification Questions With Solved Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Height And Distance Important Terms
- Right Angled Triangle In Trigonometry
- Trigonometric Ratios
- Solved Examples For Better Understanding
- Height And Distance Applications In Trigonometry
- Height And Distance Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Interest Types
- Simple Interest Vs. Compound Interest
- Selected Solved Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Basic Concepts Of Profit And Loss
- Determining Selling Price
- Calculating Discounts
- Formulas For Calculating Profit And Loss
- Examples Of Profit And Loss
- Profit & Loss Questions With Detailed Solution
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Angle Of Elevation
- Key Terms Used In Angle Of Elevation
- Angle of Elevation Formula with Example
- Angle of Elevation vs. Angle of Depression
- Angle of Elevation MCQs with Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining HCF And LCM
- Calculation Methods Of HCF And LCM
- HCF By Prime Factorization Method
- LCM By Prime Factorization Method
- Difference Between HCF And LCM
- HCF & LCM Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- What is fraction and decimal?
- Understanding Decimal Fraction
- Place Value in Decimal Fraction
- Mathematical Operations with Decimal Fraction
- Practice with Solved Examples
- Summary
- Frequently Asked Questions
Table of content:
- All About Decimals
- All About Fractions
- How to Convert a Decimal into Fraction
- Simple vs Recurring Decimals
- Converting Recurring Decimals to Fractions
- Conversion Charts
- Practice Questions (With Solutions)
- Closing Thoughts
- Frequently Asked Questions
Table of content:
- What is Arithmetic Mean?
- Arithmetic Mean Formula- Ungroup Data & Group Data
- Merits of Arithmetic Mean
- Demerits of Arithmetic Mean
- Alternatives to Arithmetic Mean
- What is the Weighted Arithmetic Mean?
- Arithmetic vs. Geometric Mean
- Arithmetic Mean Application in Statistical Analysis
- Arithmetic Mean Practice Questions with Explanation
- Frequently Asked Questions
Table of content:
- What is Geometric Progression?
- Key Properties of Geometric Progression
- General Form Of Geometric Progression
- General Term or the Nth Term of Geometric Progression
- The sum of nth Terms of GP
- Types Of Geometric Progression
- Solved Questions and Answers of GP
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Average in Maths
- Average Formula in Maths
- Differentiating Types of Average
- How to Calculate Average of Negative Numbers?
- Practical Applications of Averages
- Average Questions For Practice
- Frequently Asked Questions
Table of content:
- What is Simple Interest in Maths?
- Simple Interest Formula Explained
- Simple Interest Formula for Years, Months & Days
- Simple Interest Examples & Practice Questions
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Defining Mathematical Ratios
- Understanding Proportions Fundamentals
- Differentiating Ratios from Proportions
- Ratio and Proportion Formulas
- Properties of Ratio and Proportion
- How to Solve Ratio and Proportion Problems
- Ratio and Proportion Problems (With Solutions)
- Summary
- Frequently Asked Questions
Table of content:
- What is Number in Maths?
- Types of Numbers With Example
- Real vs Complex Numbers Explored
- Basic Operations on Numbers
- Practice Questions (With Solutions)
- Frequently Asked Questions
Table of content:
- What is Arithmetic Progression (AP) in Maths?
- Important Terminologies in Arithmetic Progression
- Basic Terms in Arithmetic Progression
- General Form Of Arithmetic Progression Series
- Types Of Arithmetic Progression
- Solved Questions With Explanation (MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Understanding Basic Concept
- Importance Of Train Problems In Aptitude
- Tips To Solve Train Problems
- Selected Practice Questions & Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Definition Of Mode
- Calculating Mode For Ungrouped Data
- Calculating Mode For Grouped Data
- Bimodal, Trimodal & Multimodal Distributions
- Comparing Mean, Median & Mode
- Selected Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Important Concept In Races And Games
- Calculating Time, Speed & Distance
- Importance Of Races And Games Problems
- Sample Practice Questions With Answers
- Conclusion
- Frequently Asked Questions (FAQs)
Table of content:
- Types Of Partnership
- Formula For Partnership Questions
- Tips To Solve Partnership Aptitude Questions
- Selected Partnership Questions (Practice MCQs)
- Conclusion
- Frequently Asked Questions (FAQs)
Mixture and Alligation: Basic Concept, Formulas and Solved MCQs
Understanding how different components combine in a mixture and alligation can be perplexing but essential, especially when it comes to quantitative aptitude tests, be it for placement interviews or competitive exams.
A detailed explanation of the concept and formula with selected questions and answers related to mixture and alligation is provided here in this article.
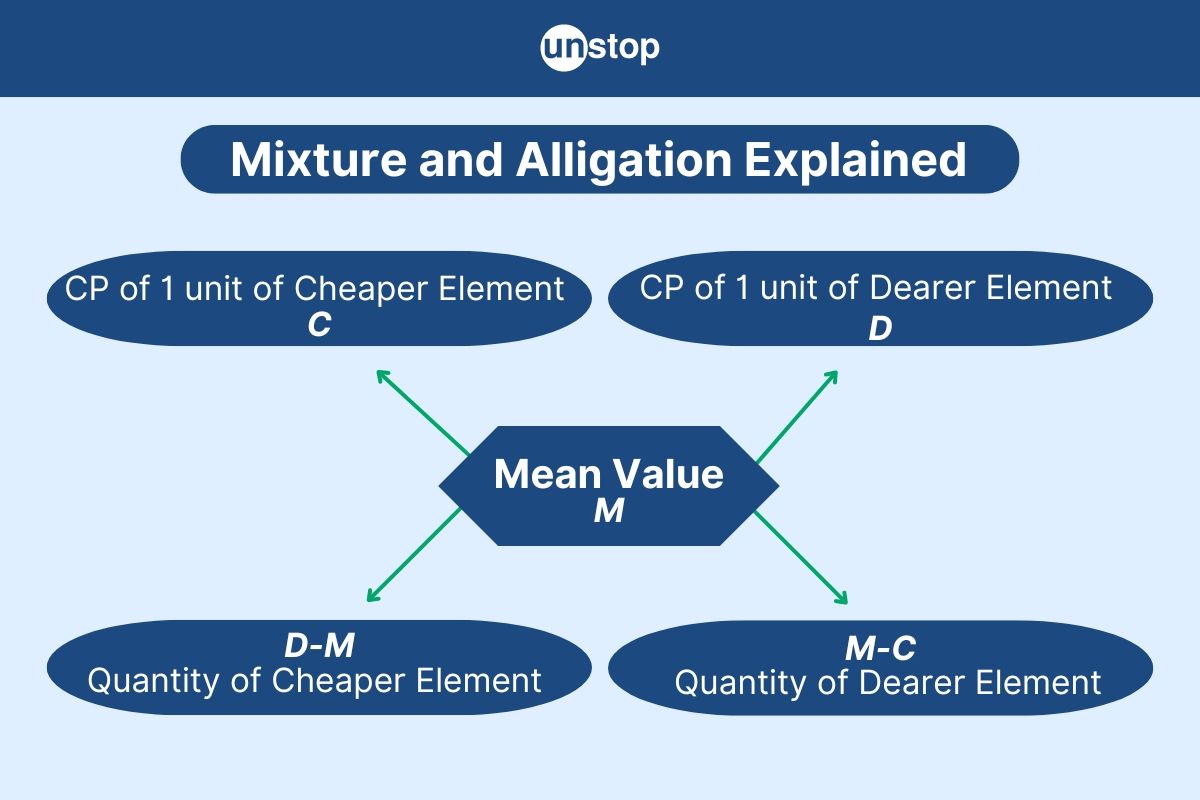
Concept of Mixture and Alligation
Mixture refers to a combination of two or more different substances, while alligation is a method used to solve problems related to mixtures.
Mixture and alligation help in determining the quantities of different components needed to create a mixture with specific properties.
The alligation rule states that if two ingredients are mixed to form a mixture, then the ratio of quantities of the two ingredients is inversely proportional to their prices.
Types Of Alligation Questions
Alligation questions typically fall into two categories:
-
Simple alligation involves finding the ratio in which two or more ingredients must be mixed to obtain a mixture at a specified price.
-
Compound alligation, on the other hand, deals with solutions containing multiple ingredients of different prices.
Formula for Solving Mixture & Alligation
To tackle numerical ability problems effectively, it's essential to understand the important formulas related to mixture and alligation. These formulas can significantly simplify the process, making it quicker and more efficient. Provided below are some of the basic formulas related to mixture and alligation:
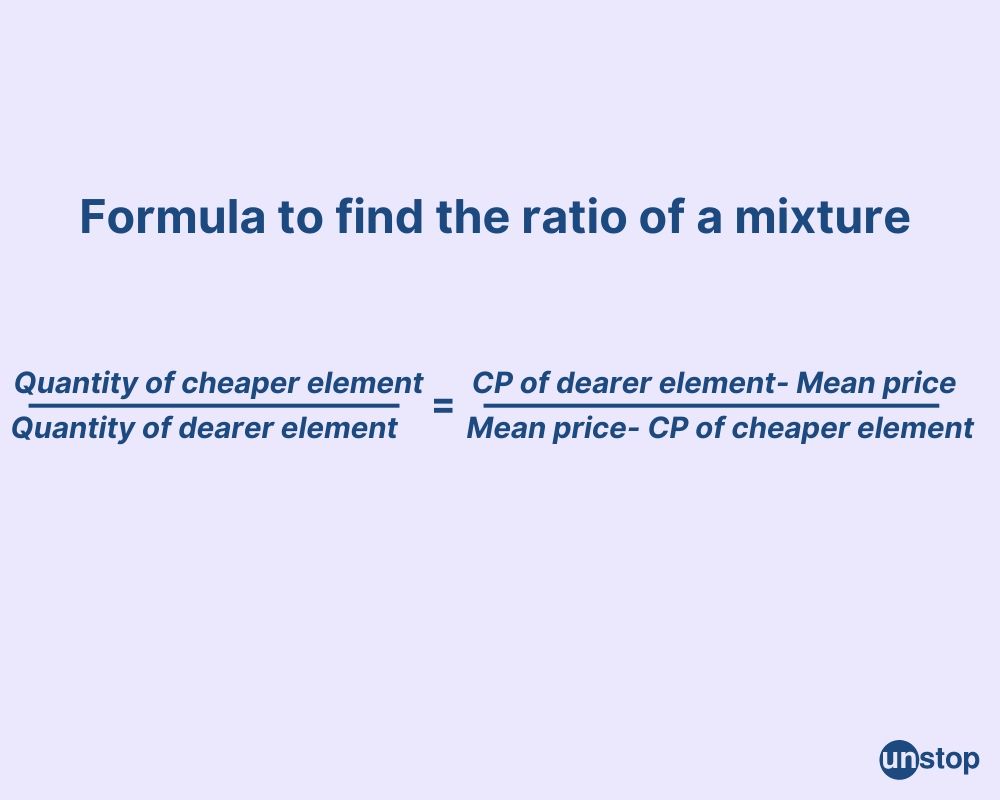
Formula to find the ratio of Mixture
Rule of Alligation Formula
The rule of alligation calculates the ratio for mixing ingredients of different costs to achieve a desired average. It compares the differences between individual prices and the desired price.
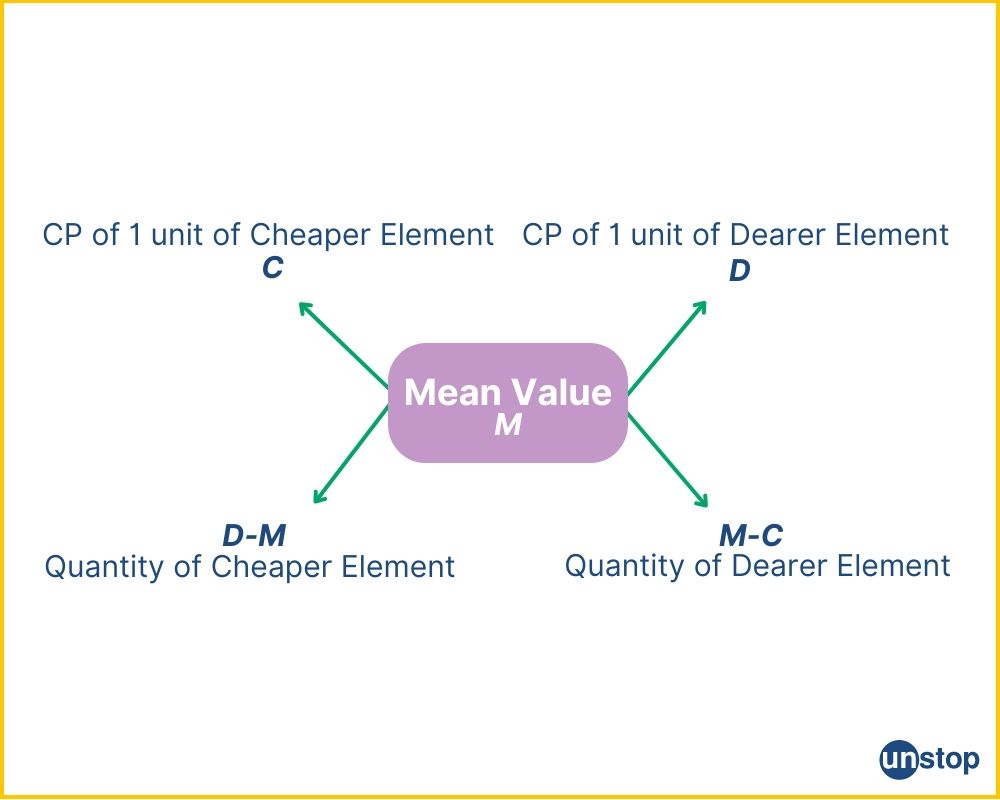
The ratio shows how much of each ingredient is needed.
Tips For Solving Mixture And Alligation
Let us study some of the tips to solve mixture and alligation problems:

Practice Consistently
Consistent practice is crucial for mastering mixture and alligation problems. Regularly solving a variety of questions helps in understanding different scenarios and improves problem-solving skills over time.
Utilize Time-Saving Techniques
Implement time-saving techniques to tackle complex mixture and alligation problems efficiently. One such technique is setting up equations systematically, ensuring accuracy while saving valuable time during examinations or assessments.
Conceptual Clarity
Develop a strong conceptual clarity about the principles of mixture and alligation. Understanding the underlying concepts allows for quicker identification of problem types and application of appropriate solution strategies.
Click here to consistently enhance and upskill your quantitative aptitude and reasoning prowess.
Selected Questions With Answers For Practice
For your practice, we have selected some questions with a detailed explanation of mixture and alligation below:
Question 1. The average salary per head of all workers (Grade A and Grade B) of a company is INR 400. The average salary of 100 Grade-A workers is INR 1000. If the average salary per head of the rest of the Grade-B workers is INR 300, find the total number of workers in the company.
A) 1000
B) 800
C) 500
D) 700
Solution: D)
Explanation:
Total workers = Number of Grade-A workers + Number of Grade-B workers. Let 'x' be the number of Grade-B workers. So, total workers = 100+x
The average salary of all workers = ₹400. Therefore, the total salary for all workers = ₹400 × (100 + x).
Total salary of Grade-A workers = ₹1,000 × 100 = ₹1,00,000.
The average salary of Grade-B workers = ₹300. So, the total salary for Grade-B workers = ₹300 × x.
Combine total salaries:
₹400×(100+x)=₹1,00,000+₹300×x
Solve for x:
₹40,000+₹400x=₹1,00,000+₹300x
₹400x−₹300x=₹1,00,000−₹40,000
₹100x=₹60,000
x=600
Total number of workers = 100 (Grade-A) + 600 (Grade-B) = 700.
Question 2. Two types of sugar are priced at ₹40 per kg and ₹60 per kg. In what ratio should they be mixed to obtain a mixture costing ₹50 per kg?
A) 1:1
B) 2:3
C) 1:2
D) 2:1
Solution: A) 1:1
Explanation:
Difference between ₹60 (costlier sugar) and ₹50 (desired cost) = ₹10
Difference between ₹50 (desired cost) and ₹40 (cheaper sugar) = ₹10
According to the alligation formula, the ratio is the inverse of the differences: ₹10 (from ₹60): ₹10 (from ₹40) = 1:1.
Question 3. A tank contains 500 liters of wine. 50 litres of wine is taken out of it and replaced by water. The process is repeated again. Find the proportion of water and wine in the resulting mixture.
A) 1 : 4
B) 41:50
C) 19:81
D) 8 :19
Solution: C) 19:81
Explanation:
The initial amount of wine is 500 litres, of which 50 litres (i.e., 1/10th) is replaced with water. The remaining 9/10th is wine. When the step is repeated, 9/10th of the remaining mixture is wine. So, the amount of wine left = 500 X 9/10 X 9/10 = 405 liters.
Hence, the amount of water = 95 liters. The ratio of water and wine = 19:81.
Question 4. A man purchased a table and a chair for INR 2000. He sold the table at a profit of 20% and the chair at a profit of 40%. In this way, his total profit was 25%. Find the cost price (in INR) of the table.
A) 1500
B) 900
C) 1000
D) 800
Solution: A) 1500
Explanation:
As per the question, the total cost of the table and chair = ₹2000, the profit on the table = 20%, the profit on the chair = 40% and the overall profit = 25%.
Let the cost of the table be T and the chair be C.
So, T+C=2000.
Using alligation:
- Difference between chair’s profit (40%) and overall (25%) = 15%.
- Difference between overall (25%) and table’s profit (20%) = 5%.
Thus, the ratio of the cost of the table to the chair is 15:5=3:1.
Since the ratio is 3:13:13:1, the total cost is split into 4 parts (3 parts for the table, 1 part for the chair)
Now, the cost of the table T=3/4×2000=₹1500.
Question 5. A dishonest shopkeeper purchased milk at INR 100 per litre and mixed 10 litres of water in it. By selling the mixture at the rate of INR 100 per litre, he earns a profit of 25%. The quantity of the amount of the mixture that he had was:
A) 50 litres
B) 40 litres
C) 25 litres
D) 60 litres
Solution: A) 50 litres
Explanation:
As per the question, the shopkeeper buys milk at ₹100 per litre and adds 10 litres of water to the milk. He sells the entire mixture at ₹100 per litre and makes a profit of 25%.
Let the quantity of pure milk purchased be x litres.
C.P. of pure milk=100×x=100x
Since the shopkeeper earns a profit of 25%, the Selling Price (S.P.) must be 25% higher than the cost price.
S.P.=C.P.+25%of C.P.=100x+0.25×100x=125x
The shopkeeper added 10 litres of water to the pure milk. So, the total mixture quantity = x litres of milk+10 litres of water.
The selling price per litre = ₹100, and he sells the entire mixture. Thus:
125x=100×(x+10)
125x=100x+1000
125x−100x=1000
x=1000/25=40
Therefore, the total quantity of the mixture is x+10=40+10=50 litres.
Question 6. A milkman stole milk from a can that contained 50% milk, and he replaced what he had stolen with milk having 20% milk. The bottle then contained only 25% milk. How much of the bottle did he steal?
A) 80%
B) 83.33%
C) 85.71%
D) 88.88%
Solution: B) 83.33%
Explanation:
As per the question, initially, the bottle contained 50% milk. The milkman stole some milk and replaced it with another mixture that had 20% milk. After mixing, the final percentage of milk in the bottle became 25%.
Let 'x' be the fraction of the bottle the milkman stole and replaced.
The fraction of milk left after the milkman stole and replaced is (1−x) of the initial 50% milk. The fraction of milk added is x, but it contains only 20% milk.
The final percentage of milk is 25% after the theft and replacement, so the total milk percentage can be written as:
(1−x)×50%+x×20%=25%
(1−x)×50+x×20=25
50−50x+20x=25
50−30x=25
50−25=30x
25=30x
x=25/30=5/6
The milkman stole 5/6 of the bottle, which is approximately 83.33% of the total content.
Question 7. A grocer has two types of wheat. One type costs ₹25 per kg, and the other costs ₹40 per kg. He wants to mix them to get a mixture costing ₹30 per kg. In what ratio should the two types of wheat be mixed?
A) 2:3
B) 3:1
C) 5:3
D) 2:1
Solution: D) 2:1
Explanation:
Difference between ₹40 (costlier wheat) and ₹30 (desired cost) = ₹10
Difference between ₹30 (desired cost) and ₹25 (cheaper wheat) = ₹5
Using alliagation rule, the ratio of the two types of wheat is the inverse of these differences:
Ratio=10:5=2:1.
Question 8. A 10 percent gain is made by selling the mixture of two types of milk at INR 48 per kg. If the type costing INR 61 per kg was mixed with 100 kg of the other, how many kilograms of the former was mixed?
A) 38 kg
B) 30.5 kg
C) 19 kg
D) Cannot be determined
Solution: D) Cannot be determined
Explanation:
We cannot determine the answer to this question as we do not know the price per kg of the other type of milk. Hence, we cannot find the ratio of mixing that would be required in order to move further in this question.
Question 9. A person purchased a pen and a pencil for INR 15. He sold the pen at a profit of 20% and the pencil at a profit of 30%. If his total profit was 24%, find the cost price of the pen.
A) INR 10.50
B) INR 12
C) INR 9
D) INR 10
Solution: C) INR 9
Explanation: As per the question, the total cost of the pen and pencil is INR 15. The profit on the pen is 20%, the profit on the pencil is 30%, and the overall profit is 24%.
Let the cost price of the pen be x, and the cost price of the pencil be 15−x.
Total Profit=24%×15=24/100×15=3.6
The profit from the pen is 20% of x
Profit from the pen=0.20×x=0.2x
The profit from the pencil is 30% of 15−x
Profit from the pencil=0.30×(15−x)=4.5−0.3x
The sum of the individual profits must equal the total profit:
0.2x+(4.5−0.3x)=3.6
0.2x−0.3x+4.5=3.6
−0.1x+4.5=3.6
Subtract 4.5 from both sides:
−0.1x=3.6−4.5=−0.9
x=−0.9/−0.1=9
The pen cost INR 9.
Question 10. A shopkeeper has two types of rice. One type costs ₹30 per kg, and the other costs ₹50 per kg. He wants to create a mixture that costs ₹40 per kg. In what ratio should he mix the two types of rice?
A) 2:3
B) 2:3
C) 1:1
D) 2:4
Solution: D) 1:1
Explanation:
Calculate the differences:
Difference between ₹50 (costlier rice) and ₹40 (desired cost) = ₹10
Difference between ₹40 (desired cost) and ₹30 (cheaper rice) = ₹10
Apply the alligation rule:
The ratio of the two types of rice is the inverse of these differences:
Ratio=10:10=1:1
Conclusion
Understanding mixture and alligation, mastering key techniques, and practicing with aptitude quiz challenges are vital steps in enhancing problem-solving skills. The importance of these skills cannot be overstated, as they play a crucial role in various fields requiring quantitative analysis.
Take the opportunity to delve deeper into the realm of mixture and alligation by practicing regularly, seeking additional resources, and expanding your knowledge base. Engage with challenging problems, participate in online classes, and collaborate with peers to sharpen your skills further.
Time For A Short Quiz
Frequently Asked Questions (FAQs)
1. What is mixture and alligation method?
The mixture and alligation method is a mathematical technique used to solve problems related to the mixing of different ingredients at various concentrations to obtain a desired overall mixture. By using the principles of alligation, which involves finding the weighted average of the concentrations of different components, one can easily calculate the quantities needed to achieve a specific mixture.
2. What is an example of a mixture alligation?
An example of a mixture alligation is when different concentrations of two substances are mixed together to create a desired concentration. For instance, if you have a 20% solution of sugar and a 50% solution of sugar, you can use alligation to calculate how much of each solution to mix in order to achieve a final solution with a specific sugar concentration, such as 30%.
3. What is the logic of mixture and alligation?
The logic of mixture and alligation revolves around finding the proportions in which two or more ingredients with different strengths or concentrations should be mixed to obtain a desired strength or concentration in the final mixture.
4. What is the rule of alligation formula?
The rule of alligation formula involves finding the weighted average of two or more components with different concentrations to achieve a desired overall concentration.
5. Can practice with aptitude quiz challenges improve proficiency in mixture and allegation?
Yes, practicing with aptitude quiz challenges that include mixture and alligation questions can enhance proficiency by providing exposure to various problem scenarios. Regular practice helps identify patterns, improve calculation speed, boost confidence levels, and refine problem-solving strategies for better performance in assessments.
Suggested reads:
Boats And Streams: Formula, Top Question With Solution (Aptitude)
Clock Questions: Selected Question & Answer (Aptitude) Explained
Calendar Questions- Selected Aptitude Questions & Answers
Pipes And Cisterns | Top Selected Question With Answer & Formula
Problems On Age - Mastering Best Aptitude Questions & Solutions
Instinctively, I fall for nature, music, humour, reading, writing, listening, travelling, observing, learning, unlearning, friendship, exercise, etc., all these from the cradle to the grave- that's ME! It's my irrefutable belief in the uniqueness of all. I'll vehemently defend your right to be your best while I expect the same from you!
Comments
Add commentLogin to continue reading
And access exclusive content, personalized recommendations, and career-boosting opportunities.
Subscribe
to our newsletter







Khushal Bansal 1 week ago
YASH KAPOOR 1 month ago
avneesh kaur 1 month ago
Pardha Venkata Sai Patnam 1 month ago
Hrishant Bharati 1 month ago
Aryan Anand Chanduka 1 month ago