Average in Maths: Definition, Symbol, Formula & Solved MCQs

13 mins read

Struggling with the average in maths? Don't fret! By the end of this read, you'll be equipped with the knowledge and confidence needed to tackle average-related problems with ease.

Average in Maths

Average Definition: Average in maths refers to the central value of a dataset. It represents the typical value around which the data points tend to cluster.

To calculate the average, you sum up all the data points in the dataset and then divide by the total number of data points. This ratio gives you the average value. In statistics, the average is commonly referred to as the mean.

Understanding averages is crucial in real-life scenarios. For example, when analyzing exam results for a class, calculating the average score provides insight into how well students performed collectively. It simplifies complex data sets into a single representative value.

Average Symbols: Symbols like x̄ and ‘μ’ are utilized to represent average in maths. For instance, if we have numbers 10, 15, and 20, their average would be:

x̄ = (10 + 15 + 20) / 3.

Why use symbols? Symbols hold immense importance in mathematical representations as they provide a concise and standardized way to convey complex information. By using symbols like x̄, mathematicians can easily perform calculations and analyze data sets efficiently.

Average Formula in Maths

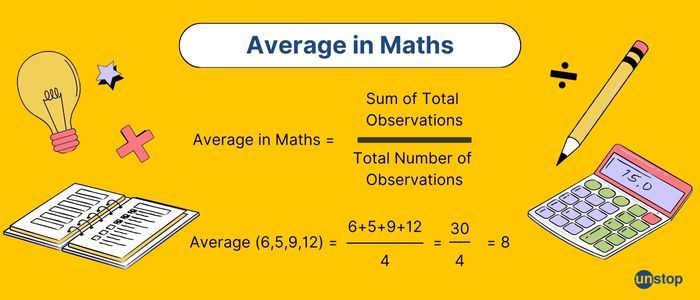
To calculate the average, you need to sum up all the values in a list and then divide by the total number of values.

The formula for average is Average = Sum of Values/ Number of values.

Example: For instance, if you have a list of ages {25, 30, 35, 40}, to find the average age:
(25 + 30 + 35 + 40) / 4 = 32.5.

How to Calculate Average?

Here’s a step-by-step approach to calculate average in maths:

• Step 1: To calculate the average of a set of numbers, begin by adding all the numbers together to find the total sum. This step is crucial as it forms the basis for determining the average accurately.
• Step 2: Next, count the total number of observations in your dataset. This involves identifying how many numbers you have in your list. The accuracy of the average calculation depends on correctly identifying this factor.
• Step 3: Once you have obtained the sum of all numbers and determined the total observations, measure by dividing the sum by the number of observations. This division yields the average value of the dataset, providing a representative figure for the overall data set.

Differentiating Types of Average

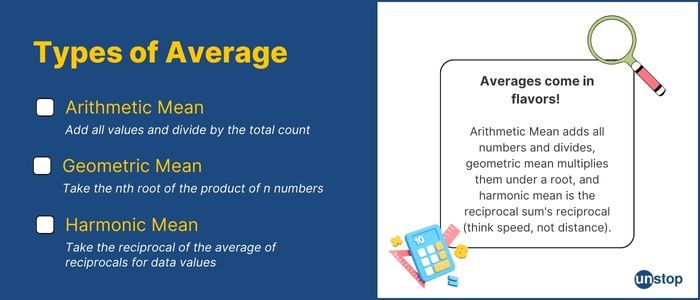
Let’s take a look at the most common types of averages in Maths:

Arithmetic Mean

Arithmetic Mean, a common type of average, is calculated by adding all values and dividing by the total count. It is straightforward to calculate the Arithmetic Mean: simply add up all the values and divide by how many there are.

Geometric Mean

Geometric Mean, another type of average in Maths, involves taking the nth root of the product of n numbers. To find the Geometric Mean, multiply all values together and then take the nth root, where n represents the number of values.

Harmonic Mean

Harmonic Mean, a part of averages, is found by taking the reciprocal of the average of reciprocals for data values. When calculating the Harmonic Mean, first find the reciprocals of all values, then find their average, and finally take the reciprocal of this result.

How to Calculate Average of Negative Numbers?

When dealing with negative numbers, the process of calculating averages remains consistent. The presence of negative values does not complicate the method; it merely adds a variation to the data set.

Thus, negative numbers do not modify the fundamental formula for determining averages. The formula still involves adding all numbers together, and dividing by the total count.

Examples

• For instance, when finding the average of -5, 3, and -7, you would add them (-5 + 3 + -7 = -9) and divide the total by 3 (total count), resulting in an average of -3.
• Similarly, if you have -10, -2, and -4, adding them (-10 + -2 + -4 = -16) and dividing by 3 gives an average of -5.33.

Practical Applications of Averages

Finance

Averages play a crucial role in finance, helping individuals analyze investment returns over years. By calculating the central value of stock prices, investors can make informed decisions.

Sports

In sports, averages are used to evaluate player performance based on observations from games. Coaches rely on average scores to assess player consistency and identify areas for improvement.

Academics

In academics, averages are essential for evaluating student performance in school. Teachers use average grades to understand overall class progress and identify students who may need extra support.

Averages are also utilized in survey data analysis to understand public opinion on various questions. By calculating the average responses, researchers can draw meaningful insights about a group of people.

Average Questions For Practice

Q1. Find the average of 5, 10, and 15.

Options:
a) 30
b) 15
c) 10
d) 5

Answer: c) 10

Solution Explained:

To find the average of a set of numbers, add all the numbers together and then divide by the total count.

• Add: 5 + 10 + 15 = 30
• Divide: 30/3 (sum/total count) = 10
• The average is 10

Q2. If the average of three numbers is 15, and two of the numbers are 10 and 20, what is the third number?

Options:
a) 5
b) 10
c) 25
d) 15

Answer: d) 15

Solution Explained:

Here’s how to solve this:

• Consider the third number as x.
• If the average is 15, then: (x+10+20)/3 = 15 OR (x +30)/3 = 15
• Isolate x by multiplying the denominator 3 with 15 on RHS: x+30 = 45 (15*3)
• Solve for x: x = 45-30 = 15
• Thus, the third number is 15.

Q3. A student scores 60, 70, and 80 marks out of 100 in three subjects. What is the average score?

Options:
a) 60
b) 75
c) 80
d) 70

Answer: d) 70

Solution Explained:

Here’s how to solve this:

• The average formula = sum of all values/total number of values
• The sum of scores: 60+70+80: 210
• Total number of values: 3
• Average: 210/3 = 70
• Thus, the average score is 70.

Q4. The average weight of three boys is 50 kg. If one boy weighs 45 kg and another weighs 55 kg, what is the weight of the third boy?

Options:
a) 40
b) 45
c) 50
d) 60

Answer: c) 50

Solution Explained:

Here’s how to solve this

• Let’s consider the weight of the third boy to be X.
• As per the average formula: (X+45+55)/3 = 50 OR (X+100)/3 = 50
• Isolate X by multiplying 3 from the denominator with the RHS: X+100=150 (50*3)
• Solve for X: X= 150-100=50
• Thus, the weight of the third boy is 50 kg.

Q5. The average of five numbers is 60. If one number is excluded, the average becomes 50. What is the excluded number?

Options:
a) 100
b) 60
c) 70
d) 50

Answer: a) 100

Solution Explained:

Here’s how to solve this:

• Let’s consider the sum of five numbers = x
• Going by the formula for average, x/5 = 60
• Solving for x: x= 300 (60*5)
• Now, let’s consider the excluded number = a
• To calculate the new average, subtract the excluded number from the total sum (x) and reduce the total count by 1. Thus, the new average is: (300-a)/4=50
• Solving for a:
  • 300-a=200 (50*4)
  • a=300-200=100
• Thus, the excluded number is 100.

Q6. The average age of a group of 10 friends is 30 years. If one friend leaves the group and the average age becomes 29 years, how old was the friend who left?

Options:
a) 25
b) 39
c) 35
d) 20

Answer: b) 39 years

Solution Explained:

Here’s how to solve this:

• Let’s consider the sum of the ages of all 10 friends = x
• Going by the formula for average, x/10 = 30
• Solving for x: x= 300 (30*10)
• Now, let’s consider the age of the friend who’s leaving the group = a
• To calculate the new average, subtract the excluded friend’s age from the total sum (x). Also, reduce the total count by 1. Thus, the new average is: (300-a)/9=29
• Solving for a:
  • 300-a=261 (29*9)
  • a=300-261=39
• Thus, the age of the friend leaving the group is 39 years.

Q7. A teacher's average score on five tests is 80. If the average of the first four tests is 78, what was the score of the fifth test?

Options:
a) 76
b) 88
c) 84
d) 80

Answer: b) 88

Solution Explained:

To find the score of the fifth test, we need to subtract the sum of the scores of the four tests from the sum of the scores of the five tests.

• Let’s consider the sum of the scores on the five tests = x
• Using the average formula: x/5= 80. This brings x=400
• Now, let’s consider the score of the fifth test = a
• If we remove the score of the fifth test, the average will be: (400-a)/4=78
• Solving for a:
  • 400-a=312 (78*4)
  • a=400-312=88
• Thus, the score of the fifth test is 88.

Q8. The average age of a family of four is 25 years. If the father's age is included, the average becomes 30 years. How old is the father?

Options:
a) 45
b) 50
c) 40
d) 55

Answer: b) 50

Solution Explained:

To find the father’s age, we need to subtract the sum of the ages of four family members (excluding the father) from the sum of the ages of the five family members (including the father)

• Let’s consider the sum of the ages of the five family members = x
• Using the average formula: x/5= 30. This brings x=150
• Now, let’s consider the age of father = a
• If we remove the age of the father, the average will be: (150-a)/4=25
• Solving for a:
  • 150-a=100 (25*4)
  • a=150-100=50
• Thus, the father’s age is 50 years.

Q9. The average of 10 numbers is 15. If each number is multiplied by 3, what is the new average?

Options:
a) 30
b) 35
c) 40
d) 45

Answer: d) 45

Solution Explained:

Here’s how to solve this:

• If the average of 10 numbers is 15, then using the formula, we can get the sum of the 10 numbers.
  • The sum of 10 numbers/10 = 15
  • The sum of 10 numbers: 15*10= 150
• Now if each number is multiplied by 3, then the overall sum will be multiplied by 3 as well. Thus, the new average will become:
  • 3 (Sum of 10 numbers)/10 = 3*150/10 = 450/10 = 45
• So, the new average is 45.

Q10. The average of five numbers is 40. If each number is multiplied by 2 and then 5 is subtracted from each, what is the new average?

Options:
a) 75
b) 40
c) 45
d) 30

Answer: a) 75

Solution Explained:

Here’s how to solve this:

• Let’s consider the sum of five numbers = x
• As per the formula for average: x/5= 40. Thus, x= 200 (40*5)
• Now, if each number is multiplied by 2, the overall sum will be multiplied by 2 as well.
  • Thus, the new sum: 2x = 2*200 = 400.
• If 5 is subtracted from each number, the overall sum will be subtracted by 25 (5*5).
  • Thus, the revised sum: 400-25 = 375
• Now, the new average is: 375/5 = 75

Q11. The average of six numbers is 18. If each number is increased by 4, what is the new average?

Options:
a) 20
b) 22
c) 24
d) 18

Answer: b) 22

Solution Explained:

Here’s how to solve this:

• Let’s consider the sum of six numbers = x
• Using the average formula, we can solve for x:
  • x/6= 18 OR x = 108 (18*6)
• If each number is increased by 4, the overall sum will increase by 24 (6*4).
  • Thus, the new sum: 108+24= 132
• Now, the new average is: 132/6 = 22

Q12. In a group of seven friends, the average age is 28 years. If the youngest person is removed from the group, the average age becomes 30 years. What was the age of the youngest person?

Options:
a) 24 years
b) 16 years
c) 18 years
d) 22 years

Answer: b) 16 years

Solution Explained:

Here’s how to solve this:

• Let’s consider the sum of the ages of seven friends = x

• Using the average formula, we can solve for x:
  • x/7= 28 OR x = 196 (28*7)
• Let’s consider the age of the youngest person = a
• If a is removed, the sum of the ages of the remaining six friends = 196-a
• The average age of these six friends: (196-a)/6=30
• Solving for a:
  • 196-a=180 (30*6)
  • a= 196-180-16
• Thus, the age of the youngest person is 16 years.

Q13. The average of three numbers is 40. The first number is the smaller. The second number is thrice the first number and and the third number is double the second number. Find the smallest number

Options:
a) 12
b) 42
c) 24
d) 20

Answer: a) 12

Solution Explained:

Here’s how to solve this:

• Let’s consider the smallest number to be x. The other numbers are:
  • Second number: 3x (Thrice the first number)
  • Third number: 6x (Double the second number, i.e. 2*3x)
• Now, as per the average formula: (x+3x+6x)/3=40 OR 10x/3 = 40
• Solving for x:
  • 10x = 120 (40*3)
  • x= 12 (120/10)
• Thus, the smallest number is 12.

Explore the topic, along with other key mathematical concepts, in detail through the Quantitative Aptitude course on Unstop.

Understanding the definition, symbol, and formula for average in math helps enhance your knowledge. To further sharpen your skills, take on more challenging problems involving averages.

Time For A Short Quiz

Frequently Asked Questions

Q1. What is the definition of average in mathematics?

The average, also known as the mean, is a value representing the central tendency of a set of numbers. It is calculated by adding all values and dividing by the total count.

Q2. How can I identify the symbol for average in mathematical equations?

The symbol for average in mathematics is often denoted by "x̄" or sometimes simply "Ave" when representing the mean of a set of numbers.

Q3. What is the formula used to calculate the average?

To find the average of a set of numbers, you sum up all the values and then divide by the total count of numbers in the dataset.

The formula for calculating the average: Average = Sum of all numbers / Total count of numbers.

Q4. Can you explain how to calculate averages step-by-step?

To calculate averages step-by-step, add up all the numbers in your dataset, then divide this sum by the total count of numbers. This will give you the average value representing the central tendency of your data.

Q5. What are some practical applications of averages in real life?

Averages are widely used in various fields such as finance, statistics, and everyday life scenarios like calculating grades, sports statistics, and determining trends in data analysis. They provide a simple yet powerful way to understand and interpret numerical information efficiently.

If you liked this, also read:

• Arithmetic Mean: Get Definition, Formula, Solved Practice Questions 
• HCF And LCM Methods: Formula, Practice Questions And Answers
• Decimal Fraction: Find Types, Operations & Practice Questions
• Decimal To Fraction Conversion Guide (+ Solved Questions)
• Angle Of Elevation: Formula With Practice Questions & Answers