Clock Questions: Basic Concepts & Aptitude Questions (MCQs) Explained

Understanding the concept and questions on clocks is essential for tackling questions in almost all competitive examinations. This topic plays a crucial role in logical reasoning, requiring both thorough analytical skills and precise calculations to arrive at accurate answers.

Basic Concepts of Clock

The clock face consists of the hour hand, the minute hand, and the second hand. These elements work together to indicate time accurately. Horology is the field that focuses on the study of clocks.

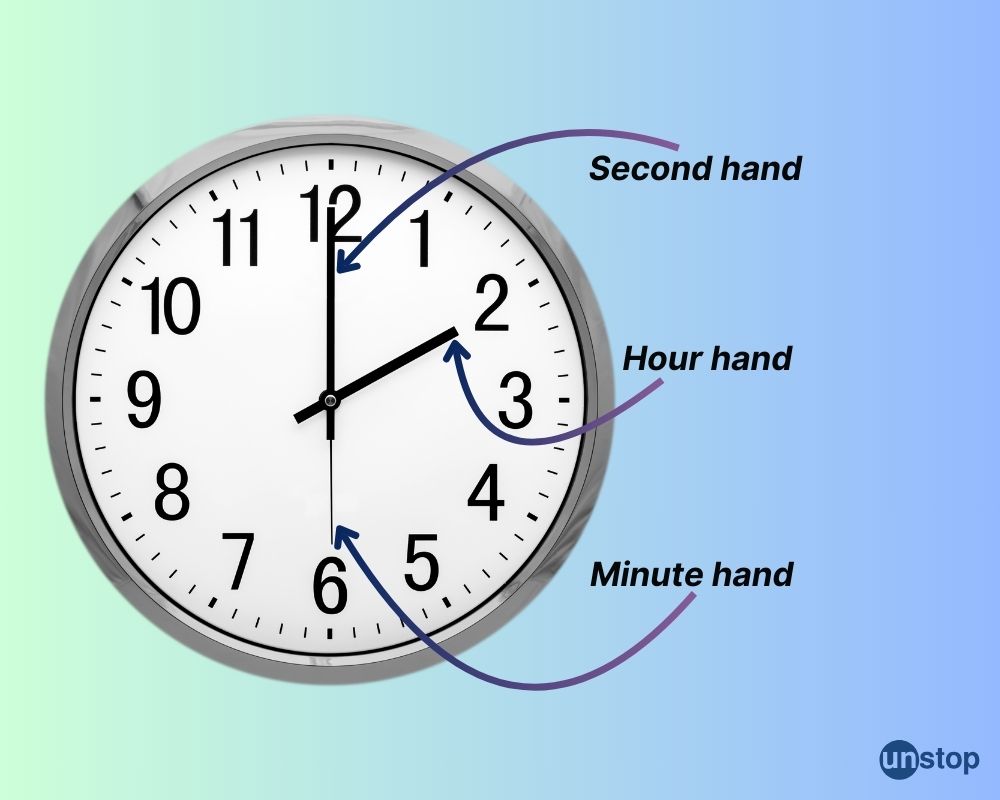
Structure of a Clock

A clock is made up of 360°, which is split into 12 equal parts. To find the angle between each part, you divide the total angle of the clock, which is 360°, by the number of sections, which is 12.

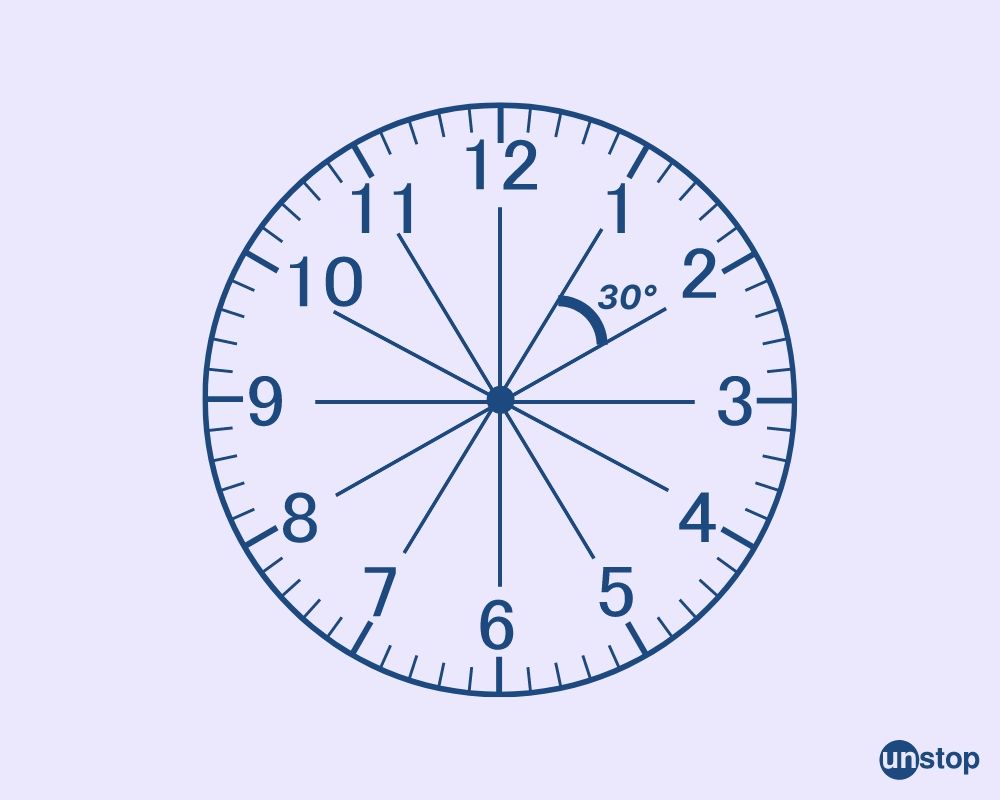
The angle between any two consecutive divisions = 360°/12= 30°

Angular Value of a Minute in a Clock

The angular value of a minute in a clock refers to the angle covered by the minute hand as it moves between minute markers. Since a clock is a circle, which is 360 degrees, we can calculate the angular movement for each minute by dividing the full 360 degrees by the 60 minutes in one hour.

Formula for Angular Value

A clock is a circle, so it has 360 degrees.
The minute hand completes one full rotation (360 degrees) in 60 minutes.
Therefore, each minute, the minute hand moves by 6 degrees.

So, the angular value of a minute on a clock is 6 degrees.

The speed of a minute hand

In one hour, the minute hand of a clock makes a complete rotation of 360°. This means it moves across all 12 sections of the clock face during that time. Since there are 60 minutes in an hour, the speed of the minute hand can be calculated as (360°)/(60 minutes).

Speed of a minute hand = 6° per minute

The speed of an hour hand

The hour hand moves at an angle of 30 degrees every hour. This means it covers the distance between each division on the clock face, which represents 5 minutes, within a span of 60 minutes. Therefore, the speed of the hour hand can be calculated as 30 degrees divided by 60 minutes, which equals 1/2 ° per minute.

Click here to enhance and upskill your quantitative and reasoning prowess consistently. It provides study resources, ensuring quality over quantity.

Angle Equilavalence in Clock

Understanding minute angle equivalence is vital for accurately calculating time-related angles. Here is the tabular representation of the angle in relation to the minute’s hand in a clock:

Minutes	Angular Value (In degree)
1 minute	6°
2 minutes	12°
3 minutes	18°
4 minutes	24°
5 minutes	30°
6 minutes	36°
7 minutes	42°
8 minutes	48°
9 minutes	54°
10 minutes	60°
15 minutes	90°
30 minutes	180°
60 minutes	360°

Tips For Solving Clock Questions

Let us study some of the tips for solving clock problems:

Angle Calculation

To quickly determine the angle between a clock's hour and minute hands, multiply the difference in hours by 30 degrees.

Pattern Recognition

Identifying recurring patterns in clock problems can streamline your problem-solving process. Look for symmetrical positions like 3:15 and 9:45, which form right angles.

Selected Clock Questions & Answers (MCQs)

Here, we provide you with a set of selected questions with detailed explanations for answers related to clock questions for placement interviews and exams:

Question 1. What is the angle between the hour and minute hands on a clock at 5:00?

a) 90 degrees

b) 120 degrees

c) 150 degrees

d) 180 degrees

Solution: At 5:00, the hour hand is exactly on the 5, and the minute hand points directly at the 12. Each hour mark represents 30 degrees on the clock face.

So, the angle covered by the hour hand from the 12 o'clock position is 5×30=150 degrees.

The minute hand points to the minute mark corresponding to the current minute. At 5:00, it's at the 12. Since it's exactly on the hour mark, the minute hand has covered 0 degrees.

To find the angle between the hands, we calculate their positions' differences. Since the hour hand is ahead of the minute hand, we subtract the minute hand's position from the hour hand's position: 150−0=150 degrees.

So, at 5:00, the angle between the minute and hour hands of the clock is 150 degrees.

Question 2. The hour and minute hands of a clock at 9:45 is

a) 22.5 degrees

b) 90 degrees

c) 112.5 degrees

d) 135 degrees

Solution: At 9:45, the hour hand is closer to the 10 than the 9 because 45 minutes have passed.

Since the hour hand moves 30 degrees in one hour and 0.5 degrees for every minute passed within an hour, we calculate its position as follows:

Hour hand angle=(9×30)+(45×0.5)=270+22.5=292.5 degrees.

The minute hand is at the 9, pointing to 45 minutes past the hour.

In one minute, the minute hand moves 6 degrees, so at 9:45, it has covered 45×6=270 degrees.

To find the angle between the hands, we calculate the absolute difference between their positions.

Since the hour hand is ahead of the minute hand, we subtract the minute hand's position from the hour hand's position: 292.5−270=22.5 degrees.

Question 3. The hour and minute hands of a clock form a right angle between 3 o'clock and 4 o'clock at

a) 3:15

b) 3:20

c) 3:30

d) 3:45

Solution: We're asked to find the time between 3 o'clock and 4 o'clock when the hour and minute hands form a right angle. This interval covers the hours between 3 and 4.

A right angle measures 90 degrees. In a clock, when the hour and minute hands form a right angle, it means the angle between them is 90 degrees.

At 3:00, the hour hand points exactly at the 3. Since the hour hand moves 30 degrees in one hour and 0.5 degrees for every minute passed within an hour, we calculate its position at each minute past 3:00 to see when it forms a right angle with the minute hand.

At 3:00, the minute hand points exactly at the 12. In one minute, the minute hand moves 6 degrees, so we calculate its position at each minute past 3:00. At each minute past 3:00, we check if the angle between the hour and minute hands is 90 degrees.

We go through the options given (3:15, 3:20, 3:30, 3:45) and determine which one satisfies the condition of the hour and minute hands forming a right angle within the 3-4 o'clock interval.

We find that at 3:45, the hour hand is at the 3, and the minute hand is pointing directly at the 9, forming a right angle with the hour hand.

Question 4. In a clock, the hour and minute hands will be aligned between 4 and 5 o'clock at

a) 4:00

b) 4:15

c) 4:30

d) 4:45

Solution: We're asked to find the time between 4 o'clock and 5 o'clock when the hour and minute hands of a clock are aligned. This interval covers the hours between 4 and 5.

When the hour and minute hands of a clock are aligned, they are pointing in the same direction, indicating the same minute mark.

At 4:00, the hour hand points exactly at the 4. Since the hour hand moves 30 degrees in one hour and 0.5 degrees for every minute passed within an hour, we calculate its position at each minute past 4:00 to see when it aligns with the minute hand.

At 4:00, the minute hand points exactly at the 12. In one minute, the minute hand moves 6 degrees, so we calculate its position at each minute past 4:00. At each minute past 4:00, we check if the hour and minute hands are pointing to the same minute mark.

When we find the time, the hour and minute hands align, that's our answer. We go through the options given (4:00, 4:15, 4:30, 4:45) and determine which one satisfies the condition of the hour and minute hands being aligned within the 4-5 o'clock interval.

We find that at 4:30, the hour hand is halfway between the 4 and the 5, and the minute hand is also pointing at the 6, indicating 30 minutes past the hour. Therefore, the hour and minute hands are aligned.

Question 5. A clock is set correctly at 5:00 a.m. on a Monday. However, the clock loses 16 minutes every 24 hours. By the time the clock shows 10:00 p.m. on Thursday, what will be the true time?

a) 10:15 pm

b) 10:59 pm

c) 10:30 pm

d) 10:00 pm

Solution: From 5:00 a.m. Monday to 10:00 p.m. Thursday is 89 hours. The clock loses 16 minutes every 24 hours, which is minutes per hour.

Over 89 hours, it loses:

So, when the clock shows 10:00 p.m., the true time is 10:59 p.m.

Question 6. The angle between the hour and minute hands at 6:45 is

a) 15 degrees

b) 22.5 degrees

c) 30 degrees

d) 67.5 degrees

Solution: At 6:45, the hour hand is exactly on the 7, but it has moved slightly past 6 due to the 45 minutes passed. The hour hand moves 30 degrees in one hour and 0.5 degrees for every minute passed within an hour.

So, at 6:45, the angle covered by the hour hand is:

Hour hand angle=(6×30)+(45×0.5)=180+22.5=202.5 degrees

At 6:45, the minute hand points directly to the 9, representing 45 minutes past the hour. In one minute, the minute hand moves 6 degrees, so at 6:45, the angle covered by the minute hand is 45×6=270 degrees.

To find the angle between the hands, we calculate the absolute difference between their positions.

Since the hour hand is behind the minute hand, we subtract the hour hand's position from the minute hand's position: 270−202.5=67.5 degrees. So, at 6:45, the angle between the hour and minute hands of the clock is 67.5 degrees.

Question 7. The angle between the hour and minute hands at 10:30 is

a) 45 degrees

b) 75 degrees

c) 105 degrees

d) 135 degrees

Solution: At 10:30, the hour hand is exactly on the 10, but it has moved slightly past 10 due to the 30 minutes passed. The hour hand moves 30 degrees in one hour and 0.5 degrees for every minute passed within an hour.

So, at 10:30, the angle covered by the hour hand is:

Hour hand angle=(10×30)+(30×0.5)=300+15=315 degrees

At 10:30, the minute hand points directly to the 6, representing 30 minutes past the hour. In one minute, the minute hand moves 6 degrees, so at 10:30, the angle covered by the minute hand is 30×6=180 degrees.

To find the angle between the hands, we calculate the absolute difference between their positions. Since the hour hand is behind the minute hand, we subtract the hour hand's position from the minute hand's position: 180−315=135 degrees. So, at 10:30, the angle between the hour and minute hands of the clock is 135 degrees.

Question 8. At what time between 1 and 2 o'clock will a clock's hour and minute hands be closest together?

a) 1:05

b) 1:15

c) 1:30

d) 1:45

Solution: We're asked to find the time between 1 o'clock and 2 o'clock when the hour and minute hands of a clock are closest together. This interval covers the hours between 1 and 2. The rate at which the angle between the hour and minute hands changes is not constant but varies as time progresses.

At 1:00, the hour hand points directly to the 1, and the minute hand points to the 12, overlapping with the hour hand. The angle between the hour and minute hands is 0 degrees at this time.

To find when the hour and minute hands are closest together, we need to determine when the difference between their positions is smallest.

We go through the options given (1:05, 1:15, 1:30, 1:45) and determine which one satisfies the condition of the hour and minute hands being closest together within the 1-2 o'clock interval.

We find that at 1:15, the hour hand has moved slightly past the 1, and the minute hand has moved away from the 12. This time is when the hour and minute hands are closest together.

Question 9. The hour and minute hands form a right angle between 3 o'clock and 4 o'clock at

a) 3:05

b) 3:15

c) 3:30

d) 3:45

Solution: We're asked to find the time between 3 o'clock and 4 o'clock when the hour and minute hands of a clock form a right angle. This interval covers the hours between 3 and 4.

A right angle between the hour and minute hands of a clock occurs twice in every hour: once when the minute hand is ahead of the hour hand and once when it's behind.

At 3:00, the hour hand points exactly at the 3.

Since the hour hand moves 30 degrees in one hour, we calculate its position at each minute past 3:00 to see when it forms a right angle with the minute hand. At 3:00, the minute hand points exactly at the 12.

In one minute, the minute hand moves 6 degrees, so we calculate its position at each minute past 3:00. At each minute past 3:00, we check if the angle between the hour and minute hands is 90 degrees.

We go through the options given (3:05, 3:15, 3:30, 3:45) and determine which satisfies the condition of the hour and minute hands forming a right angle within the 3-4 o'clock interval.

Therefore, the hour and minute hands form a right angle at 3:30.

Question 10: Between 9 o'clock and 10 o'clock, the hour and minute hands of a clock are farthest apart at

a) 9:15

b) 9:30

c) 9:45

d) 9:50

Solution: We're asked to find the time between 9 o'clock and 10 o'clock when the hour and minute hands of a clock are farthest apart. This interval covers the hours between 9 and 10. The hour and minute hands of a clock are farthest apart when they are at opposite ends of the clock face.

At 9:00, the hour hand points exactly at the 9.

Since the hour hand moves 30 degrees in one hour, we calculate its position at each minute past 9:00 to determine when it's farthest from the minute hand. At 9:00, the minute hand points exactly at the 12.

In one minute, the minute hand moves 6 degrees, so we calculate its position at each minute past 9:00. We go through the options given (9:15, 9:30, 9:45, 9:50) and determine which one satisfies the condition of the hour and minute hands being farthest apart within the 9-10 o'clock interval.

We find that at 9:50, the hour hand is close to the 10, and the minute hand is close to the 12. This time represents when the hour and minute hands are farthest apart.

Conclusion

In conclusion, one can effectively understand the practical applications of clock knowledge by exploring the speed comparison of clock hands, learning tips for efficient problem-solving, and practising selected sample questions.

Take the time to engage with the material covered, practice regularly, and explore additional resources to deepen your understanding of clock questions.

Frequently Asked Questions (FAQs)

1. How can one understand the structure of a clock?

Clock structure involves the face, hands, and markings. The face has numbers or Roman numerals representing hours. The hour hand is shorter and moves slowly, while the minute hand is longer and moves faster. The second hand, if present, ticks continuously. Understanding these components aids in reading time accurately.

2. What are the essential clock formulas and concepts to grasp?

Key formulas include calculating angles between clock hands and determining time elapsed based on their positions. Concepts like the relative speed of hands and angular displacement are crucial for solving clock problems efficiently.

3. Can you explain minute angle equivalence in clocks?

Minute angle equivalence means every minute mark on a clock corresponds to 6 degrees. This principle simplifies calculating angles between the hour and minute hands at any given time by converting minutes into degrees.

4. How does one compare the speed of clock hands?

The minute hand is 12 times faster than the hour hand since it covers 360 degrees (a full circle) in an hour compared to the hour hand's 30 degrees per hour. Understanding this speed difference is vital for accurate time interpretation.

5. What are some key shortcuts for solving clock problems effectively?

Shortcuts like visualizing fractions of an hour or using multiples of 5 minutes can expedite calculations involving clock angles or time intervals. These techniques streamline problem-solving and enhance accuracy when dealing with various clock-related scenarios.

Kaihrii Thomas

Associate Content Writer

Instinctively, I fall for nature, music, humour, reading, writing, listening, travelling, observing, learning, unlearning, friendship, exercise, etc., all these from the cradle to the grave- that's ME! It's my irrefutable belief in the uniqueness of all. I'll vehemently defend your right to be your best while I expect the same from you!